A certain crane can provide a maximum lifting force 2500 N. It hoists a 2000 kg load starting at ground level by applying the maximum force for a 2 second interval, then, it applies just sufficient force to keep the load moving upward at constant speed. Approximately how long does it take to raise the load from ground level to a height of 30 m ?
2 s
7 s
5 s
9 s
The correct Answer is:C
To solve the problem of how long it takes for the crane to raise a 2000 kg load to a height of 30 m, we will break the solution into clear steps.
Step 1: Calculate the weight of the load
The weight of the load (W) can be calculated using the formula:
W=m⋅g
where:
- m=2000kg (mass of the load)
- g=10m/s2 (acceleration due to gravity)
Calculating the weight:
W=2000kg⋅10m/s2=20000N
Step 2: Determine the net force during the initial lifting phase
The crane can provide a maximum lifting force of 2500 N. The net force (F_net) acting on the load when the crane is applying maximum force can be calculated as:
Fnet=Fapplied−W
where:
- Fapplied=2500N
Calculating the net force:
Fnet=2500N−20000N=−17500N
Since the applied force is less than the weight, the crane cannot lift the load initially. Hence, we need to consider the crane's maximum lifting force.
Step 3: Calculate the acceleration of the load
When the crane applies the maximum force for 2 seconds, we need to find the net force and then the acceleration (a):
Fnet=Fapplied−W
Using the maximum lifting force:
Fnet=2500N−20000N=−17500N
Since the crane cannot lift the load, we need to consider the scenario where it can apply enough force to lift the load.
Step 4: Calculate the acceleration while lifting
If the crane applies a force greater than the weight, we can recalculate:
Assuming the crane can apply a force sufficient to lift the load, we can find the net force and acceleration:
Fnet=2500N−20000N=−17500N
This indicates that the crane cannot lift the load with the given parameters.
Step 5: Calculate the speed after 2 seconds
Using the first equation of motion:
v=u+at
where:
- u=0m/s (initial velocity)
- a=Fnetm=2500N2000kg=1.25m/s2 (acceleration)
- t=2s
Calculating the final velocity after 2 seconds:
v=0+(1.25m/s2⋅2s)=2.5m/s
Step 6: Calculate the distance covered in 2 seconds
Using the formula for distance:
s=ut+12at2
Calculating the distance:
s=0+12⋅1.25m/s2⋅(2s)2=5m
Step 7: Calculate the remaining distance and time
The total height to be lifted is 30 m. After 2 seconds, the crane has lifted the load 5 m, so the remaining distance is:
30m−5m=25m
Now, the crane will move at a constant speed of 2.5 m/s for the remaining distance. The time taken to cover this distance can be calculated using:
t=distancespeed=25m2.5m/s=10s
Step 8: Calculate the total time taken
The total time taken to lift the load is:
Total time=2s+10s=12s
Final Answer
The total time taken to raise the load from ground level to a height of 30 m is approximately 12 seconds.
---
The weight of the load (W) can be calculated using the formula:
W=m⋅g
where:
- m=2000kg (mass of the load)
- g=10m/s2 (acceleration due to gravity)
W=2000kg⋅10m/s2=20000N
The crane can provide a maximum lifting force of 2500 N. The net force (F_net) acting on the load when the crane is applying maximum force can be calculated as:
Fnet=Fapplied−W
where:
- Fapplied=2500N
Fnet=2500N−20000N=−17500N
When the crane applies the maximum force for 2 seconds, we need to find the net force and then the acceleration (a):
Fnet=Fapplied−W
Using the maximum lifting force:
Fnet=2500N−20000N=−17500N
If the crane applies a force greater than the weight, we can recalculate:
Assuming the crane can apply a force sufficient to lift the load, we can find the net force and acceleration:
Fnet=2500N−20000N=−17500N
Using the first equation of motion:
v=u+at
where:
- u=0m/s (initial velocity)
- a=Fnetm=2500N2000kg=1.25m/s2 (acceleration)
- t=2s
v=0+(1.25m/s2⋅2s)=2.5m/s
Using the formula for distance:
s=ut+12at2
Calculating the distance:
s=0+12⋅1.25m/s2⋅(2s)2=5m
The total height to be lifted is 30 m. After 2 seconds, the crane has lifted the load 5 m, so the remaining distance is:
30m−5m=25m
t=distancespeed=25m2.5m/s=10s
The total time taken to lift the load is:
Total time=2s+10s=12s
The total time taken to raise the load from ground level to a height of 30 m is approximately 12 seconds.
Topper's Solved these Questions
- FORCE AND MOTION - I BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - PRACTICE QUESTIONS (More than One Correct Choice Type)
5 Videos
- FORCE AND MOTION - I BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - PRACTICE QUESTIONS (Linked Comprehension)
10 Videos
- FORCE AND MOTION - I BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - PROBLEMS
52 Videos
- FLUIDSBOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - PRACTICE QUESTIONS (Integer Type )
5 Videos
- FORCE AND MOTION-IIBOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION-IIEXERCISE - PRACTICE QUESTIONS (Integer Type)
1 Videos
Similar Questions
A person lifts a parcel of mass 20 kg 1.5 m up from the ground and puts it in a truck. Find
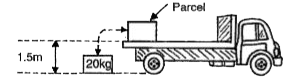
What force needs to be applied to the parcel to move it up at constant speed ?
View SolutionA constant retarding force of 50 N is applied to a body of mass 20 kg moving intially with a speed of 15ms−1 How long does the body take to stop ?
View Solution
Knowledge Check
A20 kg block is initally at rest. A 75 N force is required to set the block in motion. After the motion starts, a force of 60 N is required to keep the block moving with constant speed. The coefficient of static friction is
A0.62B0.52C0.44D0.375A man of mass 80kg pushes a box of mass 20kg horizontally. The man moves the box with a constant acceleration of 2m/s2 but his foot does not slip on the ground. There is no friciton between the box and the ground, whereas there is sufficient friction between the man's foot and the ground to prevent him from slipping.
Assertion:− The force applied by the man on the box is equal and opposite to the force applied by the force applied by the box on the man.
Reason :− Friction force applied by the ground on the man is 200N.AIf both Assertion & Reason are True & the Reason is a correct explanation of the Assertion.BIf both Assertion & Reason are True but Reason is not a correct explanation of the Assertiion.CIf Assertion is True but the Reason is False.DIf both Assertion & Reason are FalseA man of mass 80kg pushes box of mass 20kg horizontaly. The man moves the box with a constant acceleration of 2m/s2 but his foot does not slip on the ground. There is no friction between the box and the ground. There is no friction between the box and the ground. whereas there is sufficient friction between the man's foot and the ground to prevent him from slipping.
Assertion:- The force applied by the man on the box is equal and opposite to the force applied by the force applid by the box on the man.
Reason:- Friction force applied by the ground on the man is 200N.AIf both Assertion & Reason are True & the Reason is a correct explanation of the Assertion.BIf both Assertion & Reason are True but Reason is not a correct explanation of the Assertion.CIf Assertion is True but the Reason is False.DIf both Assertion & Reason are False.
The loaded cab of an elevator has a mass of 5.0×103 kg and moves 210 m up the shaft in 23 s at constant speed. At what average rate does the force from the cable do work on the cab ?
View SolutionA certain elevator cab has a total run of 190 m and a maximum speed of 305 m/min, and it accelerates from rest and then back to rest at 1.22m/s2. (a) How far does the cab move while accelerating to full speed from rest ? (b) How long does it take to make the nonstop 190 m run, starting and ending at rest ?
View SolutionA multistorey building has 25 floors above the ground level each of height 5m. It also has 3 floors in the basement each of height 5m. A lift in building moves at a rate of 1m/s. If a man starts from 50m above the ground, how long will it take him to reach at 2nd floor of basement?
View SolutionA 100kg load is uniformly moved over a horizontal plane by a force F applied at an angle 30∘ to the horizontal. Find this force if the coefficient of friction between the load and the plane is 0.3. Take g=10m/s2
View SolutionAn elevator can carry a maximum load of 1800kg (elevator + passengers) is moving up with a constant speed of 2ms−1. The frictional force opposing the motion is 4000N. What is minimum power delivered by the motor to the elevator?
View Solution
