Figure 26-21 shows wire section 1 of diameter D1=4.00R and wire section 2 of diameter D2=1.75R, connected by a tapered section. The wire is Copper and carries a current. Assume that the current is uniformly distributed across any cross-sectional area through the wire's width. The electric potential change V along the length L= 2.00 m shown in section 2 is 10.0μV. The number of charge carners per unit volume is 8.49×1028m−3. What is the drift speed of the conduction electrons in section 1? 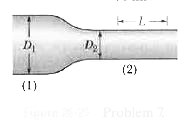Figure 26-21 shows wire section 1 of diameter D1= 4.00R and wire section 2 of diameter D2= 1.75R, connected by a tapered section. The wire is Copper and carries a current. Assume that the current is uniformly distributed across any cross-sectional area through the wire's width. The electric potential change V along the length L= 2.00 m shown in section 2 is 10.0 muV. The number of charge carners per unit volume is 8.49 xx 10^(28) m^(-3). What is the drift speed of the conduction electrons in section 1?

Text Solution
Verified by Experts

The correct Answer is:6.22×10−9m/s

Updated on:21/07/2023
Doubtnut Promotions Banner
|
Class 12PHYSICSCURRENT ELECTRICITY

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • A uniform current I is flowing in a long wire of radius R. If the current is uniformly distributed across the cross-sectional area of the wire, then

    Amagnetic field increases linearly from centre to surface
    Bmagnetic field decays inversely with distance r from the centre of wire for r>R
    Cmagnetic field at the centre of wire is zero
    DNone of the above

  • A long cylindrical wire carries a steady current which is uniformly distributed across its cross section. Which of the following is true?

    AMagnetic field is zero at the centre.
    BElectric field is zero at the centre.
    CElectric field just outside the cylinder is zero.
    DMagnetic field just outside the cylinder is zero.

  • A long straight wire of radius a carries a steady current i. The current is uniformly distributed across its cross section. The ratio of the magnetis field at (a)/(2)and(2a) is

    A(1/2)
    B(1/4)
    C4
    D1