A wire 2.30 m long carries a current of 13.0 A and makes an angle of with a uniform magnetic field of magnitude B = 1.50 T. Calculate the magnetic force on the wire.
To calculate the magnetic force on a wire carrying a current in a magnetic field, we can use the formula:
F=I⋅B⋅L⋅sin(θ)
Where:
- F is the magnetic force,
- I is the current in the wire (in Amperes),
- B is the magnetic field strength (in Teslas),
- L is the length of the wire (in meters),
- θ is the angle between the wire and the magnetic field (in degrees).
1. Identify the given values:
- Length of the wire, L=2.30m
- Current, I=13.0A
- Magnetic field strength, B=1.50T
- Angle, θ=35.0∘
2. Convert the angle to radians (if necessary):
- In this case, we can use the sine function directly with degrees, so we do not need to convert to radians.
3. Calculate sin(35∘):
- Using a calculator, find sin(35∘).
- sin(35∘)≈0.5736
4. Substitute the values into the formula:
F=I⋅B⋅L⋅sin(θ)
F=13.0A⋅1.50T⋅2.30m⋅sin(35∘)
5. Calculate the force:
F=13.0⋅1.50⋅2.30⋅0.5736
F≈13.0⋅1.50⋅2.30⋅0.5736≈25.72N
Final Answer:
The magnetic force on the wire is approximately 25.72N.
---
- F is the magnetic force,
- I is the current in the wire (in Amperes),
- B is the magnetic field strength (in Teslas),
- L is the length of the wire (in meters),
- θ is the angle between the wire and the magnetic field (in degrees).
- Length of the wire, L=2.30m
- Current, I=13.0A
- Magnetic field strength, B=1.50T
- Angle, θ=35.0∘
- In this case, we can use the sine function directly with degrees, so we do not need to convert to radians.
- Using a calculator, find sin(35∘).
- sin(35∘)≈0.5736
F=I⋅B⋅L⋅sin(θ)
F=13.0A⋅1.50T⋅2.30m⋅sin(35∘)
F=13.0⋅1.50⋅2.30⋅0.5736
F≈13.0⋅1.50⋅2.30⋅0.5736≈25.72N
The magnetic force on the wire is approximately 25.72N.
Topper's Solved these Questions
- MAGNETIC FORCEBOOK - RESNICK AND HALLIDAYCHAPTER - MAGNETIC FORCEEXERCISE - Practice Questions (Single correct Choice Type )
24 Videos
- MAGNETIC FORCEBOOK - RESNICK AND HALLIDAYCHAPTER - MAGNETIC FORCEEXERCISE - Practice Questions (More than one correct choice Type)
11 Videos
- MAGNETIC FORCEBOOK - RESNICK AND HALLIDAYCHAPTER - MAGNETIC FORCEEXERCISE - Check point
6 Videos
- MAGNETIC FIELDS DUE TO CURRENTSBOOK - RESNICK AND HALLIDAYCHAPTER - MAGNETIC FIELDS DUE TO CURRENTSEXERCISE - Practice Question (Integer Type)
4 Videos
- MOTION ALONG A STRAIGHT LINEBOOK - RESNICK AND HALLIDAYCHAPTER - MOTION ALONG A STRAIGHT LINEEXERCISE - PRACTICE QUESTIONS ( Integer Type )
3 Videos
Similar Questions
A straight wire of1.4 m long carries a current of 7 A at right angles to a uniform magnetic field of 0.01 T. Calculate the mechanical force acting on the wire due to the magnetic field.
View SolutionA straight wire 50cm long carries a current of 5A. It is placed at right angle to a uniform magnetic field of 1T. Find the mechanical force on the wire and the power required to move it at 10ms−1 in a plane at right angles to the magnetic field.
View Solution
Knowledge Check
A straight wire of length 2 m carries a current of 10 A. If this wire is placed in a uniform magnetic field of 0.15 T making an angle of 45∘ with the magnetic field, the applied force on the wire will be
A1.5NB3NC3√2ND3√2NThe magnetic force per unit length on a wire carrying a current of 10 A and making an angle of 45∘ with the direction of a uniform magnetic field of 0.20 T is
A2√2Nm−1B2√2Nm−1C√22Nm−1D4√2Nm−1The magnetic force per unit length on a wire carrying a current of 10 A and making an angle of 45∘ with the direction of a uniform magnetic field of 0.20 T is
A2√2Nm−1B2√2Nm−1C√22Nm−1D4√2Nm−1
A horizontal rod 0.2m long is mounted on a balnce and carries a current. At the location of the rod a uniform horizontal magnetic field has magnitude 0.067T and direction perpendicular to the rod. The magnetic force on the rod is measured by the balance and is found to be 0.13N. What is the current?
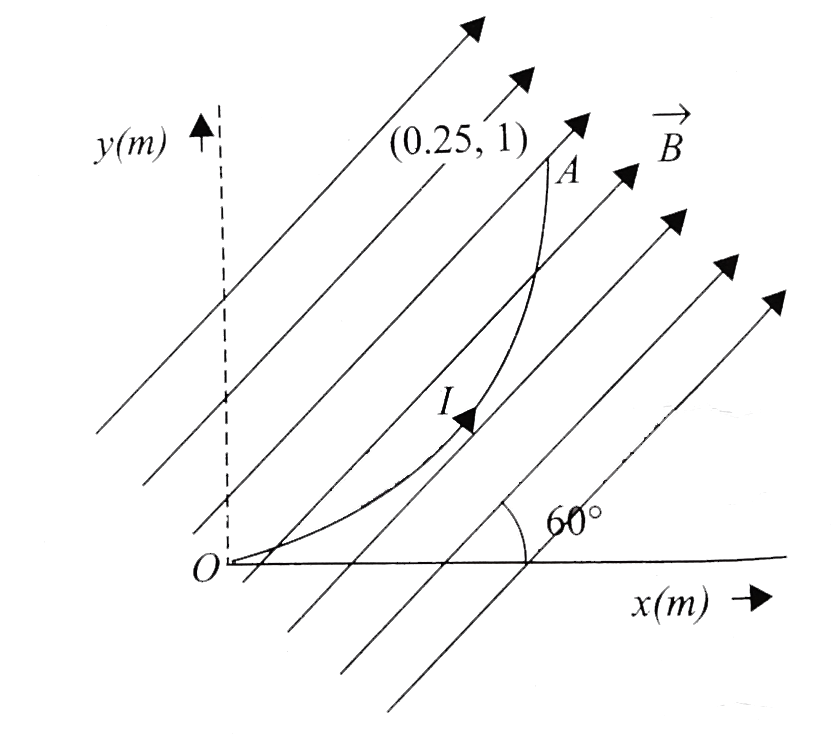
View SolutionA parabolic section of wire OA is located in the x-y plane and carries current I=12A. A uniform magnetic field B=4.0T making an angle 60∘ with x axis exists in x-y plane. Calculate the magnetic force on the wire OA. Coordinates of A are (0.25 m, 1 m)
View SolutionA straight wire of length 2 m carries a current of 10 A. If this wire is placed in uniform magnetic field of 0.15 T making an angle of 45∘ with the magnetic field, the applied force on the wire will he
View SolutionA straight wire of length 2m carries a current of 10A. If this wire is placed in a uniform magnetic field of 0.15T making an angle of 45∘ with the magnetic field, the applied force on the wire will be
View SolutionA wire bent in the form a right angled triangle PQR, carries a current 1A. It is placed in a region of a uniform magnetic field B=0.2T. If PR=1m, the net force on the wire is

View Solution
