Consider the neutron capture reaction
109Ag+n→110A>o110Ag+γ (40-34)
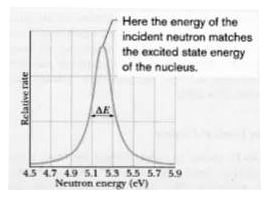
in which a compound nucleus (110Ag) is formed. Figure 40-18 shows the relative rate at which such events take place, plotted against the energy of the incoming neutron. Find the mean lifetime of this compound nucleus by using the uncertainty principle in the form
ΔE.Δt≈ˆh. (40-35)
here ΔE is a mesure of the uncertainty with which the energy of a state can be defined. The quantity Δt is a measure of the time available of measure this energy. In fact here Δt is just tavg, the average life of the compound nucleus befoe it decays to its ground state.
Reasoning: We see that the relative reaction rate peaks sharply at a neutron energy of about 5.2 eV.This suggests that we are dealing with a single excited energy level of the compund nucleus 110Ag. When the availabel energy(of the incoming neutron) just matches the energy of this level above the neutron) just matches the energy of this level above the 110Ag ground state, we have resonance and the reaction of Eq. 40 -34 really goes.
However, the resonance peak is not infinitely sharp but has an approximate half peak is not infinitely shart but has an approximate half width (ΔE in the figue) of about 0.20 eV. We can account for this resonance peak width by saying that the excited level is not sharply defined in energy but has an energy uncertainty ΔE of about 0.20 eV.
Calculation : Subsitiuting that uncertainty of 0.20 2V into Eq. given us
Δ=tavg≈hΔE≈(4.14×10−15eV.s)/2π0.20eV
≈3×10−15s.
This is several hundraed times greater than the time a 5.2 eV neutron takes to cross the diameter of a 109Ag nucleus . Therefore , the neutron is spending this time of 3×10−15 s as part of the nucleus.
Reasoning: We see that the relative reaction rate peaks sharply at a neutron energy of about 5.2 eV.This suggests that we are dealing with a single excited energy level of the compund nucleus 110Ag. When the available energy(of the incoming neutron) just matches the energy of this level above the neutron) just matches the energy of this level above the 110Ag ground state, we have resonance and the reaction of Eq. 40 -34 really goes.
However, the resonance peak is not infinitely sharp but has an approximate half peak is not infinitely shart but has an approximate half width (ΔE in the figue) of about 0.20 eV. We can account for this resonance peak width by saying that the excited level is not sharply defined in energy but has an energy uncertainty ΔE of about 0.20 eV.
Calculations: Substituting that uncertainty of 0.20 eV into Eq. 40-35 gives us
Δt=tavg≈ˆhDe<AE≈(4.14×1015eV.s)/2π0.20eV
≈3×10−15s
this is several hundred times greater than the time a 5.2 eV neutron takes to cross the diameter of a 109Ag nucleus. Therefore, the neutron is spending this time of 3×10−15 as a part of the nucleus.
Topper's Solved these Questions
- THE NUCLEUSBOOK - RESNICK AND HALLIDAYCHAPTER - THE NUCLEUSEXERCISE - CHECKPOINT
5 Videos
- THE NUCLEUSBOOK - RESNICK AND HALLIDAYCHAPTER - THE NUCLEUSEXERCISE - PROBLEMS
66 Videos
- THE KINETIC THEORY OF GASESBOOK - RESNICK AND HALLIDAYCHAPTER - THE KINETIC THEORY OF GASESEXERCISE - PRACTICE QUESTIONS
72 Videos
- UNITS AND MEASUREMENT BOOK - RESNICK AND HALLIDAYCHAPTER - UNITS AND MEASUREMENT EXERCISE - PRACTICE QUESTIONS (MATRIX-MATCH)
11 Videos
Similar Questions
A measurement of the energy ΔE of an intermediate nucleus must be made within the mean lifetime Δtof the nucleus and necessarily carries an uncertainty ΔEaccording to the uncertainty principle
ΔE.Δ=h
(a) What is the uncertainty ΔE in the energy for an intermediate nucleus if the nucleus has a mean lifetime of 10−22s? (b) Is the nucleus a compound nucleus?
View SolutionWhy electron cannot exist inside the nucleus according to Heisenberg's uncertainty principle?
View SolutionWhy electron cannot exist inside the nucleus according to Heisenberg's uncertainty principle?
View SolutionWhy electron cannot exist inside the nucleus according to Heisenberg's uncertainty principle ?
View SolutionWhy electron cannot exist inside the nucleus according to Heisenberg's uncertainty principle?
View SolutionHow many neutrons are present in .4018Ca ?
View SolutionOn the basis of Heisenbergs uncertainty principle show that the electron cannot exist within the nucleus.
View SolutionOn the basis of heisenhergs uncertainty principle show that the electron correct exist within the nucleus
View SolutionOn the basis of heisenhergs uncertainty principle show that the electron can not exist within the nucleus
View SolutionSum of the ages of a father and the son is 40 years. If father’s age is three times that of his son, then find their ages.
View Solution
