From two identical wires, a rod XY of length l is hung. A wooden block of mass m is hung at point O of the rod at a distance a from wire A. Find the value of a in the case when a tuning fork excites the fundamental note in wire A and third harmonic in wire B.
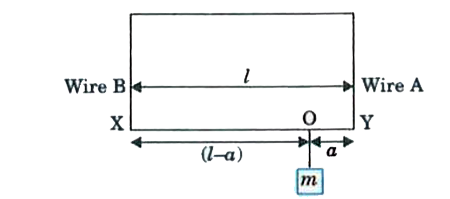
m' = mass per unit length of each wire.
T1,T2 are tensions produced in wires A and B.
According to given condition
For wire 1
v = frequency =12l√T1m ...(i)
For wire 2
v=32l√T2m ...(ii)
From (i) and (ii)
12l√T1m=32l√T2m
⇒ T1T2=9
Applying rotational equilibrium of rod about O. We have,
T1(a)=T2(l−a)
T1T2=l−aa
But T1T2=9
∴ l−aa=9
l - a = 9a
⇒ 10a=l
a=l10
Topper's Solved these Questions
- WAVESBOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - REVISION EXERCISES
107 Videos
- WAVESBOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - MULTIPLE CHOICE QUESTIONS
88 Videos
- WAVESBOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - NCERT EXEMPLAR PROBLEMS
31 Videos
- UNITS AND MEASUREMENTBOOK - MODERN PUBLICATIONCHAPTER - UNITS AND MEASUREMENTEXERCISE - CHAPTER PRACTICE TEST
15 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Chapter Practice Test
16 Videos
Similar Questions
A uniform rod of length l and mass m is hung from, strings of equal length from a ceiling as shown in figure. Determine the tensions in the strings?

View SolutionA rod of length l is rotating with an angular speed ω about one of its ends which is at a distance a from an infinitely lonf wire carrying current i. Find the emf induced in the rod at the instant shown in .

View Solution
Knowledge Check
A rod PQ of length 'L' is hung from two identical wires A and B. A block of mass 'm' is hung at point R of the rod as shown in figure. The value of 'x' so that the fundamental mode in wire A is in resonance with first overtone of B is
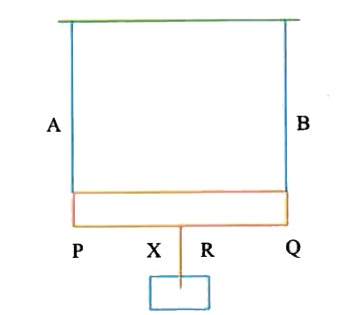 A4L5BL4CL5D2L3
A4L5BL4CL5D2L3A massless rod BD is suspended by two identical massless strings AB and CD of equal lengths. A block of mass m is suspended at point P such that BP is equal to x, If the fundamental frequency of the left wire is twice the fundamental frequency of right wire, then the value of x is :-
_E01_188_Q01.png) Al5Bl4C4l5D3l4
Al5Bl4C4l5D3l4The potential gradient along the length of a uniform wire is 5 V/m. There are two points on the same wire at a distance of 20 cm and 40 cm from initial end of the wire. The potential difference between these points is
A1VB2VC3VD4V
A rod of length l is rotating with an angular speed ω about its one end which is at a distance a from an infinitely long wire carrying current i.Find the emf induced in the rod at the instant shown in the figure.

View SolutionA rod of length l is rotating with an angular speed ω about one of its ends which is at a distance a from an infinitely long wire carrying current i. Find the emf induecd in the rod at the instanr shown in .
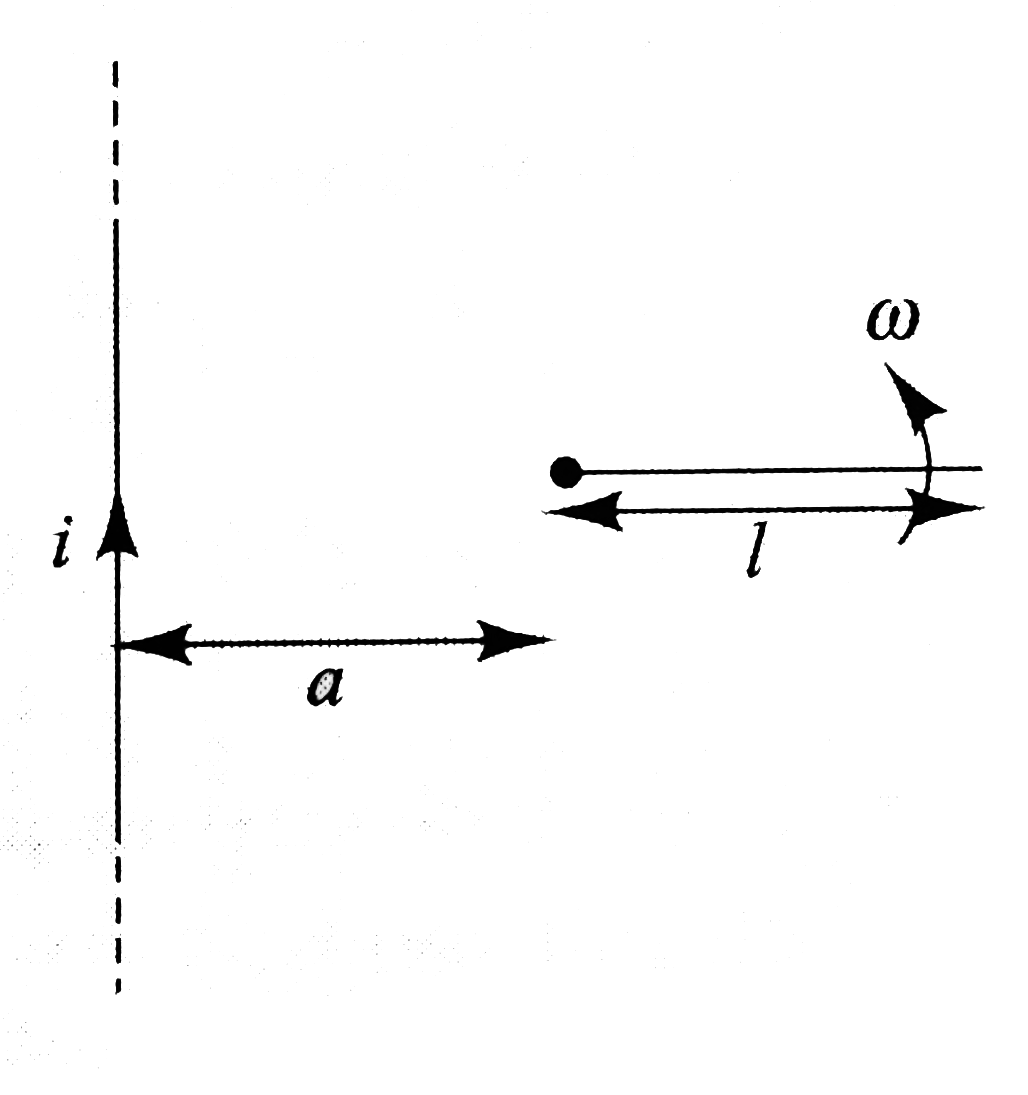
View SolutionA uniform horizontal rod of length 40cm and mass 1.2kg is supported by two identical wires as shown in figure. Where should a mass of 4.8kg be placed on the ord form left end (in cm) so that the same tuning fork may excite the wire on left into its fundament vibrations and that on right into its first overtone? Take g=10m/s2.

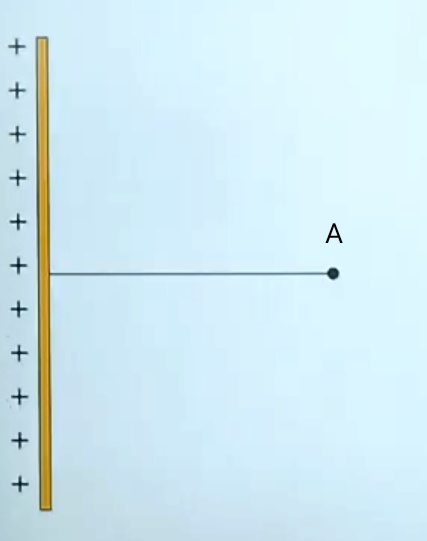
View SolutionA wire of length L carries charge Q uniformly distributed along its length. Find the electric field at a distance √3L2 from the wire at point A as shown.
View Solutionthe fundamental frequency of a sonometer wire of length is f0.A bridge is now introduced at a distance of Δlfrom the centre of the wire (Δl<<l). The number of beats heard if their fundamental mode are
View Solution
