Where should a stretched wire, held between two fixed supports, be plucked and touched to excite its second harmonic ?
To determine where to pluck and touch a stretched wire held between two fixed supports to excite its second harmonic, we can follow these steps:
Step 1: Understand the concept of harmonics
The second harmonic of a stretched wire corresponds to a standing wave pattern with two loops (antinodes) and one node in the center. The general formula for the harmonics of a string fixed at both ends is given by:
- The first harmonic (fundamental frequency) has 1 loop (1 antinode).
- The second harmonic has 2 loops (2 antinodes).
Step 2: Visualize the wire and its nodes/antinodes
In the case of a stretched wire, the fixed ends are nodes (points of no displacement), while the points of maximum displacement are the antinodes. For the second harmonic:
- There will be a node at the center of the wire.
- There will be two antinodes, one at each quarter length of the wire.
Step 3: Determine the positions of the antinodes
If the total length of the wire is L:
- The first antinode (maximum displacement) for the second harmonic will be at L/4 from one end.
- The second antinode will be at 3L/4 from the same end.
Step 4: Conclusion
To excite the second harmonic, the wire should be plucked and touched at either:
- L/4 (one-quarter of the length from one end) or
- 3L/4 (three-quarters of the length from the same end).
Final Answer
You should pluck and touch the wire at positions L/4 and 3L/4 to excite its second harmonic.
---
The second harmonic of a stretched wire corresponds to a standing wave pattern with two loops (antinodes) and one node in the center. The general formula for the harmonics of a string fixed at both ends is given by:
- The first harmonic (fundamental frequency) has 1 loop (1 antinode).
- The second harmonic has 2 loops (2 antinodes).
In the case of a stretched wire, the fixed ends are nodes (points of no displacement), while the points of maximum displacement are the antinodes. For the second harmonic:
- There will be a node at the center of the wire.
- There will be two antinodes, one at each quarter length of the wire.
If the total length of the wire is L:
- The first antinode (maximum displacement) for the second harmonic will be at L/4 from one end.
- The second antinode will be at 3L/4 from the same end.
To excite the second harmonic, the wire should be plucked and touched at either:
- L/4 (one-quarter of the length from one end) or
- 3L/4 (three-quarters of the length from the same end).
You should pluck and touch the wire at positions L/4 and 3L/4 to excite its second harmonic.
Topper's Solved these Questions
- WAVESBOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - MULTIPLE CHOICE QUESTIONS
88 Videos
- WAVESBOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - ASSERTION REASON TYPE QUESTIONS
11 Videos
- WAVESBOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - HIGH ORDER THINKING SKILLS & ADVANCED LEVEL
15 Videos
- UNITS AND MEASUREMENTBOOK - MODERN PUBLICATIONCHAPTER - UNITS AND MEASUREMENTEXERCISE - CHAPTER PRACTICE TEST
15 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Chapter Practice Test
16 Videos
Similar Questions
What points of the stretched string between the fixed points must be plucked and touched to excite its second harmonic ?
View SolutionA steel wire of length 50√3cm is connected to an aluminium wire of length 60cm and stretched between two fixed supports. The tension produced is 104N, if the cross section area of each wire is 1mm2. If a transverse wave is set up in the wire, find the lowest frequency for which standing waves with node at the joint are produced . (density of aluminimum =2.6gm/cm3 and density of steel =7.8gm/cm3).
View Solution
Knowledge Check
Show that all harmonics are present on a stretched string between two rigid supports.
Aa nodeBan antinodeCeither a node or an antinodeDneither a node nor an antinode.A second harmonic has to be generated in a string of length l stretched between two rigid supports. The point where the string has to be plucked and touched are
Al4,l2Bl4,3l4Cl2,l2Dl2,3l4between two rigid support. The point where the string has to be pluked and touched are
Apluked at l4 and touch at l2BPluked at l4 and touch at 3l4CPluked at l2 and touched at l4DPluked at l2 and touched at 3l4
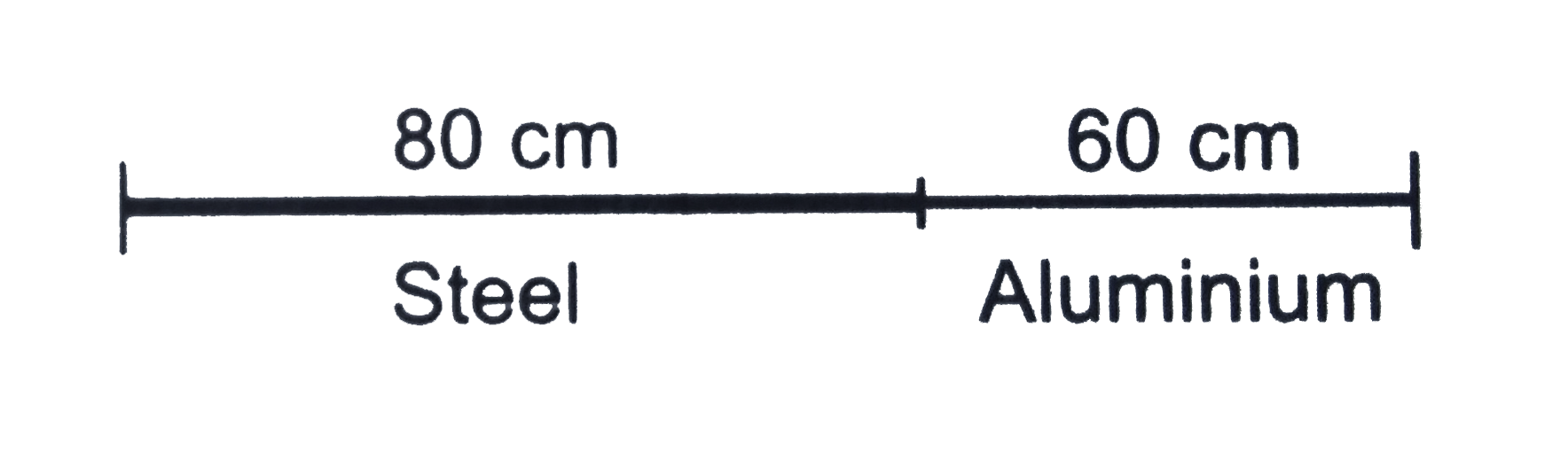
Figure shows an aluminium wire of length 60 cm joined to a steel wire of length 80 cm and stretched between two fixed supports. The tension produced is 40 N. The cross-sectional area of the steel wire is .1.0mm2 and that of the aluminium wire is 3.0 mm 2. What could be the minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node ? The density of aluminium is 2.6gcm−3and that of steel is 7.8gcm−3
View SolutionA string of length 75 cm is stretched between two fixed supports. It is found that standing waves may be excited at frequencies of 315 Hz and 420Hz, but at no frequencies in between. What is the lowest frequency at which a standing wave may be excited in this string ?
View SolutionA decrease in temperature of 40∘C produces a 0.1 % strain in a wire stretched between two fixed supports. If the area of cross section of the wire is πmm2 and Young's modulus of its material is 160 GPa, the thermal stress in the wire is
View SolutionA second harmonic has to be generated in a string of length l stretched between two rigid supports. The point where the string has to be plucked and touched are
View SolutionOne metre long sonometer wire is stretched with a force of 4kg wt, another wire of same material and diamter is arranged along a side. The second wire is stretched with a force of 16kg wt. if the length of the second wire is in its second harmonic is the same as fifth harmonic of the first wire, then the length of the second wire will be
View Solution
