Cacluate the amount of CO2 dissolved at 4 atm in 1 dm3 of water at 298 K . The Henry's law constant for CO2 at 298K is 1.67 k bar .
The correct Answer is:5.85 g
To solve the problem of calculating the amount of CO2 dissolved at 4 atm in 1 dm³ of water at 298 K, we will use Henry's Law and follow these steps:
Step 1: Understand Henry's Law
Henry's Law states that the amount of gas dissolved in a liquid at a given temperature is directly proportional to the partial pressure of the gas above the liquid. The formula is given by:
P=kH⋅X
Where:
- P = partial pressure of the gas (in atm)
- kH = Henry's law constant (in atm or bar)
- X = mole fraction of the gas in the liquid
Step 2: Convert Henry's Law Constant
Given kH=1.67kbar, we need to convert it to atm for consistency.
1kbar=1000bar=1000×0.98692atm≈986.92atm
Thus,
kH=1.67kbar=1.67×1000bar=1670bar≈1670×0.98692atm≈1648.57atm
Step 3: Calculate the Mole Fraction
Given the partial pressure P=4atm:
Using Henry's Law:
4atm=1648.57atm⋅X
Rearranging to find X:
X=4atm1648.57atm≈0.00243
Step 4: Calculate Moles of Water
The volume of water is 1 dm³ (or 1 L). The density of water is approximately 1 g/cm³, so:
Mass of water=1000g
The molar mass of water H2O is approximately 18 g/mol. Therefore, the number of moles of water nH2O is:
nH2O=1000g18g/mol≈55.56mol
Step 5: Calculate Moles of CO2
Using the mole fraction formula:
X=nCO2nCO2+nH2O
Substituting the values we have:
0.00243=nCO2nCO2+55.56
Let nCO2=x:
0.00243=xx+55.56
Cross-multiplying gives:
0.00243(x+55.56)=x
Expanding and rearranging:
0.00243x+0.135=x
0.135=x−0.00243x
0.135=0.99757x
x≈0.1350.99757≈0.135
Step 6: Calculate Mass of CO2 Dissolved
The molar mass of CO2 is approximately 44 g/mol. Therefore, the mass of CO2 dissolved is:
Mass=nCO2×Molar mass of CO2=0.135mol×44g/mol≈5.94g
Final Answer
The amount of CO2 dissolved at 4 atm in 1 dm³ of water at 298 K is approximately 5.94 g.
---
Henry's Law states that the amount of gas dissolved in a liquid at a given temperature is directly proportional to the partial pressure of the gas above the liquid. The formula is given by:
- P = partial pressure of the gas (in atm)
- kH = Henry's law constant (in atm or bar)
- X = mole fraction of the gas in the liquid
Given kH=1.67kbar, we need to convert it to atm for consistency.
Given the partial pressure P=4atm:
The volume of water is 1 dm³ (or 1 L). The density of water is approximately 1 g/cm³, so:
Using the mole fraction formula:
The molar mass of CO2 is approximately 44 g/mol. Therefore, the mass of CO2 dissolved is:
The amount of CO2 dissolved at 4 atm in 1 dm³ of water at 298 K is approximately 5.94 g.
Topper's Solved these Questions
- SOLUTIONSBOOK - MODERN PUBLICATIONCHAPTER - SOLUTIONSEXERCISE - ADVANCED LEVEL (PROBLEMS)
21 Videos
- SOLUTIONSBOOK - MODERN PUBLICATIONCHAPTER - SOLUTIONSEXERCISE - CONCEPTUAL QUESTIONS
44 Videos
- SOLUTIONSBOOK - MODERN PUBLICATIONCHAPTER - SOLUTIONSEXERCISE - UNIT PRACTICE TEST
14 Videos
- SOLID STATEBOOK - MODERN PUBLICATIONCHAPTER - SOLID STATEEXERCISE - UNIT PRACTICE TEST
13 Videos
- SURFACE CHEMISTRYBOOK - MODERN PUBLICATIONCHAPTER - SURFACE CHEMISTRYEXERCISE - COMPETITION FILE (OBJECTIVE TYPE QUESTIONS) (MATCHING LIST TYPE QUESTIONS)
2 Videos
Similar Questions
CO2 gas is bubbled through water during a soft drink manufacturing process at 298 K. If CO2 exerts a partial pressure of 0.835 bar then x m mol of CO2 would dissolve in 0.9 L of water. The value of x is ________ . (Nearest integer)
(Henry's law constant for CO2 at 298 K is 1.67×103 bar )
View SolutionCalculate the concentration of CO2 in a soft drink that is bottled with a partial pressure of CO2 of 4 atm over the liquid at 25∘C. The Henry's law constant for CO2 in water at 25∘C is 3.1×10−2mol//litre-atm.
View Solution
Knowledge Check
If CO2 gas having a partial pressure of 1.67 bar is bubbled through 1 L water at 298 K, the amount of CO2 dissolved in water in g L−1 is approximately :
(Herny's law constant of CO2is 1.67 k bar at 298 K)A24.42B12.21C2.44D1.22N2 gas is bubbled through water at 293K and the partial pressure of N2 is 0.987 bar .If the henry's law constant for N2 at 293K is 76.84 kbar, the number of millimoles of N2 gas that will dissolve in 1L of water at 293K is
A1.29B0.716C2.29D7.16For the solution of the gases W,X,Y and z in water at 298 K , the Henrys law constants (KH) are 0.5, 2, 35 and 40 kbar, respectively. The correct plot for the given data is :
A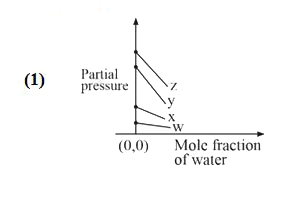 B
B C
C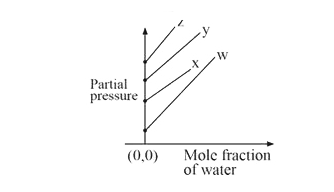 D
D
At what partial pressure , oxygen will have a solubility of 0.06gL−1 in water at 298 K ? Henry's law constant (KH) of O2 in water at 303 K is 46.82 k bar .(Assume the density of the solution to be the same as that of water).
View SolutionIf N2 gas is bubbled through water at 293K, how many millimoles of N2 gas would dissolve in1L of water. Assume thatN2 exerts a partial pressure of 0.987 bar. Given that Henry law constant for N2 at 293K is 76.48 kbar.
View Solution(a) Explain why on addition of 1 mole glucose to 1 litre water the boling point of water increases .5
(b) Henry's law constant for CO2 in water is 1.67108Paat298K.Calculate the number of moles of CO2 in 500 ml of soda water when packed under 2.53×105 Pa at same temperature.
View SolutionThe air is a mixture of a number of gases. The major components are oxygen and nitrogen with approximate proportion of 20% is to 79% by volume at 298 K. The water is in equilibrium with air at a pressure of 10 atm. At 298 K, if the Henry's law constants for oxygen and nitrogen at 298 K are 3.30 x 10' mm and 6.51×107 mm, respectively, calculate the composition of these gases in water.
View SolutionLet gas (A) present in air is dissolved in 20 moles of water at 298K and 20 atm pressure. The mole fraction of gas (A) in air is 0.2 and the Henry's law constant for solubility of gas (A) in water at 298K is 1×105atm.The number of mole of gas (A) dissolved in water will be
View Solution
