Express 3825 as product of its prime factors using factor tree.
To express 3825 as a product of its prime factors using a factor tree, we will follow these steps:
Step 1: Start with the number 3825
We will create a factor tree starting with the number 3825.
Step 2: Find the smallest prime factor
We check if 3825 is divisible by the smallest prime number, which is 2. Since 3825 is odd, it is not divisible by 2. Next, we check 3:
- Sum of digits of 3825 = 3 + 8 + 2 + 5 = 18 (which is divisible by 3).
Thus, 3825 is divisible by 3.
Step 3: Divide by 3
Now, we divide 3825 by 3:
3825÷3=1275
So, we write:
3825=3×1275
Step 4: Factor 1275
Next, we factor 1275. Again, we check for divisibility by 3:
- Sum of digits of 1275 = 1 + 2 + 7 + 5 = 15 (which is divisible by 3).
So, we divide 1275 by 3:
1275÷3=425
Thus, we write:
1275=3×425
So, now we have:
3825=32×425
Step 5: Factor 425
Next, we factor 425. We check for divisibility by 5 (the next smallest prime):
- The last digit of 425 is 5, so it is divisible by 5.
We divide 425 by 5:
425÷5=85
So, we write:
425=5×85
Now, we have:
3825=32×5×85
Step 6: Factor 85
Next, we factor 85. We again check for divisibility by 5:
- The last digit of 85 is 5, so it is divisible by 5.
We divide 85 by 5:
85÷5=17
So, we write:
85=5×17
Now, we have:
3825=32×52×17
Final Result
Thus, the prime factorization of 3825 is:
3825=32×52×17
---
We will create a factor tree starting with the number 3825.
We check if 3825 is divisible by the smallest prime number, which is 2. Since 3825 is odd, it is not divisible by 2. Next, we check 3:
- Sum of digits of 3825 = 3 + 8 + 2 + 5 = 18 (which is divisible by 3).
Thus, 3825 is divisible by 3.
Now, we divide 3825 by 3:
3825÷3=1275
So, we write:
3825=3×1275
Next, we factor 1275. Again, we check for divisibility by 3:
- Sum of digits of 1275 = 1 + 2 + 7 + 5 = 15 (which is divisible by 3).
So, we divide 1275 by 3:
1275÷3=425
Thus, we write:
1275=3×425
So, now we have:
3825=32×425
Next, we factor 425. We check for divisibility by 5 (the next smallest prime):
- The last digit of 425 is 5, so it is divisible by 5.
We divide 425 by 5:
425÷5=85
So, we write:
425=5×85
Now, we have:
3825=32×5×85
Next, we factor 85. We again check for divisibility by 5:
- The last digit of 85 is 5, so it is divisible by 5.
We divide 85 by 5:
85÷5=17
So, we write:
85=5×17
Now, we have:
3825=32×52×17
Thus, the prime factorization of 3825 is:
3825=32×52×17
Topper's Solved these Questions
- REAL NUMBERSBOOK - VK GLOBAL PUBLICATIONCHAPTER - REAL NUMBERSEXERCISE - Proficiency Exercise (Long Answer Questions)
9 Videos
- REAL NUMBERSBOOK - VK GLOBAL PUBLICATIONCHAPTER - REAL NUMBERSEXERCISE - Self -Assessment Test
11 Videos
- REAL NUMBERSBOOK - VK GLOBAL PUBLICATIONCHAPTER - REAL NUMBERSEXERCISE - Proficiency Exercise (Short Answer Questions-I)
8 Videos
- QUADRATIC EQUATIONSBOOK - VK GLOBAL PUBLICATIONCHAPTER - QUADRATIC EQUATIONSEXERCISE - SELF-ASSESSMENT TEST
11 Videos
- TRIANGLESBOOK - VK GLOBAL PUBLICATIONCHAPTER - TRIANGLESEXERCISE - SELF ASSESSMENT TEST
10 Videos
Similar Questions
Express 32760 as product of its prime factors using factor tree.
View SolutionExpress 360 as product of its prime factors.
View SolutionExpress 429 as a product of its prime factors.
View SolutionExpress 429 as a product of its prime factors.
View SolutionExpress 429 as a product of its prime factors.
View SolutionExpress 7429 as a product of its prime factors.
View SolutionWrite 98 as product of its prime factors.
View SolutionExpress 75 as the product of prime factors.
View SolutionExpress 120 as the product of prime factors.
View Solution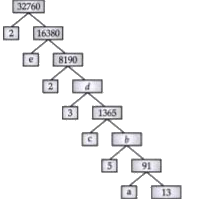
Here , number 32760 is represented as the Product of its Prime factors using the factor tree .
Find the value of a
View Solution
