Question

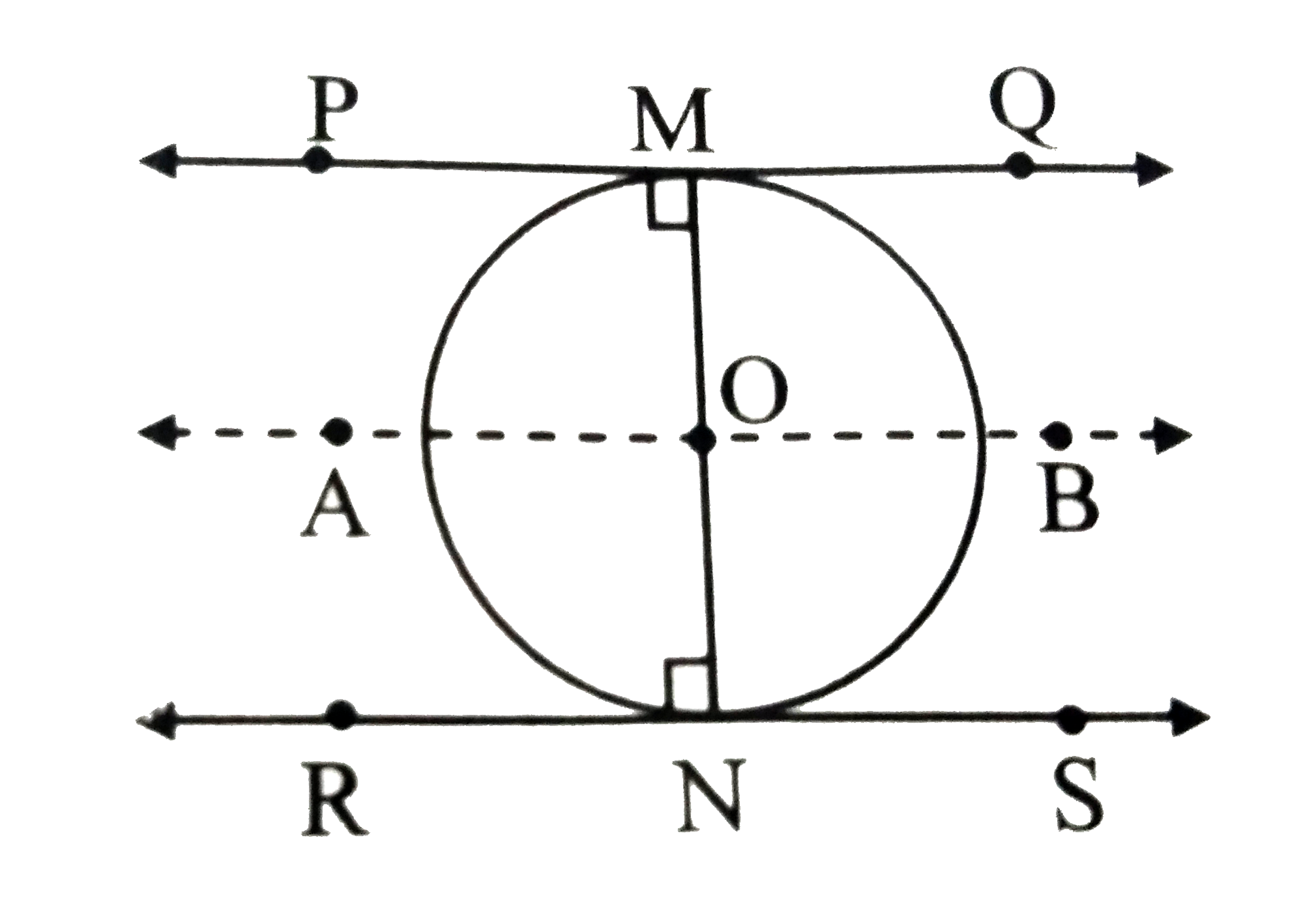
Find the distance between two parallel tangents of a circle of radius 3 cm.

Answer

6 cm.

Explanation

To find the distance between two parallel tangents of a circle with a radius of 3 cm, we can follow these steps:

Step 1: Understand the Geometry of the Circle
A circle has a center, and tangents are lines that touch the circle at exactly one point. When we have two parallel tangents, they are equidistant from the center of the circle.

Step 2: Identify the Radius
The radius of the circle is given as 3 cm. This radius is the distance from the center of the circle to any point on the circumference.

Step 3: Use the Property of Tangents
The distance between two parallel tangents can be calculated using the formula:
\( \text{Distance between two parallel tangents} = 2 \times \text{Radius} \)
This is because each tangent is at a distance equal to the radius from the center of the circle.

Step 4: Substitute the Radius into the Formula
Substituting the radius into the formula:
\( \text{Distance} = 2 \times 3 \, \text{cm} \)
\( \text{Distance} = 6 \, \text{cm} \)

Conclusion
The distance between the two parallel tangents of the circle is 6 cm.

---