A circus artist is climbing from the ground along a rope stretched from the top of a vertical pole and tied at the ground. The height of the pole is 10 m and angle made by the top with ground level is 60∘ .
(i) Calculate the distance covered by the artist in climbing to the top of the pole.
(ii) Which mathematical concept is used in this problem ?
(iii) What is its value ?
The correct Answer is:(i) Hence, distance covered by artist is 20√33 m .
(ii) Trigonometric rations of an acute of right angled triangle.
(iii) Single mindedness help us to gain success in life.
To solve the problem step by step, we will use trigonometric concepts.
Step 1: Understand the Problem
We have a vertical pole of height 10 m and a rope tied from the top of the pole to the ground, making an angle of 60 degrees with the ground. We need to find the distance covered by the artist in climbing to the top of the pole.
Step 2: Identify the Right Triangle
In this scenario, we can visualize a right triangle where:
- The height of the pole (AB) is the opposite side (10 m).
- The distance from the base of the pole to the point where the rope touches the ground (let's call it point C) is the adjacent side.
- The rope itself (AC) is the hypotenuse.
Step 3: Use the Sine Function
We can use the sine function to relate the angle to the sides of the triangle. The sine of an angle in a right triangle is defined as:
sin(θ)=OppositeHypotenuse
Here, θ=60∘, the opposite side is the height of the pole (10 m), and the hypotenuse is the length of the rope (AC).
Step 4: Set Up the Equation
Using the sine function:
sin(60∘)=10AC
We know that sin(60∘)=√32. Therefore, we can substitute this value into the equation:
√32=10AC
Step 5: Solve for AC
To find AC, we can rearrange the equation:
AC=10×2√3=20√3 meters
Step 6: Rationalize the Denominator
To express AC in a more standard form, we can rationalize the denominator:
AC=20√3×√3√3=20√33 meters
Final Answer for Part (i)
The distance covered by the artist in climbing to the top of the pole is:
AC=20√33 meters
Step 7: Identify the Mathematical Concept (Part ii)
The mathematical concept used in this problem is Trigonometry, specifically the use of the sine function in a right triangle.
Step 8: State the Value (Part iii)
The value represented in this problem can be seen as single-mindedness, which emphasizes the importance of having a clear goal and determination to achieve it.
We have a vertical pole of height 10 m and a rope tied from the top of the pole to the ground, making an angle of 60 degrees with the ground. We need to find the distance covered by the artist in climbing to the top of the pole.
In this scenario, we can visualize a right triangle where:
- The height of the pole (AB) is the opposite side (10 m).
- The distance from the base of the pole to the point where the rope touches the ground (let's call it point C) is the adjacent side.
- The rope itself (AC) is the hypotenuse.
We can use the sine function to relate the angle to the sides of the triangle. The sine of an angle in a right triangle is defined as:
sin(θ)=OppositeHypotenuse
Here, θ=60∘, the opposite side is the height of the pole (10 m), and the hypotenuse is the length of the rope (AC).
Using the sine function:
sin(60∘)=10AC
We know that sin(60∘)=√32. Therefore, we can substitute this value into the equation:
√32=10AC
To find AC, we can rearrange the equation:
AC=10×2√3=20√3 meters
To express AC in a more standard form, we can rationalize the denominator:
AC=20√3×√3√3=20√33 meters
The distance covered by the artist in climbing to the top of the pole is:
AC=20√33 meters
The mathematical concept used in this problem is Trigonometry, specifically the use of the sine function in a right triangle.
The value represented in this problem can be seen as single-mindedness, which emphasizes the importance of having a clear goal and determination to achieve it.
Topper's Solved these Questions
- VALUE-BASED QUESTIONSBOOK - VK GLOBAL PUBLICATIONCHAPTER - VALUE-BASED QUESTIONSEXERCISE - Unit-VI : (Mensuration)
5 Videos
- VALUE-BASED QUESTIONSBOOK - VK GLOBAL PUBLICATIONCHAPTER - VALUE-BASED QUESTIONSEXERCISE - Unit-VII : (Statistics & Probability)
8 Videos
- VALUE-BASED QUESTIONSBOOK - VK GLOBAL PUBLICATIONCHAPTER - VALUE-BASED QUESTIONSEXERCISE - Unit-IV : (Geometry)
3 Videos
- TRIANGLESBOOK - VK GLOBAL PUBLICATIONCHAPTER - TRIANGLESEXERCISE - SELF ASSESSMENT TEST
10 Videos
Similar Questions
A circus artist is climbing from the ground along a rope stretched from the top of a vertical pole and tied at the ground. The height of the pole is 12 m and the angle made by the rope with ground level is 30o . Calculate the distance covered by the artist in climbing to the top of the pole.
View SolutionA circus artist is climbing a 20 m long rope ; which is tightly stretched and tied from the top of a vertical pole to the ground . Find the height of the pole if the angle made by the rope with the ground level is 30∘
View Solution
Knowledge Check
At a distance 2h m from the foot of a tower of height h m,the top of the tower and a pole at the top of the tower subtend equal angles height of the pole should be
A(5h)/3mB(4h)/3mC(7h)/5mD(3h)/2mAt a distance 2h m from the foot of a tower of height h m,the top of the tower and a pole at the top of the tower subtend equal angles height of the pole should be
A(5h)/3mB(4h)/3mC(7h)/5mD(3h)/2mA vertical pole stands on the level ground. From a point on the ground, 25m away from the foot of the pole , the angle of elevation of its top is found to be 60∘. Find the height of the pole.
A25√3B50√3C55√3D65√3
A circus artists is climbing a 20m long rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole if the angle made by the rope with the ground level is 300.
View SolutionA circus artist is climbing a 20 m long rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole, if the angle made by the rope with the ground level is 30∘.
View SolutionA circus artist is climbing a 20 m long rope, which is tightly stretched and tied from the top of a vertical pole to the ground . Find the height of the pole, if the angle and by the rope with ground leave is 30∘
View SolutionAnand is watching a circus artist climbing a 20m long rope which is tightly stretched and tied from the top of vertical pole to the ground. Find the height of the pole if the angle made by the rope with the ground level is 30∘.
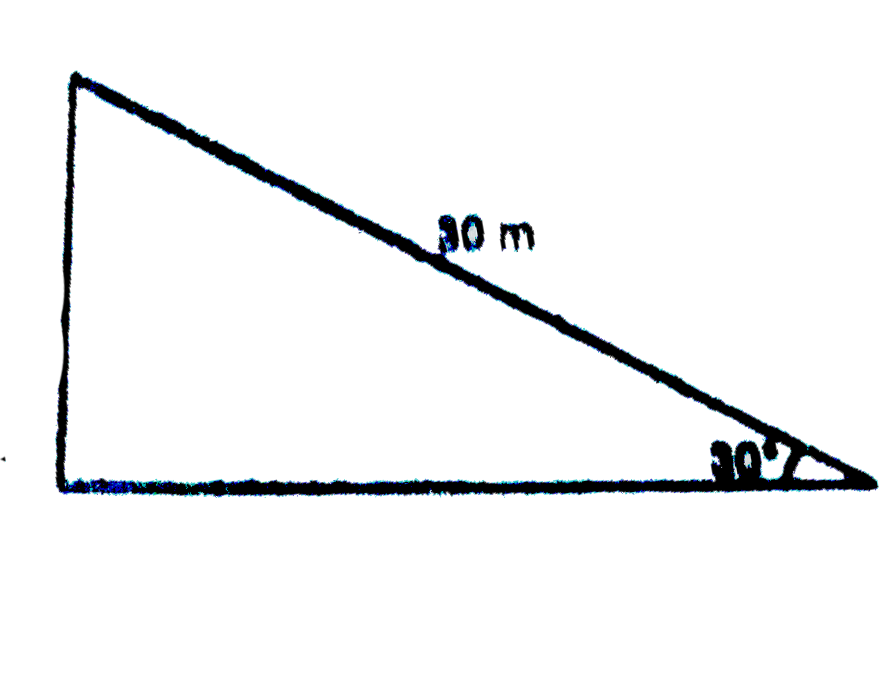
View SolutionA circus artist is climbing a 30 m rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole to the ground. Find the height of the angle made by the rope with the ground level is 30∘), as shown in the figure.
View Solution
