Keeping the source frequency equal to the resonating frequency of the series LCR circuit, if the three elements, L, C and R are arranged in parallel, show that the total current in the parallel LCR circuit is minimum at this frequency. Obtain the current rms value in each branch of the circuit for the elements and source specified in Exercise for this frequency.
Effective impedance of the parallel LCR circuit is given by
which is minimum at
Therefore, |Z| is maximum at , and the total current amplitude is minimum.
In R branch,
In L branch,
In C branch,
Note: total current , since the currents in L and C branch are out of phase and add to zero at every instant of the cycle.
Topper's Solved these Questions
Similar Questions
Keeping the source of frequency equal to the resonating frequency of the series LCR circuit, if the three elements L, C and R in are arranged in parallel , show that the total current in the parallel LCR circuit is a minimum at this frequency. Obtain the r.m.s. value of current in each brach of the circuit for the elements and source specified in for this frequency.
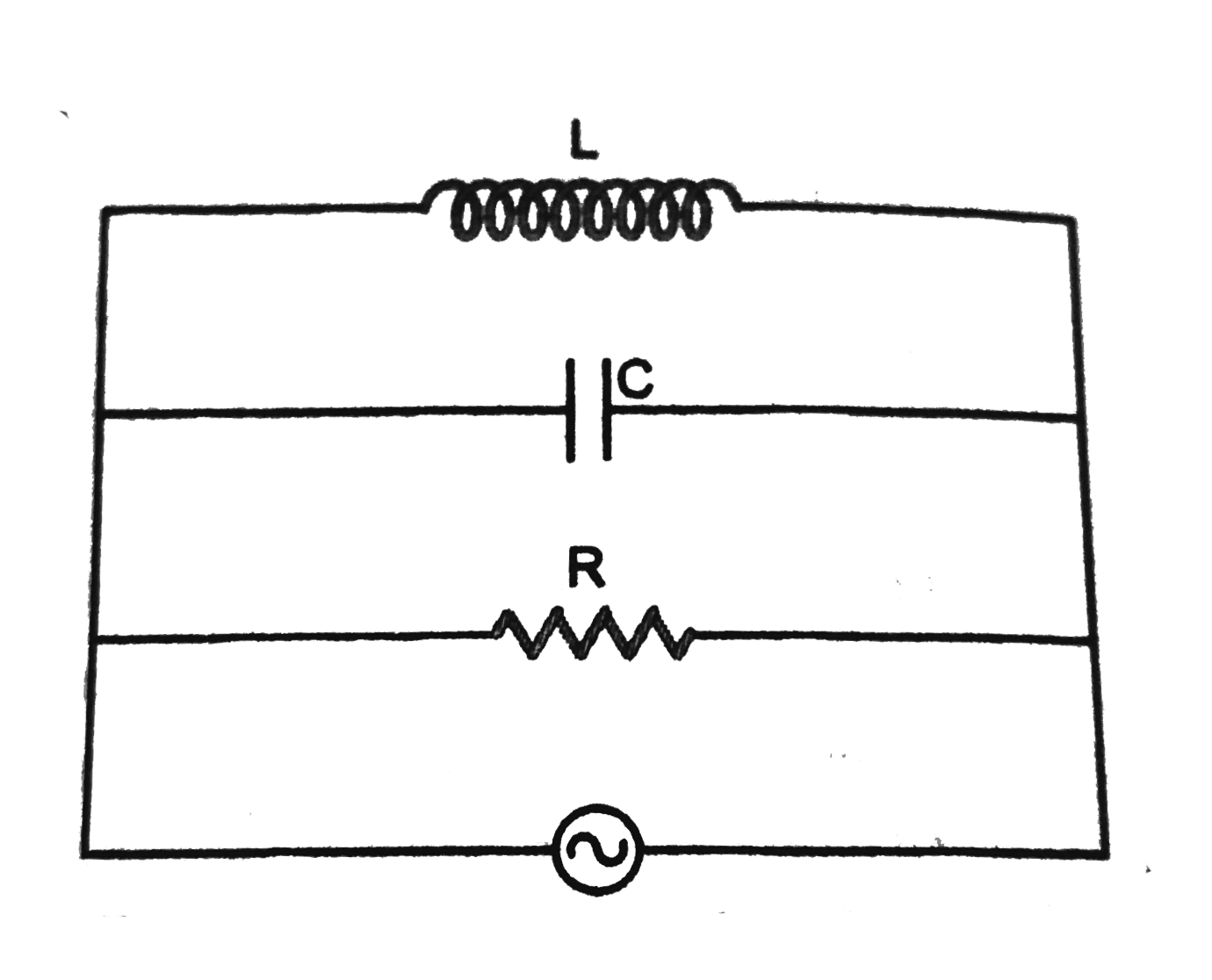
View SolutionKeeping the source of frequency equal to the resonating frequency of the series LCR circuit, if the three elements L, C and R in are arranged in parallel , show that the total current in the parallel LCR circuit is a minimum at this frequency. Obtain the r.m.s. value of current in each brach of the circuit for the elements and source specified in for this frequency.

View Solution
Knowledge Check
At resonance frequency the impedance in series LCR circuit is
AmaximumBminimumCzeroDinfinityAt resonance frequency the impedance in series LCR circuit is
AmaximumBminimumCzeroDinfinityTo reduce the resonant frequency in an LCR series circuit with a generator
Athe generator frequency should be reducedBanother capacitor should be added in parallel to the firstCthe iron core of the inductor should be removedDdielectric in the capacitor should be removed.
To reduce the resonant frequency in an LCR series circuit with a generator
View SolutionTo reduce the resonant frequency in an LCR series circuit with a generator
View SolutionObtain an expression for the resonant frequency (f0) of a series LCR circuit.
View SolutionTo reduce the resonant frequency in an LCR series circuit with a generator
View SolutionIn a series LCR circuit, at resonant frequency.
View Solution
