If ¯¯¯E and ¯¯¯F are the complementary events of E and F respectively and if 0<P(F)<1, then
A
P(E/F)+P( −E/F)=1
B
P(E/F)+P(E/ −F)=1
C
P( −E/F)+P(E/ −F)=1
D
P(E/ −F)+P( −E/ −F)=1
Video Solution
Text Solution
The correct Answer is:A, B
(a)P(E/F)+P(¯¯¯E/F)=P(E∩F)P(F)+P(¯¯¯E∩F)P(F)
=P(E∩F)+P(¯¯¯E∩F)P(F)
=P(F)P(F)=1
Therefore, option (a) is correct.
(b)P(E/F)+P(E/¯¯¯F)=P(E∩F)P(F)+P(E∩¯¯¯F)P(¯¯¯F)
=P(E∩F)P(F)+(PE∩¯¯¯F1−P(F)≠1
Therefore, option (b) is not correct.
(c)P(¯¯¯E/F)+P(E/¯¯¯F)=P(¯¯¯E∩F)P(F)+P(E∩¯¯¯F)P(¯¯¯F)
=P(¯¯¯E∩F)P(F)+P(E∩¯¯¯F)1−P(F)≠1
Therefore, option (c) is not correct.
(d)P(E/¯¯¯F)+P(¯¯¯E/¯¯¯F)=P(E∩¯¯¯F)P(¯¯¯F)+P(¯¯¯E∩¯¯¯F)P(¯¯¯F)
=P(¯¯¯F)P(¯¯¯F)=1
Therefore, option (d) is correct.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - PROBABILITYEXERCISE - TOPIC 3 INDEPENDENT AND CONDITIONAL PROBABILITY OBJECTIVE QUESTION II (FILL IN THE BLANKS )5videos
PROBABILITY
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - PROBABILITYEXERCISE - TOPIC 3 INDEPENDENT AND CONDITIONAL PROBABILITY OBJECTIVE QUESTION II (ANALYTICAL AND DESCRIPTIVE QUESTIONS )11videos
PROBABILITY
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - PROBABILITYEXERCISE - TOPIC 3 INDEPENDENT AND CONDITIONAL PROBABILITY OBJECTIVE QUESTION I (ONLY ONE CORRECT OPTION)20videos
PROBABILITY
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Dearrangement and Number of Divisors (Fill in the Blank )1videos
PERMUTATIONS AND COMBINATIONS
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - All Questions1videos
RELATIONS AND FUNCTIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
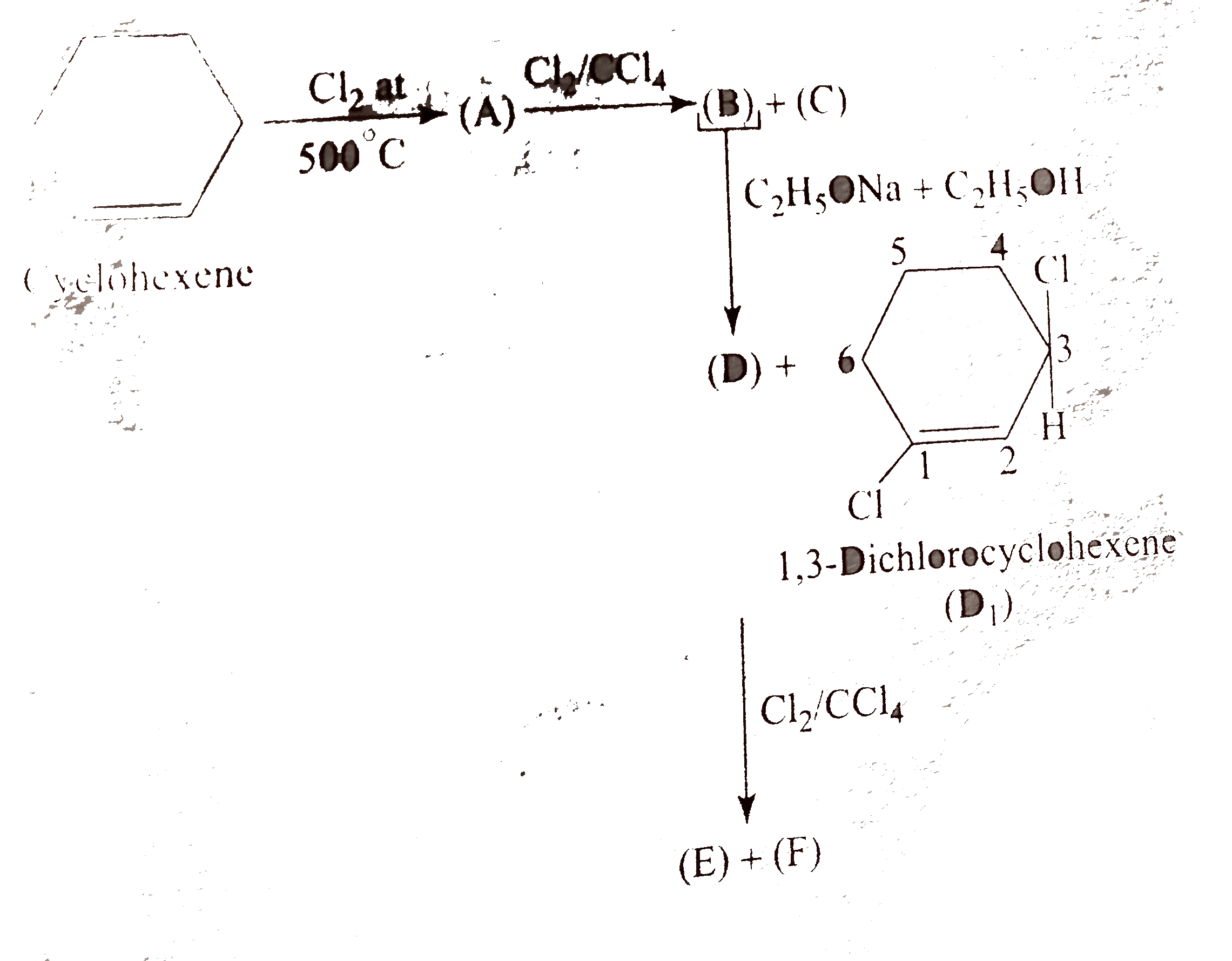
(E) and (F), respectively, are :A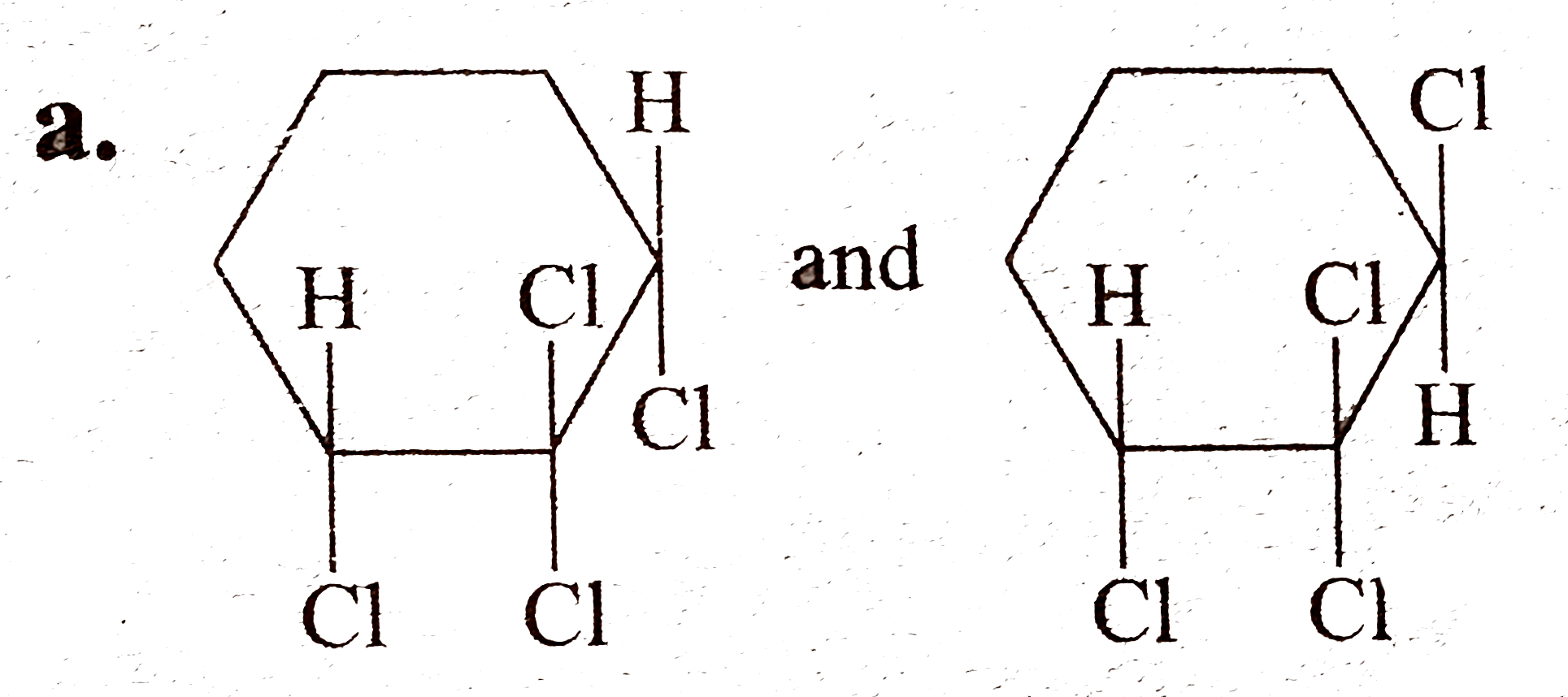 B
B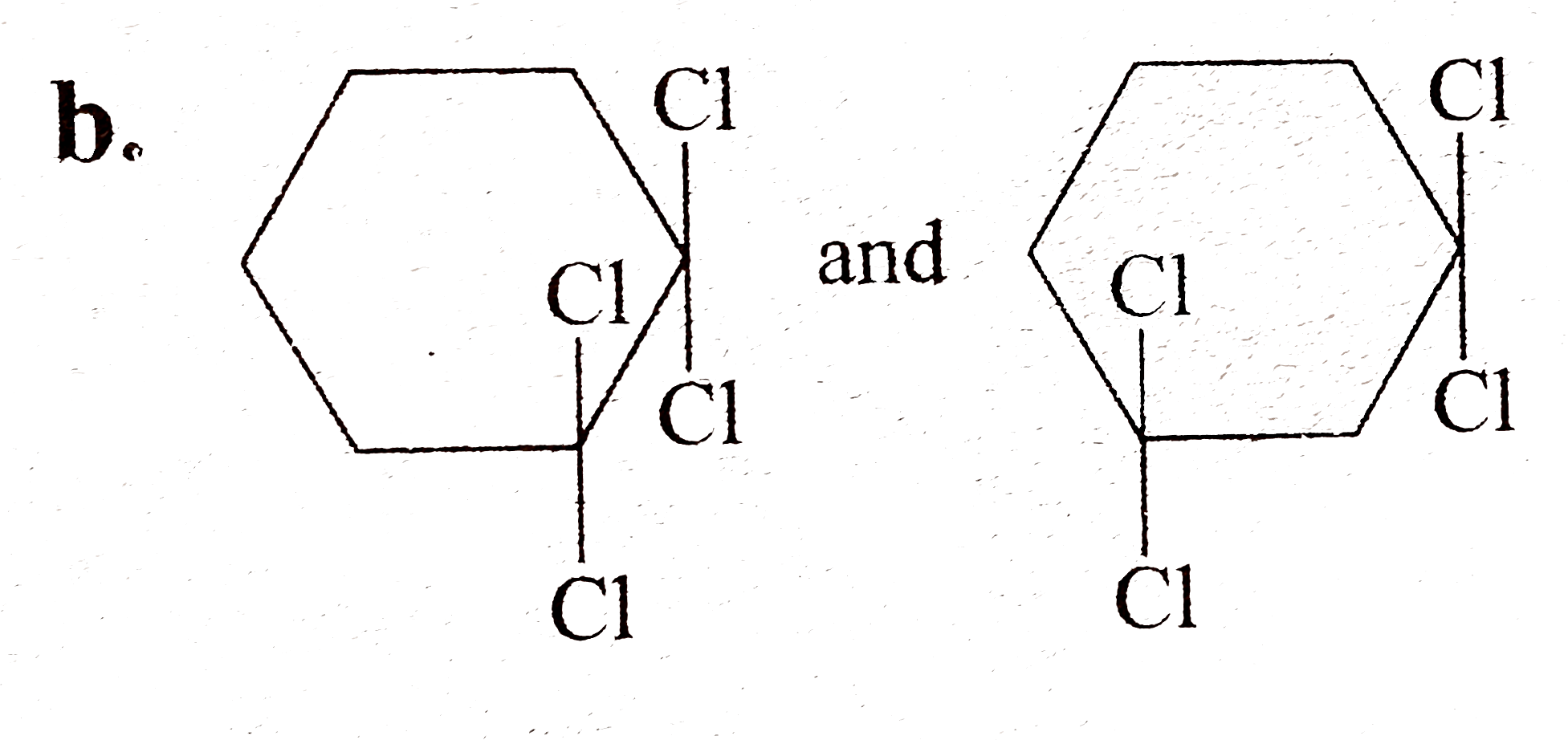 C
C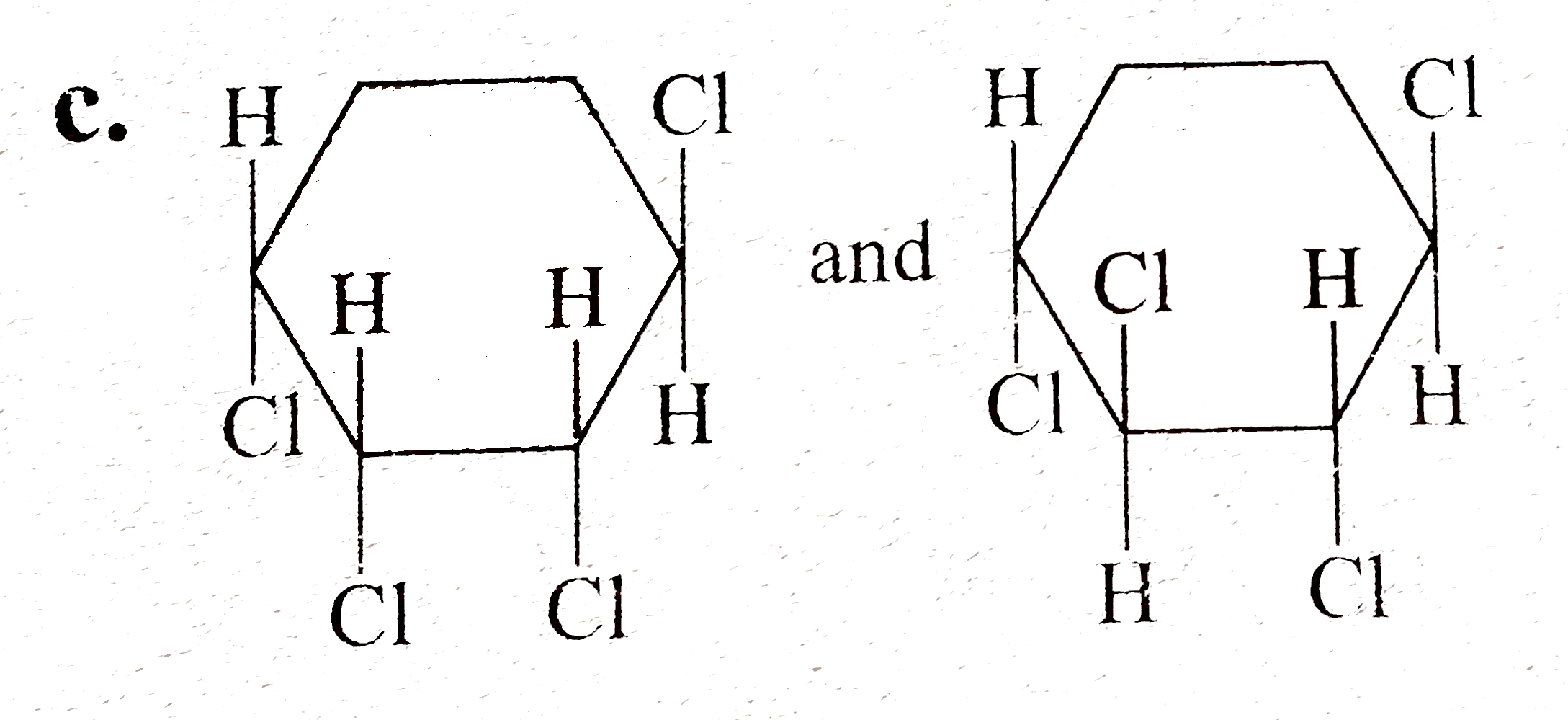 D
D
- Question 2 - Select One
If F0 andFe are the focal lengths of the objective and eye-piece respectively for a Galilean telescope, its magnifying power is about
AF0+FeBF0×FeCF0/FeD12F0+Fe - Question 3 - Select One
If A',B' are complementary events of two independent events A, B, then P(A∪B)=
A1−P(A)P(B')B1−P(A')P(B)C1−P(A')P(B')DP(A')+P(B')
If ¯¯¯E & ¯¯¯F are complementary events of events E&F respectively then P(EF)+P(¯¯¯EF)
View SolutionIf A and B are two complementary events, then what is the relation between P(A) and P(B).
View SolutionIf E and F are independent events with P(E)=p and P(F)=2p and P(exactly one of E,F) =56 then find the value of p
View SolutionIf E and F are two independent events then prove that E and F' are also independent events.
View SolutionUnits of van der Waal's constants a and bare respectively
View Solution

