Find the shortest distance of the point (0,c) from the parabola y=x2,where0≤c≤5.
Video Solution
Text Solution
The correct Answer is:√c−14,12≤c≤5
Let the point Q (x,x2) on x2=y whose distance from (0,c) is minimum.
Now PQ2=x2+(x2−c)2
Let f(x)=x2+(x2−c)2
f'(x)=2x+2(x2−c)2x
=2x(1+2x2−2c)=4x(x2−c+12)
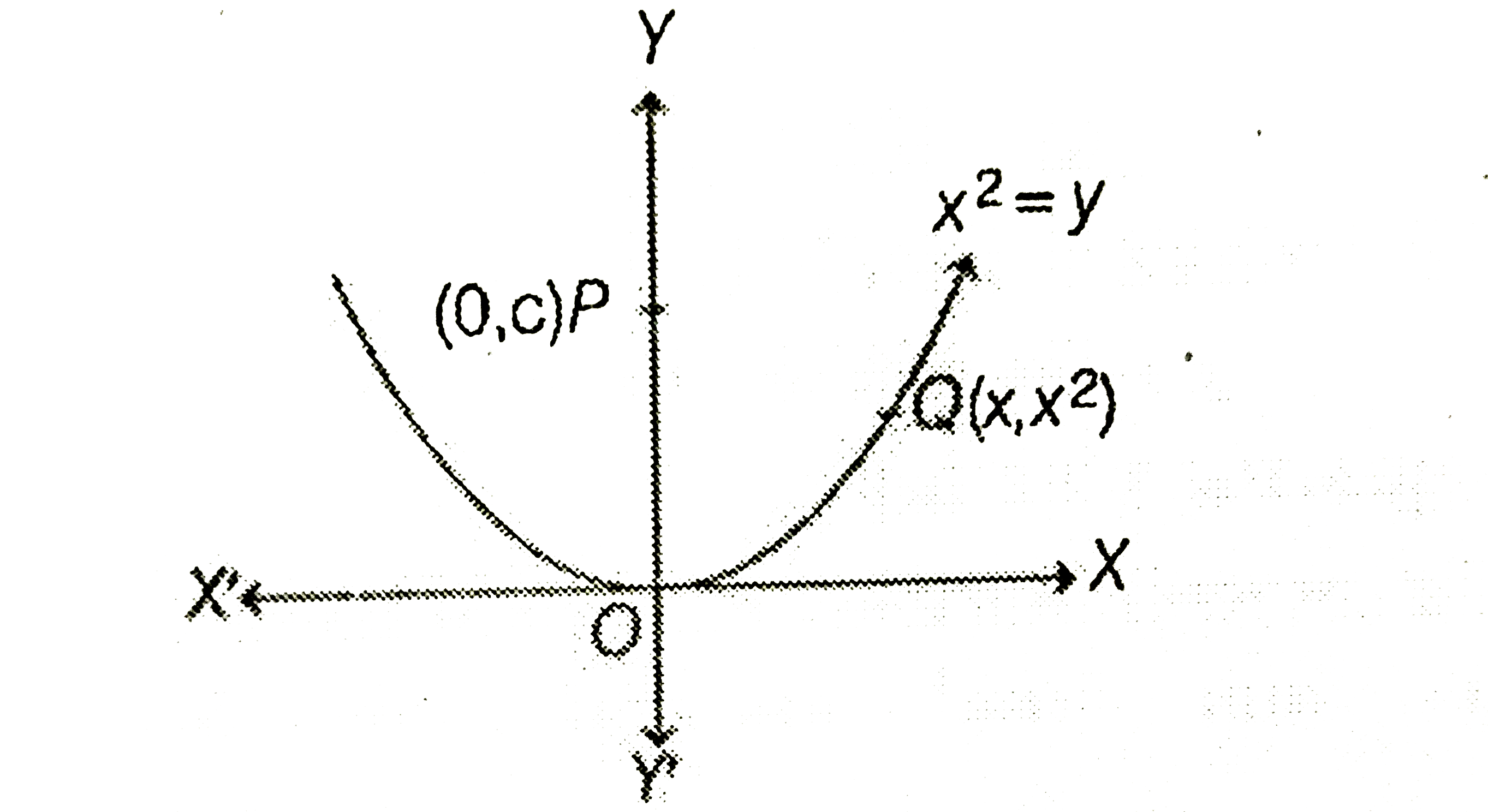
4x(x−√c−12)(x+√c−12), when c>12
For maxima put f'(x)=0
Now, f''(x)=4[x2−c+12]+4x[2x]
At x=±√c−12
f''(x)≥0
∴f(x) is minimum
Hence, minimum value of f(x)=|PQ|
⎷(√c−12)2+((srt(c−12))2−c)2
=√c−12+(c+12−c)2=√c−14,12≤c≤5
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - PARABOLAEXERCISE - TOPIC 2 EQUATION OF TANGENTS AND PROPERTIES (INTERGER)1videos
PARABOLA
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - PARABOLAEXERCISE - TOPIC 3 EQUATION OF NORMAL AND PROPERTIES OBJECTIVES QUESTIONS I (ONLY ONE CORRECT OPTION )2videos
PARABOLA
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - PARABOLAEXERCISE - TOPIC 2 EQUATION OF TANGENTS AND PROPERTIES (FILL IN THE BLANK )1videos
PARABOLA
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - MISCELLANEOUSEXERCISE - MISCELLANEOUS87videos
MISCELLANEOUS
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Dearrangement and Number of Divisors (Fill in the Blank )1videos
PERMUTATIONS AND COMBINATIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
The shortest distance of the point (1, 3, 5) from
x2+y2=0 isA5 unitsBsqrt(10) unitsC2sqrt(5) unitsD5√2 units
Find the distance of the point (2, 3, -5) from the plane x+2y−2z−9=0.
View SolutionFind the shortest distance between the line y=x−2 and the parabola y=x2+3x+2.
View SolutionFind the shortest distance between the line y=x−2 and the parabola y=x2+3x+2.
View SolutionFind the shortest distance between the line y=x−2 and the parabola y=x2+3x+2
View SolutionFind the distance of the point (21,0) from the plane 2x+y+2z+5=0.
View SolutionThe shortest distance of (0,0) from the curve y=ex+e−x2 is
View SolutionFind the shortest distance between the line x−y+1=0 and the curve y2=x.
View Solution

