Match the conditions/expression in Column I With statement in Column II. Normals at P,Q,R are drawn to y2=4x which intersect at (3,0). Then,
column Icolumn IIA.Area ofΔPQRp.2B.Radius of circumcircle ofΔPQRq.52C.Centroid ofΔPQRr.(52,0)D.Circumcentre ofΔPQRs.(23,0)
The correct Answer is:A→p;B→q:C→s;D→r
since, equation of normal to the parabola y2=4ax is y+xt=2at+at3 passes through (3,0)
⇒3t=2t+t2
⇒t=0,1−1
∴ Coordinates of the normals are P(1,2),Q(0,0)R(1,−2)
Thus,
A. Area of ΔPQR=12×1×4=2
C. Centriod of ΔPQR=(23,0)
Equation of circle passing through P,Q,R is
(x - 1)(x -1) + (y - 2) (y + 2) + λ (x - 1) = 0
⇒1−4−λ=0
⇒λ=−3
∴ Required equation of circle is
x2+y2−5x=0
∴ Centre (52,0) and radius 52.
Topper's Solved these Questions
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - PARABOLAEXERCISE - TOPIC 3 EQUATION OF NORMAL AND PROPERTIES OBJECTIVES QUESTIONS II (ONE OR MORE THAN ONE CORRECT OPTION )4videos
PARABOLA
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - PARABOLAEXERCISE - TOPIC 3 EQUATION OF NORMAL AND PROPERTIES OBJECTIVES QUESTIONS II (INTEGER )1videos
PARABOLA
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - PARABOLAEXERCISE - TOPIC 3 EQUATION OF NORMAL AND PROPERTIES OBJECTIVES QUESTIONS I (ONLY ONE CORRECT OPTION )2videos
PARABOLA
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - MISCELLANEOUSEXERCISE - MISCELLANEOUS87videos
MISCELLANEOUS
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Dearrangement and Number of Divisors (Fill in the Blank )1videos
PERMUTATIONS AND COMBINATIONS
Similar Questions
For y2=4x, pormals at P, Q, Rare concurrent at a point (3,0), then for ΔPQR
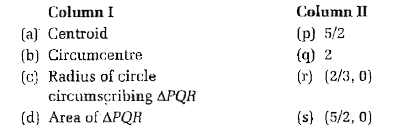 View Solution
View SolutionMatch the statement in Column I with the statements in Column II. One or more than one choice from Column II can match with a statement from Column I.
Column IColumn II(a)Young's modulus(p)−ΔVρV(b)Bulk modulus(q)FlAΔl(c)Compressibility(r)−Δd/dΔDl/l(d)Poisson's ratio(s)−ρVΔVView Solution
Knowledge Check
- Question 1 - Select One
If the normals at P, Q, R on the rectangular hyperbola xy = c2 intersect at a point S on the hyperbola, then centroid of the triangle PQR is at
Ait meets the conjugate hyperbola in imaginary pointsBthe conjugate diameter meets the given hyperbola in real pointsCthe conjugate diameter meets the conjugate hyperbola in imaginary pointsDnone of these - Question 2 - Select One
The normals at three points P,Q,R of the parabola y2=4ax meet in (h,k) The centroid of triangle PQR lies on (A)x=0(B)y=0(C)x=-a(D)y=a
Ax = 0By = 0Cx = -aDy = a - Question 3 - Select One
The normals at three points P,Q and R of the parabola y2=4ax meet at (h,k). The centroid of the △PQR lies on
Ax=0By=0Cx=-aDy=a
From the point (3 0) 3- normals are drawn to the parabola y2=4x. Feet of these normal are P ,Q ,R then area of triangle PQR is
View SolutionNormals at points P, Q and R of the parabola y2=4ax meet in a point. Find the equation of line on which centroid of the triangle PQR lies.
View SolutionNormals at points P, Q and R of the parabola y2=4ax meet in a point. Find the equation of line on which centroid of the triangle PQR lies.
View SolutionMatch the elements given in column (I) with the most appropriate expected values given in column (II)
Column IColumn IIElementElectronegative valuesA.F(p)3.5B.O(q)3.0C.N(r)4.0D.C(s)2.5View SolutionMatch the elements given in column (I) with the most appropriat expected values given in column II
Column IColumn IIElementElectronegative valuesA.F(p)3.5B.O(q)3.0C.N(r)4.0D.C(s)2.5View Solution

