Three wheels can completed respectively 60, 36, 24 revolutions per minute . There is a red spot on each wheel that touches the ground at time zero . After how much time , all these spots will simultaneously touch the ground again ?
5/2 seconds
5/3 seconds
5 seconds
7.5 seconds
The correct Answer is:C
To solve the problem of when all three red spots on the wheels will touch the ground again, we need to determine the time it takes for each wheel to complete one full revolution and then find the least common multiple (LCM) of these times.
1. Identify the Revolutions per Minute (RPM):
- Wheel A: 60 revolutions per minute (RPM)
- Wheel B: 36 revolutions per minute (RPM)
- Wheel C: 24 revolutions per minute (RPM)
2. Convert RPM to Revolutions per Second:
- For Wheel A:
Revolutions per second=60 RPM60=1 revolution/second
- For Wheel B:
Revolutions per second=36 RPM60=0.6 revolutions/second
- For Wheel C:
Revolutions per second=24 RPM60=0.4 revolutions/second
3. Calculate Time for One Revolution:
- For Wheel A:
Time for one revolution=11=1 second
- For Wheel B:
Time for one revolution=10.6=53 seconds
- For Wheel C:
Time for one revolution=10.4=52 seconds
4. Find the Least Common Multiple (LCM):
- We need to find the LCM of the times for one revolution:
- 1 second
- 53 seconds
- 52 seconds
- To find the LCM, we first convert all times to a common fraction:
- 1=66
- 53=106
- 52=156
- The LCM of the numerators (6, 10, 15) can be calculated:
- The prime factorization is:
- 6=2×3
- 10=2×5
- 15=3×5
- The LCM is 21×31×51=30.
- Now, since we have the LCM of the numerators, we divide by the common denominator (6):
LCM=306=5 seconds
5. Conclusion:
- Therefore, all three red spots will simultaneously touch the ground again after 5 seconds.
- Wheel A: 60 revolutions per minute (RPM)
- Wheel B: 36 revolutions per minute (RPM)
- Wheel C: 24 revolutions per minute (RPM)
- For Wheel A:
Revolutions per second=60 RPM60=1 revolution/second
- For Wheel B:
Revolutions per second=36 RPM60=0.6 revolutions/second
- For Wheel C:
Revolutions per second=24 RPM60=0.4 revolutions/second
- For Wheel A:
Time for one revolution=11=1 second
- For Wheel B:
Time for one revolution=10.6=53 seconds
- For Wheel C:
Time for one revolution=10.4=52 seconds
- We need to find the LCM of the times for one revolution:
- 1 second
- 53 seconds
- 52 seconds
- 1=66
- 53=106
- 52=156
- The prime factorization is:
- 6=2×3
- 10=2×5
- 15=3×5
- The LCM is 21×31×51=30.
LCM=306=5 seconds
- Therefore, all three red spots will simultaneously touch the ground again after 5 seconds.
Topper's Solved these Questions
Similar Questions
Three wheels can complete 40, 24 and 16 revolutions per minute respectively. There is a red spot on each wheel that touches the ground at time zero. After how much time, all these spots will simultaneously touch the ground again? 712 sec (b) 18 sec (c) 712 min (d) 18 min
View SolutionThe radii of the front wheel and the rear wheel of a bike are 14 cm and 21 cm respectively. Rahul puts a red mark on the point of contact of each of the wheels with the ground when the bike is stationary. Once the bike start moving, then after what distance will the two red marks touch the ground again simultaneously? (a) 42 cm (b) 84 cm (c) 264 cm (d) 294 cm
View Solution
Knowledge Check
Three wheels can completed respectively 60, 36, 24 revolutions per minute . There is a red spot on each wheel that touches the ground at time zero . After how much time , all these spots will simultaneously touch the ground again ?
A52 sB53 sC6sD7.5 sThe circumferences of the fore and hind wheels of a carriage are (6)314metres and (8)118 meters respectively .At any given moment a chalk mark is put on the point of contact of each wheel with the ground. Find the distance travelled by the carriage so that both the chalk marks are again on the ground at the same time-
A218 mB217.5 mC218.5 mD217 mTwo pipe A and B can separately fill a cistern in 60 minutes and 75 minutes respectively . There is a third pipe at the bottom of the cistern to empty it, if all the three pipes are simultaneously opened, then the cistern is filled in 50 minutes . In how much time can the third pipe alone empty the cistern ?
A110 minutesB100 minutesC120 minutesD90 minutes
Two taps can fill a tank in 15 and 12 minutes respectively. A third tap can empty it in 20 minutes. If all the taps are opened at the same time, then in how much time will the tank be filled ?
View SolutionA ball is thrown vertically upward with velocity 20m/s from a tower of height 60 m. After how much time and with what velocity will it strike the ground?
View SolutionParticles P and Q of mass 20g and 40g respectively are simu ltaneously proejected from points A and B on the ground. The initial velocities of P and Q make 45∘ and 135∘ angles respectivley with the horizontal AB as shown in the Fig. 5.44 Each particle has an initial speed of 49m/s . the separation AB is 249m. both particles travel in the same vertical plane and undergo a collision. After collision P retraces its path. Determine the position of q when it hits the grou.d How much time after the collision does the particle Q take to reach the ground? (Take g=9.8m/s2)
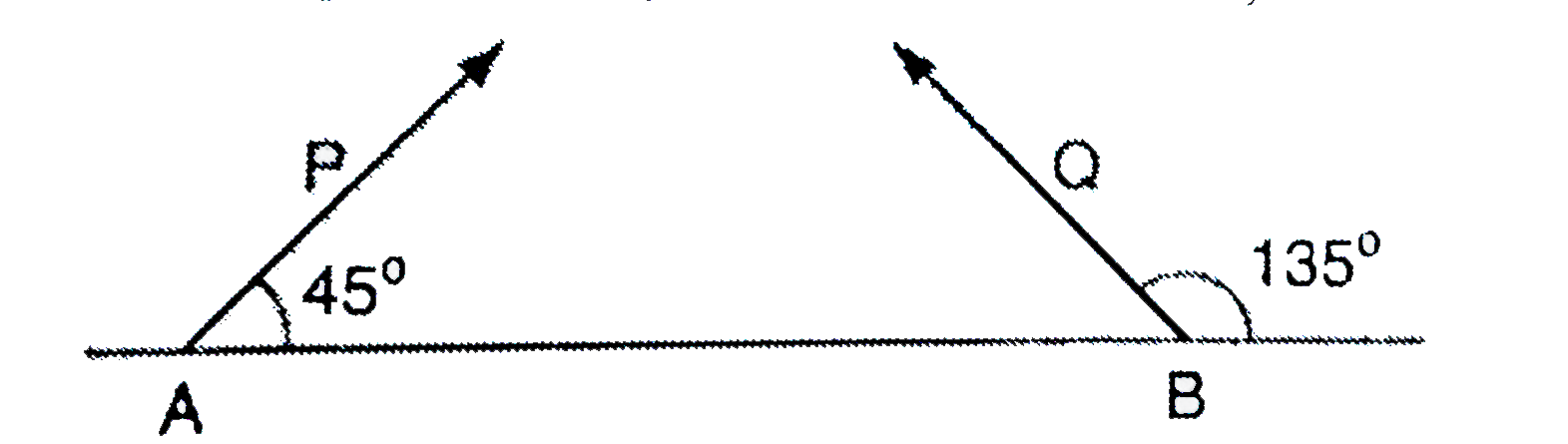
View SolutionThe cicumferences of a fore and hind wheels of a carriage are 6314m and 8118m respectively. At any given moment, a chalk mark is put on the point of contact of each wheel with the ground. Find the distance travlled by the carriage so that both the chalk marks are again on the ground at the same time
View SolutionTwo pipes A and B can fill a tank in 60 min. and 75 min. respectively. There is also awaste pipe in the tank (cistern). When all the three are opened, the cistern get filled in 50 minutes. How long will the waste pipe take to empty the full cistern.
View Solution
