A banknote which has become dirty due to udage ad includes a two pices note pasted together where in both the pieces presented belong to the same note, and form the entire note, is termed as .
Counterfeit banknote
imperfect bankote
Soiled banknote
Mutilated banknote
The correct Answer is:C
Step by step video, text & image solution for A banknote which has become dirty due to udage ad includes a two pices note pasted together where in both the pieces presented belong to the same note, and form the entire note, is termed as . by General Knowledge experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Two organ pipes are identical except that one is filled with oxygen and other with a mixture of oxgen and nitrogen. The temperature and total pressure in each pipe are the same when two are sounded together a note of 440Hz is heard, which beats one every second. What is the percentage partial pressure of the nitrogen in the mixture ? Assume that the molecular weight of oxygen is 32 and of nitrogen 28, and their principal specific heats are identical.
View SolutionThe simplest method of welding two pieces of metal together is known as pressure welding. The ends of metal are heated to a white heat-for iron, the welding temperature should be about 1300∘C-in a flame. At this temperature the metal becomes plastic. The ends are then pressed or hammered together, and the joint is smoothed off. Care must be taken to ensure that the surfaces are thoroughly clean first, for dirt will weaken the weld. Moreover, the heating of iron or steel to a high temperature cause oxidation, and a film of oxide is formed on the heated surfaces. For this reason, a flux is applied to the heated metal. At welding heat, the flux melts, and the oxide particles are dissolved in it together with any other impurities which may be present. The metal surfaces are pressed together, and the flux is squeezed out from the centre of the weld. A number of different types of weld may be used, but for fairly thick bars of metals, a vee-shaped weld should normally be employed. It is rather stronger than the ordinary but weld.
Unless the surfaces are cleaned first
View Solution
Knowledge Check
The trajectory of a body with two simultaneous, mutually perpendicular oscillations is determined by
x=asin(pωt) (1)
y=bsin(qωt+ϕ) (2)
where x and y are the projections of the body displacement on X and Y axes
For simplicity, assume pω=qω=ω0. Then, the equation of trajectory will be
y2b2+x2a2−2xyabcosϕ=sin2ϕ (3)
which is a general equation of ellipse.
But if qω≠pωandp≠q, then the graph of trajectory on the X-Y plane is either a closed curve, whose loop number is defined by the ratio n = p/q, or an open curve.
Note that at the point where the curve reverses along the same trajectory, the velocities along the X-axis and Y-axis become equal to zero simultaneously. The body moving along the curve stops exactly at this moment at a certain point, say P, and then starts moving back,
If p = 2, q = 1 and ϕ=0, the path of trajectory of motion of the body with the above two oscillations (1) and (2) isAan ellipseBa parabolaCa straight lineDnone of the aboveThe trajectory of a body with two simultaneous, mutually perpendicular oscillations is determined by
x=asin(pωt) (1)
y=bsin(qωt+ϕ) (2)
where x and y are the projections of the body displacement on X and Y axes
For simplicity, assume pω=qω=ω0. Then, the equation of trajectory will be
y2b2+x2a2−2xyabcosϕ=sin2ϕ (3)
which is a general equation of ellipse.
But if qω≠pωandp≠q, then the graph of trajectory on the X-Y plane is either a closed curve, whose loop number is defined by the ratio n = p/q, or an open curve.
Note that at the point where the curve reverses along the same trajectory, the velocities along the X-axis and Y-axis become equal to zero simultaneously. The body moving along the curve stops exactly at this moment at a certain point, say P, and then starts moving back,
The closed curve for the motion of the body with the above two oscillations (1) and (2) with certain values of p, q and ϕ is given in the adjacent figure. The value of p/q is
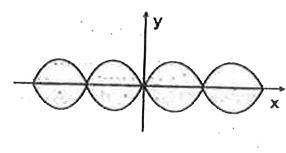 A1/2B3/2C1/4D5/2
A1/2B3/2C1/4D5/2The concept of hybridisation has been introduced to explain the shapes of molecules. It involves the intermixing of two or more atomic orbitals belonging to same atom but in or more atomic orbitals beloging to sasme atom but in different sub-shells so as to intermix and redistibute energies to from equivalent orbitals called hybrid orbitals.Depending upon toh enumber and nature of the orbitals involved, the hybridisation may be divided into sp (linear), sp2 (trigonal), sp3 (tetrahedral), sp3d (trigonal bipyramidal), sp3d3 (octahedral) and sp3d3 (pentagonal bipyramidal) types. it may be noted that the orbitals of isolated atoms never hybridise and they do so at the time of bond formation.
The hybrid state of carbon in C2H2 is same as that of carbon in:AC2H6BCO2CBenzeneDC (diamond).
The simplest method of welding two pieces of metal together is known as pressure welding. The ends of metal are heated to a white heat-for iron, the welding temperature should be about 1300∘C-in a flame. At this temperature the metal becomes plastic. The ends are then pressed or hammered together, and the joint is smoothed off. Care must be taken to ensure that the surfaces are thoroughly clean first, for dirt will weaken the weld. Moreover, the heating of iron or steel to a high temperature cause oxidation, and a film of oxide is formed on the heated surfaces. For this reason, a flux is applied to the heated metal. At welding heat, the flux melts, and the oxide particles are dissolved in it together with any other impurities which may be present. The metal surfaces are pressed together, and the flux is squeezed out from the centre of the weld. A number of different types of weld may be used, but for fairly thick bars of metals, a vee-shaped weld should normally be employed. It is rather stronger than the ordinary but weld.
The flux is used to
View SolutionThe simplest method of welding two pieces of metal together is known as pressure welding. The ends of metal are heated to a white heat-for iron, the welding temperature should be about 1300∘C-in a flame. At this temperature the metal becomes plastic. The ends are then pressed or hammered together, and the joint is smoothed off. Care must be taken to ensure that the surfaces are thoroughly clean first, for dirt will weaken the weld. Moreover, the heating of iron or steel to a high temperature cause oxidation, and a film of oxide is formed on the heated surfaces. For this reason, a flux is applied to the heated metal. At welding heat, the flux melts, and the oxide particles are dissolved in it together with any other impurities which may be present. The metal surfaces are pressed together, and the flux is squeezed out from the centre of the weld. A number of different types of weld may be used, but for fairly thick bars of metals, a vee-shaped weld should normally be employed. It is rather stronger than the ordinary but weld.
For fairly thick bars of metals
View SolutionThe simplest method of welding two pieces of metal together is known as pressure welding. The ends of metal are heated to a white heat-for iron, the welding temperature should be about 1300∘C-in a flame. At this temperature the metal becomes plastic. The ends are then pressed or hammered together, and the joint is smoothed off. Care must be taken to ensure that the surfaces are thoroughly clean first, for dirt will weaken the weld. Moreover, the heating of iron or steel to a high temperature cause oxidation, and a film of oxide is formed on the heated surfaces. For this reason, a flux is applied to the heated metal. At welding heat, the flux melts, and the oxide particles are dissolved in it together with any other impurities which may be present. The metal surfaces are pressed together, and the flux is squeezed out from the centre of the weld. A number of different types of weld may be used, but for fairly thick bars of metals, a vee-shaped weld should normally be employed. It is rather stronger than the ordinary but weld.
The simplest way of welding two pieces of metal together is
View SolutionThe simplest method of welding two pieces of metal together is known as pressure welding. The ends of metal are heated to a white heat-for iron, the welding temperature should be about 1300∘C-in a flame. At this temperature the metal becomes plastic. The ends are then pressed or hammered together, and the joint is smoothed off. Care must be taken to ensure that the surfaces are thoroughly clean first, for dirt will weaken the weld. Moreover, the heating of iron or steel to a high temperature cause oxidation, and a film of oxide is formed on the heated surfaces. For this reason, a flux is applied to the heated metal. At welding heat, the flux melts, and the oxide particles are dissolved in it together with any other impurities which may be present. The metal surfaces are pressed together, and the flux is squeezed out from the centre of the weld. A number of different types of weld may be used, but for fairly thick bars of metals, a vee-shaped weld should normally be employed. It is rather stronger than the ordinary but weld.
When iron is heated to about 1300 degree centigrade
View SolutionThe concept of hybridisation has been introduced to explain the shapes of molecules. It involves the intermixing of two or more atomic orbitals belonging to same atom but in or more atomic orbitals beloging to sasme atom but in different sub-shells so as to intermix and redistibute energies to from equivalent orbitals called hybrid orbitals.Depending upon toh enumber and nature of the orbitals involved, the hybridisation may be divided into sp (linear), sp2 (trigonal), sp3 (tetrahedral), sp3d (trigonal bipyramidal), sp3d3 (octahedral) and sp3d3 (pentagonal bipyramidal) types. it may be noted that the orbitals of isolated atoms never hybridise and they do so at the time of bond formation.
The hybridisation of phostphorus in PCOl3 is the same as:
View Solution
