A stone is falling from a mountain. The velocity of the stone is given by v = 9.8 t. Draw its graph and find the ve locity of the stone 4 seconds after start.Solution in Telugu
Step by step video & image solution for A stone is falling from a mountain. The velocity of the stone is given by v = 9.8 t. Draw its graph and find the ve locity of the stone 4 seconds after start. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - NCERT TELUGUCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - EXERCISE 8.4
12 Videos
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - NCERT TELUGUCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - EXERCISE 8.5
6 Videos
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - NCERT TELUGUCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - EXERCISE 8.2
6 Videos
- LINEAR EQUATION IN TWO VARIABLESBOOK - NCERT TELUGUCHAPTER - LINEAR EQUATION IN TWO VARIABLESEXERCISE - EXERCISE
143 Videos
- LINES AND ANGLES BOOK - NCERT TELUGUCHAPTER - LINES AND ANGLES EXERCISE - THINK, DISCUSS AND WRITE
3 Videos
Similar Questions
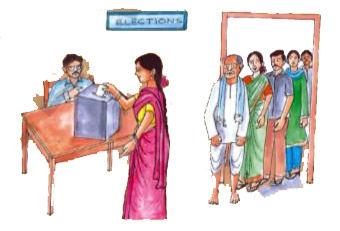
In a election 60% of voters cast their votes. Form an equation and draw the graph for this data. Find the following from the graph.
(i) The total number of voters, if 1200 voters cast their votes
(ii) The number votes cast, if the total number of voters are 800
View SolutionGive the graphical representation of the following equation.
(a) On the number line and (b)On the Cartesian plane
(i) x = 3 (ii) y + 3 = 0 (iii) y = 4 (iv) 2x – 9 = 0
(v) 3x + 5 = 0
View SolutionFind three different solutions of the each of the following equations.
(i) 3x + 4y = 7 (ii) y = 6x (iii) 2x - y = 7
(iv) 13x - 12y = 25 (v) 10x + 11y = 21 (vi) x + y = 0
View SolutionWrite any three linear equations in two variables and any three solutions for each of them.
View SolutionWhat is the difference between intersecting lines and concurrent lines ?
View SolutionA stone is let to fall from a balloon ascending with acceleration 4ms2. After time t=2s, a second stone is dropped. If the distance between the stones after time (t′=3s) since the second stone is dropped is d, find d28
View SolutionA stone is dropped form certain height which can reach the ground in 5s. If the stone is stopped after 3s of its fall and then allowed to fall again. Find the time taken (in second) by the stone to reach the ground for the remaining distance.
View Solutionपानी पर बने 44.1 मी ऊॅंचे एक पुल से एक पत्थर को पानी में गिराया जाता है| 1 सेकण्ड के पश्चात दूसरे पत्थर को ऊर्ध्वाधर नीचे की ओर फेंका जाता है, दोनों पत्थर एक साथ पानी में गिरते हैं | दूसरे पत्थर का प्रारम्भिक वेग होगा
View Solutionकिसी पत्थर को किसी भवन की छतके किनारे से गिराया गया
गिरने के 1 सेकंड तथा २ सेकंड पश्चात पत्थर का त्वरण कितना था
View SolutionA stone is falling from a mountain. The velocity of the stone is given by V = 9.8t. Draw its graph and find the velocity of the stone ‘4’ seconds after start.
View SolutionA stone is falling from a mountain. The velocity of the stone is given by V = 9.8t. Draw its graph and find the velocity of the stone ‘4’ seconds after start.
View SolutionA stone is falling from a mountain. The velocity of the stone is given by V = 9.8t. Draw its graph and find the velocity of the stone ‘4’ seconds after start.
View SolutionA stone is falling from a mountain. The velocity of the stone is given by V = 9.8t. Draw its graph and find the velocity of the stone ‘4’ seconds after start.
View SolutionA balloon starting from rest moves vertically upwards with an acceleration (g/8) ms−1.If a stone falls from it after 8 seconds from the start,find time taken by the stone to reach the ground.
View Solutionएक पत्थर 15m/s वेग से क्षैतिज रूप से फेका जाता है । 1 सेकंड में पत्थर का स्पर्शी तथा अभिलम्ब त्वरण इसके गति प्रारम्भ करने के बाद ज्ञात कीजिये।
View SolutionA balloon starting from rest moves vertically upwards with an acceleration (g//8) ms^(-2) . If a stone falls from it after 8 seconds from the start, find the time taken by the stone to reach the ground.
View Solution
