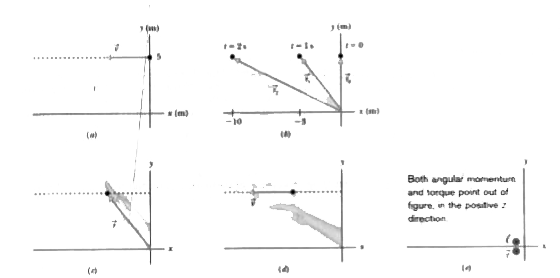
Figure shows a freeze-frame of a 0.500 kg particle moving along a straight line with a position vector given by
,
With in meters and t in seconds, starting at t = 0. The position vector points from the origin to the particle. In unit-vector notation, find expressions for the angular momentum of the particle and the torque acting on the particle, both algebraic signs in terms of the particle's motion.
(1) The point about which an angular momentum of a particle is to be calculated must always be specified. Here it is the origin. (2) The angular momentum of a particle is given by Eq. . (3) The sign associated with a particle.s angular momentum is set by the sense of rotation of the particle.s position vector as the particle moves: clock-wise is negative and counterclockwise is positive. (4) If the torque acting on a particle and the angular momentum of the particle are calculated around the smae point, then the torque is related to angular momentum by Eq. .
Calculations: In order to use Eq. to find the angular momentum about the origin, we first must find an expression for the particle.s velocity by taking a time derivative of its position vector. Following Eq. ,
= ,
with in meters per second.
Next, let.s take the cross product of using the template for cross products displayed in Eq.
Here the generic is and the generic is . However, because we really do not want to do more work than needed, let.s first just think about our substitutions into the generic cross product. Because lacks any z component and because lacks any y or z component, the only nonzero term in the generic cross product is the very last one . So, let.s cut to the chase by writing
Note that, as always, the cross product produces a vector that is perpendicular to the original vectors.
To finish up Eq. we multiply by the mass, finding
= .
The torque about the origin then immediately follows from Eq.
=
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS - IEXERCISE - CHECKPOINT18videos
RIGID BODY DYNAMICS - I
- BOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS - IEXERCISE - PROBLEMS100videos
RIGID BODY DYNAMICS - I
- BOOK - RESNICK AND HALLIDAYCHAPTER - RELATIVITYEXERCISE - PRACTICE QUESTIONS (Integer Type)5videos
RELATIVITY
- BOOK - RESNICK AND HALLIDAYCHAPTER - RIGID BODY DYNAMICS-IIEXERCISE - PRACTICE QUESTIONS (Integer Type)2videos
RIGID BODY DYNAMICS-II
Similar Questions
A particle that is moving in an xy plane has a position vector given by →r=(3.00t3−6.00t)ˆi+(7.00−8.00t4)ˆj, where →r is measued in meters and t is measured in seconds. For t = 3.00 s, in unitvector notation, find (a) →r, (b) →v, and ( c) →a. (d) Find the angle between the positive direction of the x axis and a line that is tangent to the path of the particle at t = 3.00 s.
View SolutionThe position of a particle is given by →r=(8tˆi+3t2ˆj+5ˆk)m where t is measured in second and →r in meter. Calculate, the velocity and the acceleration of the particle.
View Solution
Knowledge Check
- Question 1 - Select One
The position of a particle is given by →r=2t2ˆi+3tˆj+4ˆk where t is in second and the coefficients have proper units for →r to be in metre. The →a(t) of the particle at t = 1 s is
A4ms−2 along y-directionB3ms−2 along x-directionC4ms−2 along x-directionD2ms−2 along z-direction - Question 2 - Select One
The position of a particle is given by →r=3tˆi+2t2ˆj+5ˆk, where t is in seconds and the coefficients have the proper units for →r to be in meters. The direction of velocity of the particle at t=1 s is
A53∘ with x-axisB37∘ with x-axisC30∘ with y-axisD60∘ with y-axis - Question 3 - Select One
The position of a particle is given by →r=3tˆi+2t2ˆj+5ˆk, where t is in seconds and the coefficients have the proper units for →r to be in metres. The direction of velocity of the particle at t=1 s is
A53∘ with x-axisB37∘ with x-axisC30∘ with y-axisD60∘ with y-axis
The position of a particle is given by →r=(8tˆi+3t2ˆj+5ˆk)m where t is measured in second and →r in meter. Calculate, the velocity and the acceleration of the particle.
View SolutionThe position of a particle is given by →r=(8tˆi+3t2ˆj+5ˆk)m where t is measured in second and →r in meter. Calculate, the velocity and the acceleration of the particle.
View SolutionA 0.150 kg particle moves along an x axis according to x(t)=−13.00+2.00t+4.00t2−3.00t3, with x in meters and t in seconds. In unit-vector notation, what is the net force acting on the particle at t=2.60 s ?
View SolutionAn electron's position is given by →r=3.00ˆi−4.00t2ˆj+2.00ˆk, with t in seconds and →r in meters. (a) In unit-vector notation, what is the electron's velocity →r(t)? At t = 3.00 s, what is →v (b) in unitvector notation and as ( c) a magnitude and (d) an angle relative to the positive direction of the x axis?
View SolutionA particle moves in xy plane with its position vector changing with time (t) as →r=(sint)ˆi+(cost)ˆj ( in meter)
Find the tangential acceleration of the particle as a function of time. Describe the path of the particle.View Solution
