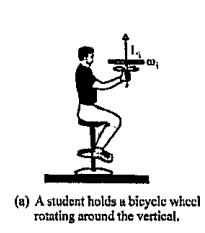
Figure shows a student, again sitting on a stool that can rotate freely about a vertical axis. The student, initially at rest, is holding a bicycle wheel whose rim is loaded with lead and whose rotational inertia $I_{w h}$ about its central axis is $1.2 k g \cdot m^{2}$ .
The wheel is rotating at an angular speed $ω_{w h}$ of 3.9 rev/s, as seen from overhead, the rotation is counterclockwise. The axis of the wheel is vertical, and the angular Momentum ${\to L}_{w h}$ of the wheel points vertically upward.

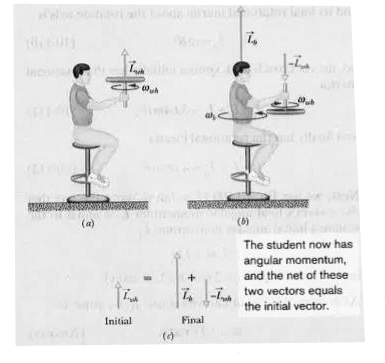
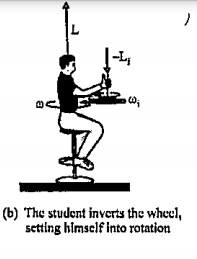
The student now inverts the wheel so that, as seen from overhead, it is rotating clockwise. Its angular momentum is now $- {\to L}_{w h}$ . The inversion results in the student, the stool, and the wheel's center rotating together as a composite rigid body about the stool's rotation axis, with rotational inertia $I_{b} = 6.8 k g \cdot m^{2}$ . With what angular speed $ω_{b}$ and in what direction does the composite body rotate after the inversion of the wheel?

Text Solution

Verified by Experts

1. The angular speed $ω_{b}$ we seek is related to the final angular momentum ${\to L}_{b}$ of the composite body about the stool.s rotation axis by $(L = I ω)$ .
2. The initial angular speed $ω_{w h}$ of the wheel is related to the angular momentum ${\to L}_{w h}$ of the wheel.s rotation about its center by the same equation.
3. The vector addition of ${\to L}_{b} and {\to L}_{w h}$ gives the total angular momentum $L_{tot}$ of the system of the student, stool, and wheel.
4. As the wheel is inverted, no net external torque acts on that system to change ${\to L}_{tot}$ about any vertical axis. So, the system.s total angular momentum is conserved about any vertical axis, including the rotation axis through the stool.
Calculations: The conservation of ${\to L}_{tot}$ is represented with vectors in Fig. We can also write this conservation in terms of components along a vertical axis as
$L_{b f} + L_{w h . f} = L_{b, i} + L_{w h, i}$ .
Where i and f refer to the initial state and the final state. Because inversion of the wheel inverted the angular momentum vector of the wheel.s rotation, we substitute $- L_{w h, i} for L_{w h, f}$ . Then, if we set $L_{b, i} = 0$ .
$L_{b, f} = 2 L_{w h, i}$ .
Using Equation, we next substitute $I_{b} ω_{b} for L_{b . f} and I_{w h} ω_{w h} for L_{w h, i}$ and solve for $ω_{b}$ , finding
$ω_{b} = \frac{2 I_{w h}}{I_{b}} ω_{w h}$
= $\frac{(2) (1.2 k g \cdot m^{2}) (3.9 r e v / s)}{6.8 k g \cdot m^{2}} = 1.4 r e v / s$ .

Updated on:21/07/2023