In the Bohr model of hydrogen atom. What is the de-Broglie wavelength λ for the electron when it is in the (i) n = 1 level (ll) n = 4 level. In each case, compare the de-Broglie wave length to the circumference of the orbit.
Data: n=1, n=4,λ=?Solution in Kannada
By Bohr.s first postulate,
angular momentum of the electron =nh2π
mvr=nh2π
∴2πrn=hmv ...(1)
But, (de brogile wave length),
λ=hmv ...(2)
From the equation (1) and (2)
λ=2πrn ...(3)
(i) n=1,r=r1=0.53Å
∴λ1=2πr1=2π(0.53)Å=3.328Å
From equation(3), λ2πr=1n, for n=1,
λ2πr=1, i.e., λ−2πr
∴ In first orbit, de-brogile wave length of the electron is equal to the circumference of the orbit.
(ii) n = 4, r4=42r1
from equation (3), λ4=2πr44=2π(42r1)4
i.e., λ4=2π×4×(0.53)Å
λ4=13.313Å
and from λ4=2πr44, the de-Brogile wavelength of the electron in the fourth orbit is equal to one fourth of the perimeter.
Topper's Solved these Questions
- BOOK - OSWAAL PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - TOPIC-2 DE-BROGLIE RELATION (LONG ANSWER TYPE QUESTIONS)1videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - OSWAAL PUBLICATIONCHAPTER - CURRENT ELECTRICITY EXERCISE - TOPIC-3 (POTENTIOMETER & APPLICATIONS) (NUMERICAL PROBLEMS)2videos
CURRENT ELECTRICITY
- BOOK - OSWAAL PUBLICATIONCHAPTER - ELECTRIC CHARGES & FIELDSEXERCISE - (TOPIC -2)Numerical Problems5videos
ELECTRIC CHARGES & FIELDS
Similar Questions
What are matter waves? Derive an expression for the de Broglie wave length.
04:52View SolutionA resistance of RΩ draws current from a potentiometer. The potentiometer has a total resistance R0Ω.A voltage V is supplied to the potentiometer. Derive an expression for the voltage across R when the sliding contact is in the middle of the potentiometer.
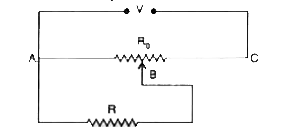 02:05View Solution
02:05View SolutionAn atom was earlier assumed to be sphere of radius a having a positively charged point nucleus of charge +Ze at its centre . This nucleus was believed to be surrounded by a uniform desity of negative charge that made the atom neutral as a whole.
Use this theorem to find the electric field of this atom at a distance r(r>a) from the centre of the atom.01:12View SolutionIn the Bohr model of the hydrogen atom, what is the de-Broglie wavelength for the electron when it is in (a) the n=1 level? (b) Then n=4 level? In each case, compare the de-Broglie wavelength to the circumference 2pir_n of the orbit.
02:12View SolutionConsider an electron in the nth orbit of a hydrogen atom in the Bohr model. The circumference of the orbit can be expressed in terms of the de Broglie wavelength lambda of that electron as
01:30View SolutionConsider an electron in the n^(th) orbit of a hydrogen atom in the Bohr model . The circumference of the orbit can be expressed in terms of the de Broglie wavelength lambda of the electron as :
02:07View Solutionde-Broglie wavelength of an electron in the nth Bohr orbit is λn and the angular momentum is Jn then
03:12View SolutionIf the de Broglie wavelength of the electron in n^(th) Bohr orbit in a hydrogenic atom is equal to 1.5pia_(0)(a_(0) is bohr radius), then the value of n//z is :
03:06View SolutionThe de-Broglie wavelength λnof the electron in the nth orbit of hydrogen atom is
05:42View SolutionAn electron transits for nth orbit to ground in a hydrogen-like atom (Z = 11). The wavelength of emitted radiation, λ is equal to the de Broglie wavelength of electron in nth orbit. Choose the correct options.
02:02View SolutionIn the Bohr model, there is an electron in the orbit of the hydrogen atom. So the circumference of the orbit of that electron can be written in terms of the de-Broglie wavelength.
02:01View SolutionIn the Bohr model of the hydrogen atom, what is the de-Broglie wavelength for the electron when it is in (a) the n=1 level?
(b) Then n=4 level? In each case, compare the de-Broglie wavelength to the circumference 2πrn of the orbit.01:52View Solutionde-Broglie wavelength of an electron in the nth Bohr orbit is lambda_(n) and the angular momentum is J_(n) then
03:09View SolutionConsider an electron in the n^th orbit of a hydrogen atom in the Bohr model. The circumference of the orbit can be expressed in terms of de Broglie wavelength of that electron as
View SolutionConsider an electron in the nth orbit of a hydrogen atom in the Bohr model. The circumference of the orbit can be expressed in terms of the de Broglie wavelength lambda of that electron as
01:20View Solution

