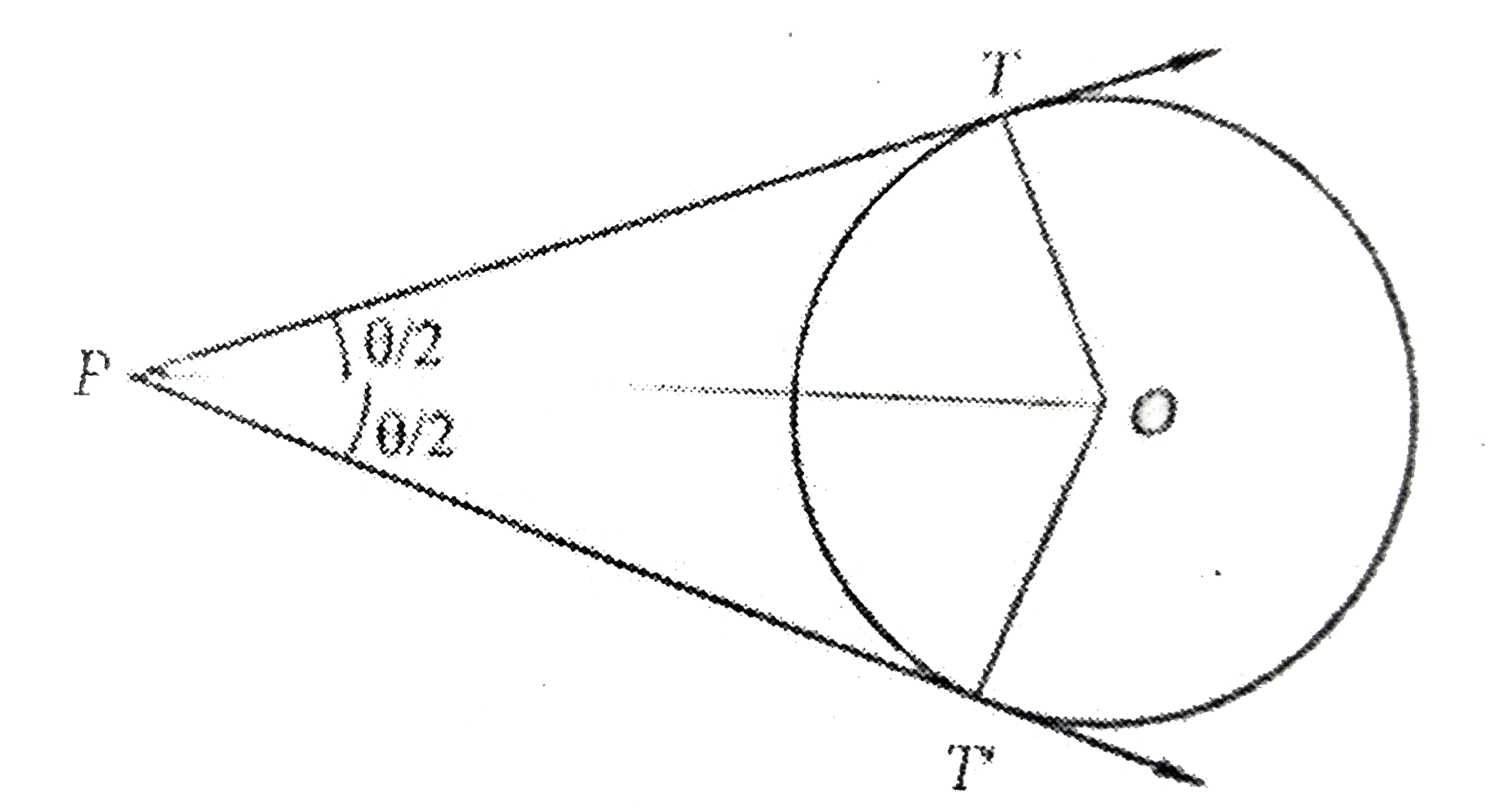
If $S_{1} = α^{2} + β^{2} - a^{2}$ , then angle between the tangents from $(α, β)$ to the circle $x^{2} + y^{2} = a^{2}$ , is

A

$\tan^{- 1} (\frac{a}{\sqrt{S_{1}}})$

B

$2 \tan^{- 1} (\frac{a}{\sqrt{S_{1}}})$

C

$2 \tan^{- 1} (\frac{\sqrt{S_{1}}}{a})$

D

none of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Let PT and PT' be the tangents drawn from $P (α, β)$ to the circle $x^{2} + y^{2} = a^{2}$ , and let $∠ T P T' = θ .$ If O is the centre of the circle, then
$∠ T P O = ∠ T' P O = θ / 2$
$∴ \tan . \frac{θ}{2} = \frac{O T}{O P} = \frac{a}{\sqrt{S_{1}}} \Rightarrow θ = 2 \tan^{- 1} (\frac{a}{\sqrt{S_{1}}}$

|

Updated on:21/07/2023

Class 12 MATHS CIRCLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If two perpendicular tangents can be drawn from the point $(α, β)$ to the hyperbola $x^{2} - y^{2} = a^{2}$ then $(α, β)$ lies on
A $y = \pm x$
B $x^{2} + y^{2} = a^{2}$
C $x^{2} + y^{2} = 2 a^{2}$
D $y^{2} = 4 a x$

OBJECTIVE RD SHARMA-CIRCLES-Chapter Test

Home
Ask Doubt
Profile