Tangents are drawn from the point (h,k) to ^circle $x^{2} + y^{2} = a^{2}$ ; Prove that the area of the triangle formed by them and the straight line joining their point of contact is $\frac{a {(h^{2} + k^{2} - a^{2})}^{\frac{3}{2}}}{h^{2} + k^{2}}$

A

$\frac{{(h^{2} + k^{2} - a^{2})}^{3 / 2}}{h^{2} + k^{2}}$

B

$\frac{a {(h^{2} + k^{2} - a^{2})}^{1 / 2}}{h^{2} + k^{2}}$

C

$\frac{a {(h^{2} + k^{2} - a^{2})}^{1 / 2}}{h^{2} + k^{2}}$

D

$\frac{{(h^{2} + k^{2} - a^{2})}^{3 / 2}}{a (h^{2} + k^{2})}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

The equation of the circle is
$x^{2} + y^{2} = a^{2} ... (i)$
The equation of the chord of contact of tangents drawn from p(h, k) to the circle (i) is
$h x + k y - a^{2} = 0 ... (i i)$
We have to find the area of $Δ P Q R$ .

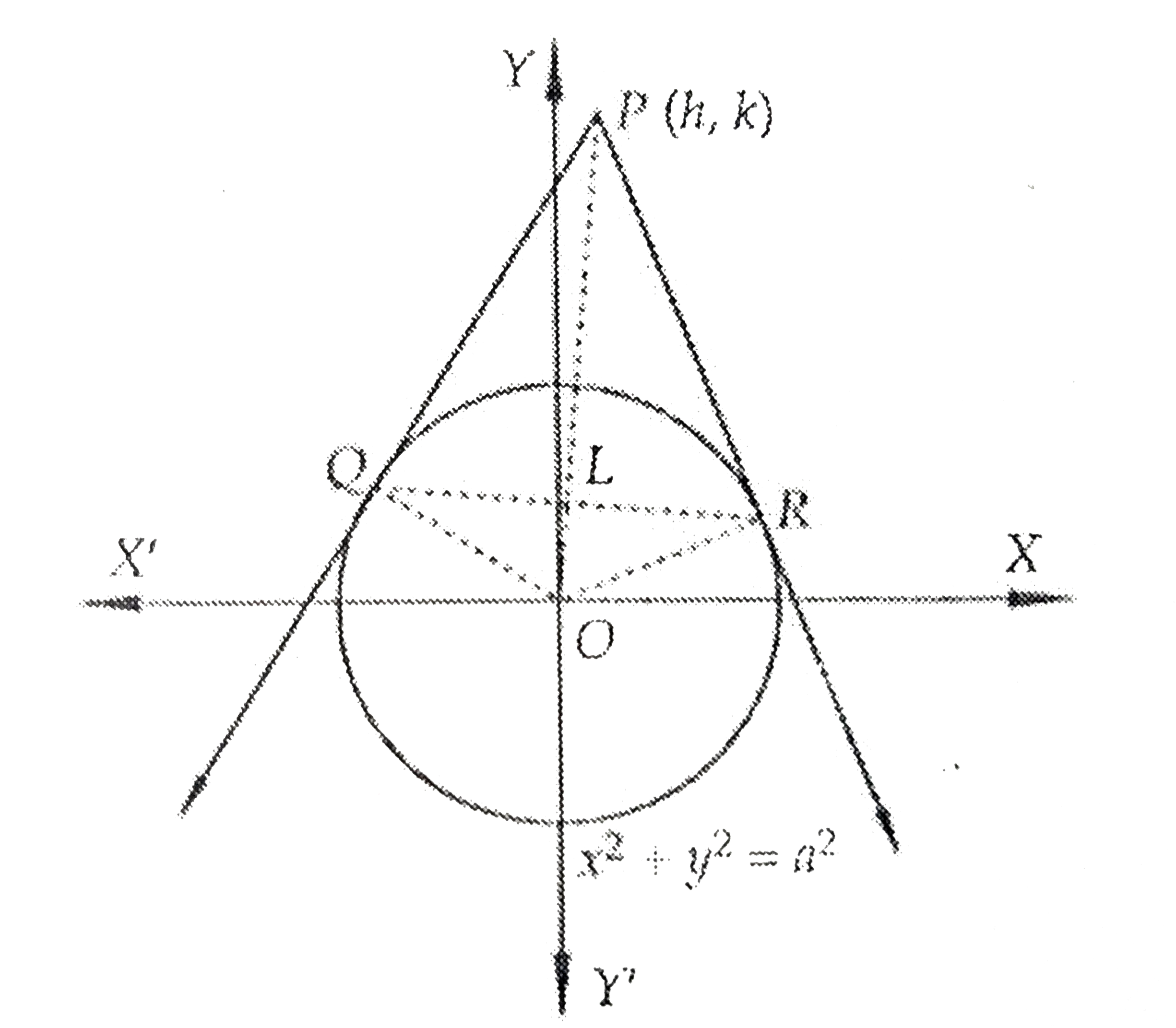
Now,
PL = Length of perpendicular from P(h, k) to (ii)
$\Rightarrow P L = ∣ ∣ ∣ \frac{h^{2} + k^{2} - a^{2}}{\sqrt{h^{2} + k^{2}}} ∣ ∣ ∣$
$\Rightarrow P L = \frac{h^{2} + k^{2} - a^{2}}{\sqrt{h^{2} + k^{2}}} [\begin{matrix} ∵ P (h, k) lies outside x^{2} + y^{2} = a^{2} ∴ h^{2} + k^{2} - a^{2} > 0 \end{matrix}]$
$∴ O L = O P - P L = \sqrt{h^{2} + k^{2}} - \frac{h^{2} + k^{2} - a^{2}}{\sqrt{h^{2} + k^{2}}} = \frac{a^{2}}{\sqrt{h^{2} + k^{2}}}$
In $Δ O L R$ , we have
$L R = \sqrt{O R^{2} - O L^{2}} = \sqrt{a^{2} - \frac{a^{4}}{h^{2} + k^{2}}} = a \sqrt{\frac{h^{2} + k^{2} - a^{2}}{h^{2} + k^{2}}}$
$∴ Q R = 2 L R = 2 a \sqrt{\frac{h^{2} + k^{2} - a^{2}}{h^{2} + k^{2}}}$
$∴$ Area of $Δ P Q R = \frac{1}{2} (Q R \times P L) = \frac{a {(h^{2} + k^{2} - a^{2})}^{3 / 2}}{h^{2} + k^{2}}$

|

Updated on:21/07/2023

Class 12 MATHS CIRCLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The area of the triangle formed by the tangents from the point (4,3) to the circle $x^{2} + y^{2} = 9$ and the line joining their points of contact in sq. units is
A $\frac{132}{25}$
B $\frac{162}{25}$
C $\frac{192}{25}$
Dnone

OBJECTIVE RD SHARMA-CIRCLES-Chapter Test

Home
Ask Doubt
Profile