Find the locus of the midpoint of the chords of the circle x2+y2=a2 which subtend a right angle at the point (c,0).
A
x2+y2=a22
B
x2+y2=2a2
C
x2+y2=a24
D
none of these
Video Solution
Text Solution
The correct Answer is:A
Let (h, k) be the mid-point of a chord AB of the circle x2+y2=a2. Then, the equation of AB is
hx+ky−a2=h2+k2−a2 [Using T = S']
or, hx+ky=h2+k2 ...(i)
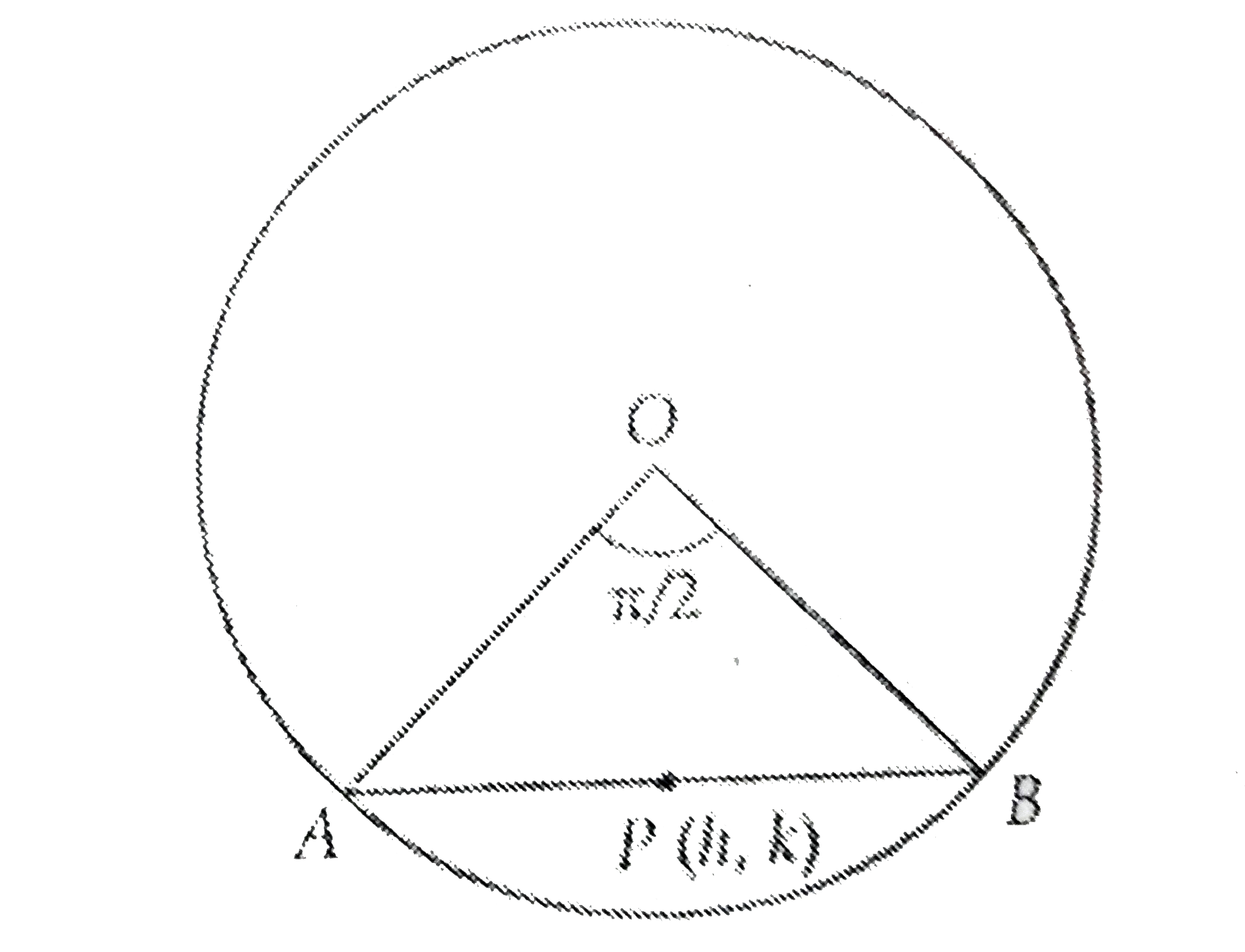
The combined equation of OA and OB is
x2+y2=a2(hx+kyh2+k2)2
or, (h2+k2)2(x2+y2)−a2(hx+ky)2=0
OA and OB will be perpendicular, if
Coeff. of x2+ Coeff. of y2=0
⇒(h2+k2)2−a2h2+(h2+k2)2−a2k2=0
⇒2(h2+k2)−a2(h2+k2)=0⇒2(h2+k2)−a2=0
So, locus of (h, k) is 2(x2+y2)−a2=0.
|
Topper's Solved these Questions
- CIRCLESBOOK - OBJECTIVE RD SHARMACHAPTER - CIRCLESEXERCISE - Section I - Solved Mcqs
108 Videos
- CIRCLESBOOK - OBJECTIVE RD SHARMACHAPTER - CIRCLESEXERCISE - Section-I (Solved MCQs)
1 Videos
- CARTESIAN PRODUCT OF SETS AND RELATIONS BOOK - OBJECTIVE RD SHARMACHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Chapter Test
31 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Chapter Test
59 Videos
Similar Questions
Knowledge Check
The locus of the midpoint of chord of the circle x2+y2=1 which subtends a right angle at the origin is
Ax2+y2=14Bx2+y2=12Cxy=0Dx2−y2=0The locus of the mid -point of the chords of a circle x2+y2=4 ,which subtends a right angle at the centre , is
Ax+y=2Bx2+y2=1Cx2+y2=2Dx−y=0
The locus of the mid point of a chord of the circle x2+y2=4 which subtends a right angle at the origin is
View SolutionThe locus of the midpoint of the chord of the circle x2+y2=4 which subtends a right angle at the origin is?
View SolutionThe locus of the mid-points of a chord of the circle x2+y2=4 which subtends a right angle at the origin is
View SolutionFind the locus of the mid point of the circle x2+y2=a2 which subtend a right angle at the point (p,q)
View SolutionThe locus of the mid-point of the chords of the circle x2+y2−4x−6y−3=0 which subtend a right angle at the point(h, k), is
View SolutionThe locus of the mid points of a chord the circle x2+y2=18 which subtends right angle at the origin is
View Solution
