Let C be the circle with centre at (1, 1) and radius = 1. If T is the circle centred at (0, y), passing through origin and touching the circle C externally, then the radius of T is equal to (1) √3√2 (2) √32 (3) 12 (3) 14
12
14
√32
√32
The correct Answer is:B
Clearly, circles C and T touch each other externally.
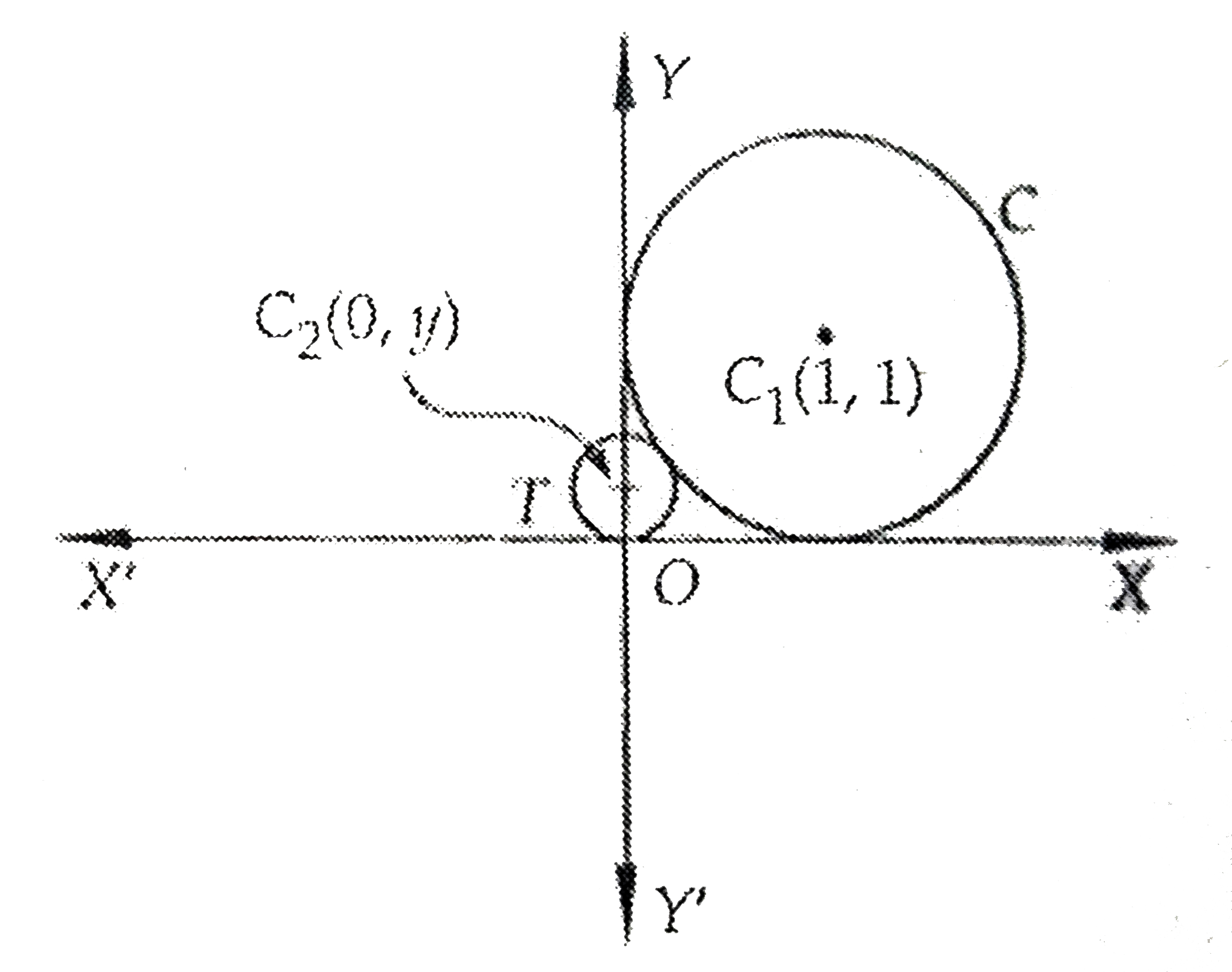
∴C1T= Sum of the radii
⇒√(0−1)2+(y−1)2=1+|y|[∵Radius of C and T are1 and |y|respectively]
⇒y2+2y+2=(1+|y|)2
⇒y2−2y+2=1+2|y|+y2
⇒2|y|+2y=1⇒4y=1⇒y=14
Topper's Solved these Questions
- CIRCLESBOOK - OBJECTIVE RD SHARMACHAPTER - CIRCLESEXERCISE - Section I - Solved Mcqs
108 Videos
- CIRCLESBOOK - OBJECTIVE RD SHARMACHAPTER - CIRCLESEXERCISE - Section-I (Solved MCQs)
1 Videos
- CARTESIAN PRODUCT OF SETS AND RELATIONS BOOK - OBJECTIVE RD SHARMACHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Chapter Test
31 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Chapter Test
59 Videos
Similar Questions
Let C be the circle with centre at (1, 1) and radius 1 If Tis the circle centred at (0, y), passing through origin and touching the circle C externally, then the radius of T is equal to V3 (a) 4
View SolutionLet C be the circle with centre at (1, 1) and radius = 1. If T is the circle centred at (0, y), passing through origin and touching the circle C externally, then the radius of T is equal to (1) √3√2 (2) √32 (3) 12 (3) 14
View Solution
Knowledge Check
Let C be the circle with centre (1,1) and radius 1 . If T is the circle centred at (0,y) , passing through origin and touching the circle C externally , then the radius of T is equal to
A12B14C√3√2D√32Equation of circle centred at (2,-3) , and passing through (-1,2) , is
Ax2+y2−4x−6y−34=0BX2+y2+4x−6y+34=0CX2+y2+4x+6y+21=0DX2+y2−4x+6y−21=0Equation of circle centred at (1,-1) , and passing through (3,2) , is
Ax2+y2−2x−2y−11=0Bx2+y2−2x+2y−11=0Cx2+y2+2x+2y−11=0Dx2+y2−2x+2y+11=0
Let C be the circle with centre (1,1) and radius 1.If T is the circle centered at (0,k) passing through origin and touching the circle C externally then the radius of T is equal to (a) √3√2 (b) √32 (c) 12 (d) 14
View Solution1) 1/5 2) 10 3) 10v2 4) 5v2 Let C be the circle with centre at (1,1) and radius -1. If T is the circle centered at (0,y), passing through origin and touching the circle C externally, then the radius of T is equal to 56. 3 2 1) 2) 3) 4)
View SolutionFind the equation of the circle with centre(-1,-3) and radius √3
View SolutionFind the equation of the circle with : Centre(1,1)andradius√2.
View SolutionIf the radisu of the circle passing through the origin and touching the line x+y=2 at (1,1) is r units, then the value of 3√2r is
View Solution
