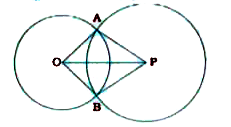
The line of centres of two intersecting circles subtends equal angles at the two points of intersection.
Not necessarily
Always true
Can not say
Not possible
The correct Answer is:b
Similar Questions
If two equal chords of a circle intersect within the circle, prove that the line joining the point of intersection to the centre makes equal angles with the chords.
View SolutionProve that the line of centres of two intersecting circles subtends equal angles at the two points of intersection
View SolutionProve that the line joining centres of two interesting circles subtends equal angles at the two points of intersection.
View SolutionIf two equal chords of a circle intersect within the circle, prove that the line joining the point of intersection to the centre makes equal angles with the chords.
View SolutionProve that the line segment joining the centres of two intersecting circles subtends equal angles at the two points of intersection.
View SolutionIf two equal chords of a circle intersect within the circle, prove that the line joining the point of intersection to the centre makes equal angles with the chord.
View SolutionA circle (with centre at O) is touching two intersecting lines AX and BY. The two points of contact A and B subtend an angle of 65∘ at any point C on the circumference of the circle. If P is the point of intersection for the two lines, then the measure of ∠APO is :
View Solution
