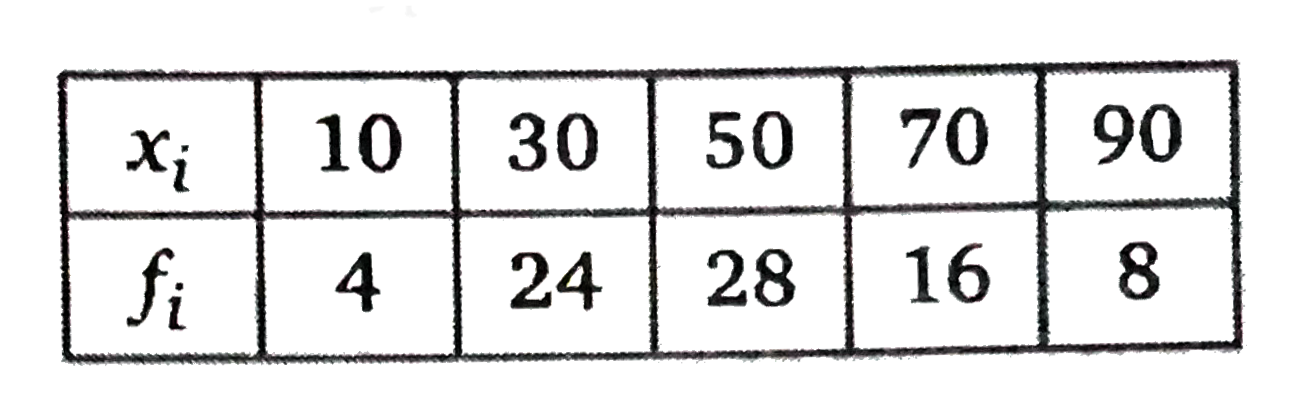
Find the mean deviation about the mean for the data :xi 10 30 50 70 90fi 4 24 28 16 8
To find the mean deviation about the mean for the given data, we will follow these steps:
Step 1: Calculate the Mean (x̄)
We will use the formula for the mean:
¯x=∑(xi⋅fi)∑fi
Where:
- xi are the data points
- fi are the frequencies
Given data:
- xi=(10,30,50,70,90)
- fi=(4,24,28,16,8)
First, we calculate ∑fi:
∑fi=4+24+28+16+8=80
Next, we calculate ∑(xi⋅fi):
10⋅4=4030⋅24=72050⋅28=140070⋅16=112090⋅8=720
Now, summing these products:
∑(xi⋅fi)=40+720+1400+1120+720=4000
Now we can find the mean:
¯x=400080=50
Step 2: Calculate the Absolute Deviations
Next, we find the absolute deviations from the mean:
|xi−¯x|
Calculating these:
|10−50|=40|30−50|=20|50−50|=0|70−50|=20|90−50|=40
Step 3: Multiply Absolute Deviations by Frequencies
Now we multiply each absolute deviation by its corresponding frequency:
fi⋅|xi−¯x|
Calculating these:
4⋅40=16024⋅20=48028⋅0=016⋅20=3208⋅40=320
Step 4: Sum the Products
Now we sum these products:
∑(fi⋅|xi−¯x|)=160+480+0+320+320=1280
Step 5: Calculate the Mean Deviation
Finally, we calculate the mean deviation using the formula:
Mean Deviation=∑(fi⋅|xi−¯x|)∑fi
Substituting the values we have:
Mean Deviation=128080=16
Conclusion
The mean deviation about the mean for the given data is 16.
---
- xi are the data points
- fi are the frequencies
- xi=(10,30,50,70,90)
- fi=(4,24,28,16,8)
Topper's Solved these Questions
- STATISTICSBOOK - NCERT ENGLISHCHAPTER - STATISTICSEXERCISE - EXERCISE 15.2
10 Videos
- STATISTICSBOOK - NCERT ENGLISHCHAPTER - STATISTICSEXERCISE - EXERCISE 15.3
5 Videos
- SETSBOOK - NCERT ENGLISHCHAPTER - SETSEXERCISE - EXERCISE 1.5
7 Videos
- STRAIGHT LINESBOOK - NCERT ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE 10.4
4 Videos
Similar Questions
Find the mean deviation about the mean for the data :xi 5 10 15 20 25fi 7 4 6 3 5
View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about the mean for the data is Question:
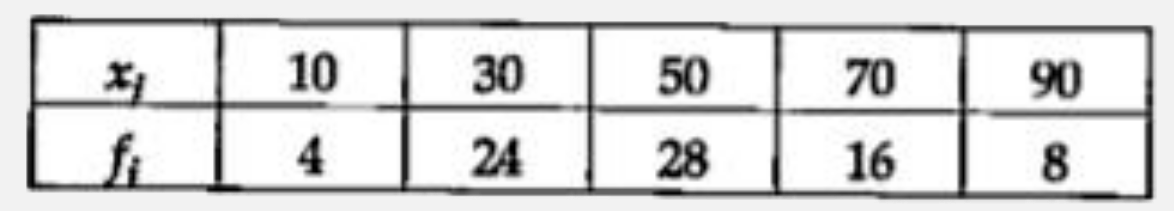
View SolutionFind the mean deviation about the mean for the data in Question:

View SolutionFind the mean deviation about the mean for the data in Question:

View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about mean for the following data:
View SolutionFind the mean deviation about the median for the data in Question

View SolutionFind the mean deviation about the mean for the following data.
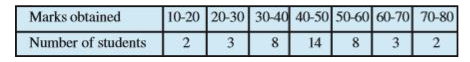
View Solution
