A rectangular sheet of tin 45 cm by 24 cm is to be made into a box without top by cutting off squares from the corners and folding up the flaps. What should be the side of the square in order the volume of the box is maximum.
x=18
x=24
x=5
None of these
The correct Answer is:C
Let the side of square be x
Then the remaining dimensions of cuboid for volume is
Length = 45−2x
width=24−2x
height =x
volume(v)=(45−2x)×(24−2x)×(x)
dvdx=(45−2x)×(24−2x)−2x(24−2x)−2x(45−2x)
dvdx=12(x2−23x+90)=12(x−5)(x−18)
Now dvdx=0atx=5,18
Now d2vdx=12(2x−23) at x=5 d2vdx<0
∴atx=5
i.e., side of square for which we will get maximum volume.
Topper's Solved these Questions
- APPLICATION OF DERIVATIVESBook:NCERT ENGLISHChapter:APPLICATION OF DERIVATIVESExercise:EXERCISE 6.1
Explore 18 Videos
- APPLICATION OF DERIVATIVESBook:NCERT ENGLISHChapter:APPLICATION OF DERIVATIVESExercise:EXERCISE 6.2
Explore 19 Videos
- APPLICATION OF DERIVATIVESBook:NCERT ENGLISHChapter:APPLICATION OF DERIVATIVESExercise:EXERCISE 6.4
Explore 9 Videos
- APPLICATION OF INTEGRALSBook:NCERT ENGLISHChapter:APPLICATION OF INTEGRALSExercise:EXERCISE 8.2
Explore 7 Videos
Similar Questions
A square piece of tin of side 18 cm is to be made into a box without top by cutting a square from each corner and folding up the flaps to form a box. What should be the side of the square to be cut off so that the volume of the box is maximum? Also, find the maximum volume.
View SolutionA rectangular sheet of tin 58 cm×44cm is to be made into an open box by cutting off equal squares from the corners and folding up the flaps.What should be the volume of box if the surface area of box is 2452 cm2?
View Solution
Knowledge Check
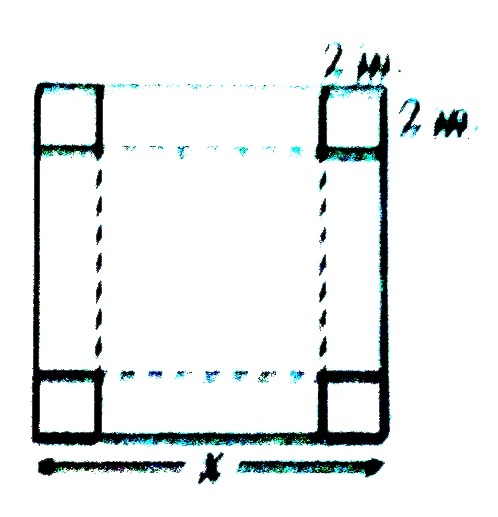
A square piece of cardbroad measuring x inches by x inches is to be used to form an open box by cutting off 2-inch squares, and then folding the slides up along the dotted lines, as shown in the figure above.
If x is an integer , and the volume of the box must be greater than 128 cubic inches , what is the smallest value of x that can be used ?A6B8C10D13
A square piece of tin of side 18 cm is to be made into a box without top, by cutting a square from each corner and folding up the flaps to form the box. What should be the side of the square to be cut off so that the volume of the box is the maximum possible?
View SolutionA box, constructed from a rectangular metal sheet, is 21 cm by 16cm by cutting equal squares of sides x from the corners of the sheet and then turning up the projected portions. The value of x os that volume of the box is maximum is (a)1 (b) 2 (c) 3 (d)4
View SolutionA box of maximum volume with top open is to be made by cutting out four equal squares from four corners of a square tin sheet of side length a feet and then folding up the flaps. Find the side of the square cut-off.
View SolutionFrom a rectangular sheet of tin, of size 100 cm by 80 cm, are cut four squares of side 10 cm from each corner. Find the area of the remaining sheet.
View SolutionAn open topped box is to be constructed by removing equal squares from each corner of a 3 metre by 8 metre rectangular sheet of aluminium and folding up the sides. Find the volume of the largest such box.
View SolutionA rectangular cardboard sheet has length 32 cm and breadth 26 cm. The four squares each of side 3 cm are cut from the corners of the sheet and the sides are folded to make a rectanguler container. Find the capacity of the container.
View SolutionA metallic sheet is of the rectangular shape with dimensions 48cm x 36cm. From each one of its corners, a square of 8cm is cut off. An open box is made of the remaining sheet. Find the volume of the box.
View Solution
