(Transportation problem) There are two factories located one at place P and the other at place Q. From these locations, a certain commodity is to be delivered to each of the three depots situated at A, B and C. The weekly requirements of the depots are respectively 5, 5 and 4 units of the commodity while the production capacity of the factories at P and Q are respectively 8 and 6 units. The cost of transportation per unit is given below : How many units should be transported from each factory to each depot in order that the transportation cost is minimum. What will be the minimum transportation cost?
The problem can be explained diagrammatically as follows (fig 1:)
Let x units and y units of the commodity be transported from the factory at P to the depots at A and B respectively. Then (8 –x–y) units will be transported to depot at C
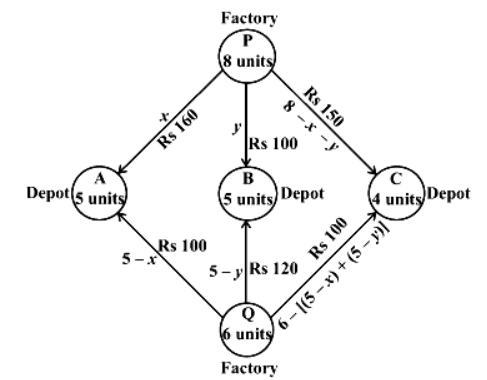
Hence we have
and
Total transportation cost Z is given by
Z = 160x+ 100y+ 100 ( 5 –x) + 120 (5 –y) + 100 (x+y– 4) + 150 (8 –x–y)= 10 (x– 7y+ 190)
Therefore, the problem reduces to
Minimize Z = 10 (x– 7y+ 190)
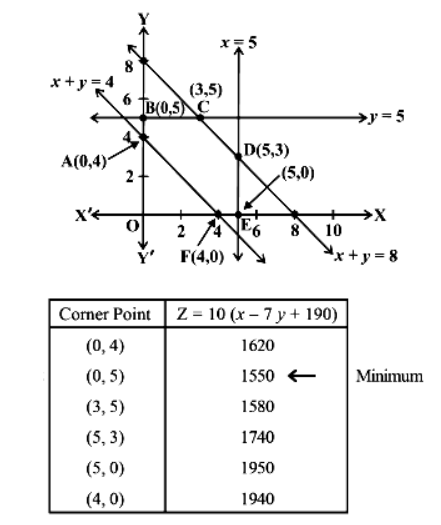
From the table, we see that the minimum value of Z is 1550 at the point (0, 5).
Hence, the optimal transportation strategy will be to deliver 0, 5 and 3 units from the factory at P and 5, 0 and 1 units from the factory at Q to the depots at A, B and C respectively. Corresponding to this strategy, the transportation cost would be minimum., Rs 1550
Topper's Solved these Questions
- LINEAR PROGRAMMINGBOOK - NCERT ENGLISHCHAPTER - LINEAR PROGRAMMINGEXERCISE - EXERCISE 12.2
11 Videos
- LINEAR PROGRAMMINGBOOK - NCERT ENGLISHCHAPTER - LINEAR PROGRAMMINGEXERCISE - MISCELLANEOUS EXERCISE
10 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - NCERT ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Solved Examples
13 Videos
- MATRICESBOOK - NCERT ENGLISHCHAPTER - MATRICESEXERCISE - All Questions
105 Videos
Similar Questions
There are two factories located one at place P and the other at place Q.From these locations, a certain commodity is to be delivered to each of the three depots situated at A, B and C. The weekly requirments of the depots are respectively 5,5 and 4 units of the commodity while the production capacity of the factories at P and Q are respectively 8 and 6 units. The cost of transportation per unit is given below:
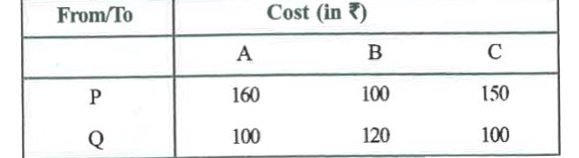
How many units should be transported from each factory to each depot in order that the transportable cost is minimum? When will be the minimum transportation cost?
View SolutionThere are factories located one at place P and other at place Q from which a certin commodity is to be delivered to each of three depot situated at A,B and C. The weekly requirement of the depots are respectively 5,5 and 4 units of the commodity while the production capacity of the factories P and Q are respectively 8 and 6 units. The cost of transportation per unit is given below.
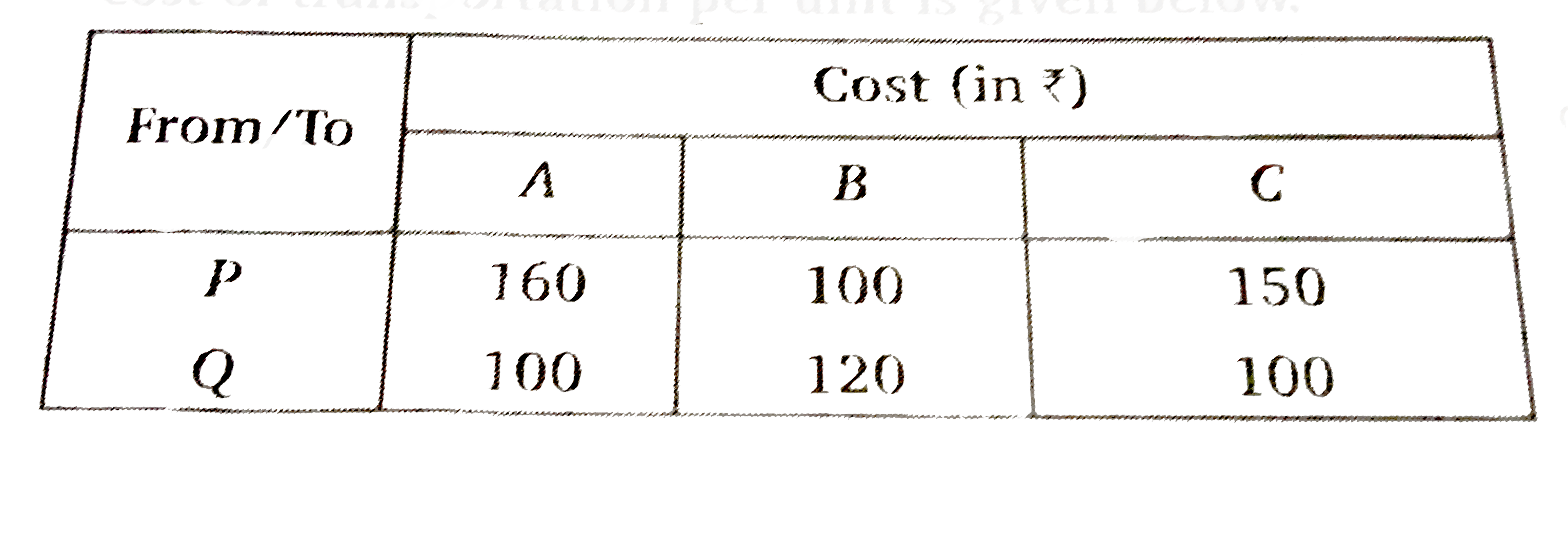
How many units should be transported from each factory to each depot in order that the transportation cost is minimum. Also find the minimum transportation cost.
View Solution
Knowledge Check
Maximum number of sugar factories are located in
AUttar PradeshBTamilnaduCBiharDAssamThe ratio of the production of scooters by factoryP to that by factory T in 1985 is
A2:3B1 : 2C3:2D2:1
A medical company has factories at two places A and B. From these places supply is made to each of its three agencies situated at P, Q and R. The monthly requirements of the agen cies are respectively 40,40 and 50 packets of the medicines, while the production capacity of the factories A and B are 60 and 70 packets respectively. The transportation costs per packet from the factories to the agencies are given below:

View SolutionA medical company has factories at two places, A and B.From these places, supply is made to each of its three agencies situated at P,Q, and R.The monthly requirements of the agencies are respectively 40,40 and 50 packets of the medicines, while the production capacity of the factories A and B, are 60 and 60 packets respectively.The transportation cost per packet from the factories to the agencies are given below:

How many packets from each factory be transported to each agency so that the cost of transportation is minimum? Also find the minimum cost.
View Solution(Transportation Problem) A catering agency has two kitchens to prepare food at two places A and B. From these places 'Mid-day Meal' is to be supplied to threee different schools situated at P,Q,R. The monthly requirments of the schools are respectively 40,40 and 50 food packets.A packet contains lunch for 1000 students.Preparing capacity of kitchen A and B are 60 and 70 packets per month respectively.The transpportation cost per packet for the kitchen to schools s given below:
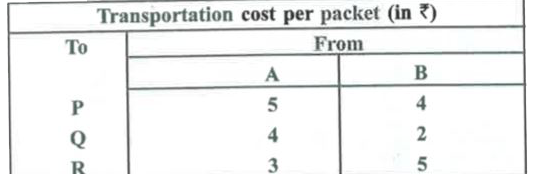
How many packets from kitechen should be transported to schools so that the cost of transportation is minimum? Also find the minimum cost.
View SolutionA brick manufacturer has two depots A and B with stocks of 30000 and 20000 bricks respectively. He receives orders from three builders P, Q and R for 15000, 20000 and 15000 bricks respectively. The cost of transporting 1000 bricks to the builders from the depots (in rupees) are given below:
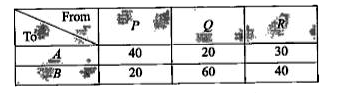
How should the manufacturer fulfil the order so as to keep the cost of transportation minimum? What is the minimum cost?
View SolutionAn oil company has two depots A and B with capacities of 7000 L and 4000 L respectively. The company is to supply oil to three petrol pumps, D, E and F whose requirements are 4500L, 3000L and 3500L respectively. The distances (in km) between the depots and the petrol pumps is given in the following table:
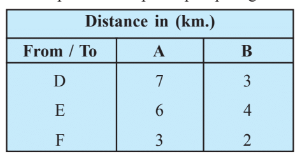
Assuming that the transportation cost of 10 litres of oil is Re 1 per km, how should the delivery be scheduled in order that the transportation cost is minimum? What is the minimum cost?
View SolutionAn oil company has two depots A and B with capacities of 7000L and 4000L respectively. The company is to supply oil to three petrol pumps, D,E and F whose requirements are 4500L,3000L and 3500L respectively. The distances (in km) between the depots and the petrol pumpa is given in the following table:

Assuming that the transportation cost of 10 litres of oil is Rs. 1 per km, how shold the delivery be scheduled in order that the transportation cost is minimum? What is the minimum cost?
View Solution
