A class has 15 students whose ages are 14, 17, 15, 14, 21, 17, 19, 20, 16, 18, 20, 17, 16, 19 and 20 years. One student is selected in such a manner that each has the same chance of being chosen and the age X of the selected student is recorded.
Below mentioned is the table of P(X)
By using above data the we can find the mean and mean, variance and standard deviation of X. 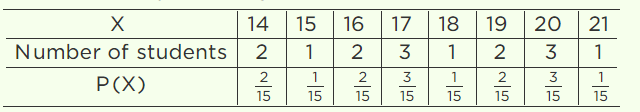
Mean X = E(X)=∑XiP(Xi)=
=14×215+15×115+16×215+17×315+18×115+19×215+20×315+21×115
by solving we will get 26315=17.53
E(X2)=∑(Xi)2P(Xi)
=142×215+152×115+162×215+172×315+182×115+192×215+202×315+212×115
by solving we will get 468315=312.2
Variance(X)=E(X2)−(E(X))2
312.2−(26315)2=4.78
Standard Variance=√Variance=√4.78=2.186
Similar Questions
The mode of given data 15, 14, 19, 20, 14, 15, 16, 14, 15, 18, 14, 19, 15, 17 and 15 is
View SolutionFor the data 14,7,13,12,13,17,8,10,6,15,18,21,20, compute P80
View SolutionFind the median of :
25, 18, 13, 20, 16, 9, 22, 8, 6, 15, 21, 11, 17
View SolutionThe median of 25, 14, 11, 26, 18 , 17, 40, 29, 19, 20, 13 is
View SolutionThe range of the data 25, 18, 20, 22, 16, 6, 17, 15, 12, 30, 32, 10, 19, 8, 11 and 20 is
View SolutionA class has 20 girls and 16 boys. One student is selected at random. Find the probability that the student is a girl.
View SolutionThe ages of 15 students in years) in a summer camp are as follows: 11, 14, 13, 15, 16, 10, 12, 12, 14, 11, 10, 12, 13, 15, 11 Find: The mean age.
View SolutionThe ages of 15 students (in years) in a summer camp are as follows: 11, 14, 13, 15, 16, 10, 12, 12, 14, 11, 10, 12, 13, 15, 11 Find:
the minimum age.
View SolutionThe marks of 19 students in a test were as follows: 5, 6, 8, 9, 10, 11, 12, 13, 13, 14, 14, 15, 15, 15, 16, 16, 18, 19, 20. Calculate the median and mode.
View SolutionThe ages of 15 students (in years) in a summer camp are as follows: 11, 14, 13, 15, 16, 10, 12, 12, 14, 11, 10, 12, 13, 15, 11 Find:
the maximum age.
View Solution
