In one fortnight of a given month, there was a rainfall of 10 cm in a river valley. If the area of the valley is , show that the total rainfall was approximately equivalent to the addition to the normal water of three rivers each 1072 km long,75 m wide and 3m deep
To solve the problem step by step, we will calculate the total rainfall in the river valley and compare it to the volume of water in three rivers.
Step 1: Calculate the volume of rainfall in the valley
The volume of rainfall can be calculated using the formula:
Volume=Area×Height
Given:
- Area of the valley = 97280km2
- Height of rainfall = 10cm=0.0001km (since 1cm=10−5km)
Now, substituting the values:
Volume=97280km2×0.0001km
Volume=9.728km3
Step 2: Calculate the volume of rainfall over 14 days
Since the rainfall volume calculated is for the entire fortnight (14 days), we can use this value directly.
Step 3: Calculate the volume of one river
The volume of one river can be calculated using the formula:
Volume=Length×Breadth×Height
Given:
- Length of the river = 1072km
- Width of the river = 75m=0.075km (since 1m=0.001km)
- Depth of the river = 3m=0.003km
Now, substituting the values:
Volume of one river=1072km×0.075km×0.003km
Volume of one river=0.2412km3
Step 4: Calculate the total volume of three rivers
Now, we multiply the volume of one river by 3:
Total volume of three rivers=3×0.2412km3=0.7236km3
Step 5: Compare the volumes
Now we compare the total rainfall volume with the total volume of three rivers:
- Volume of rainfall in the valley = 9.728km3
- Total volume of three rivers = 0.7236km3
Conclusion
Since 9.728km3 (rainfall) is approximately equivalent to 0.7236km3 (volume of three rivers), we can conclude that the total rainfall was approximately equivalent to the addition to the normal water of three rivers.
The volume of rainfall can be calculated using the formula:
Volume=Area×Height
- Area of the valley = 97280km2
- Height of rainfall = 10cm=0.0001km (since 1cm=10−5km)
Volume=97280km2×0.0001km
Volume=9.728km3
Since the rainfall volume calculated is for the entire fortnight (14 days), we can use this value directly.
The volume of one river can be calculated using the formula:
Volume=Length×Breadth×Height
- Length of the river = 1072km
- Width of the river = 75m=0.075km (since 1m=0.001km)
- Depth of the river = 3m=0.003km
Volume of one river=1072km×0.075km×0.003km
Volume of one river=0.2412km3
Now, we multiply the volume of one river by 3:
Total volume of three rivers=3×0.2412km3=0.7236km3
Now we compare the total rainfall volume with the total volume of three rivers:
- Volume of rainfall in the valley = 9.728km3
- Total volume of three rivers = 0.7236km3
Since 9.728km3 (rainfall) is approximately equivalent to 0.7236km3 (volume of three rivers), we can conclude that the total rainfall was approximately equivalent to the addition to the normal water of three rivers.
Similar Questions
In one fortnight of a given month, there was a rainfall of 10cm in a river valley. If the area of the valley is 7280km2. show that the total rainfall was approximately equivalent to the addition to the normal water of three rivers each 1072 km long, 75 m wide and 3 m deep.
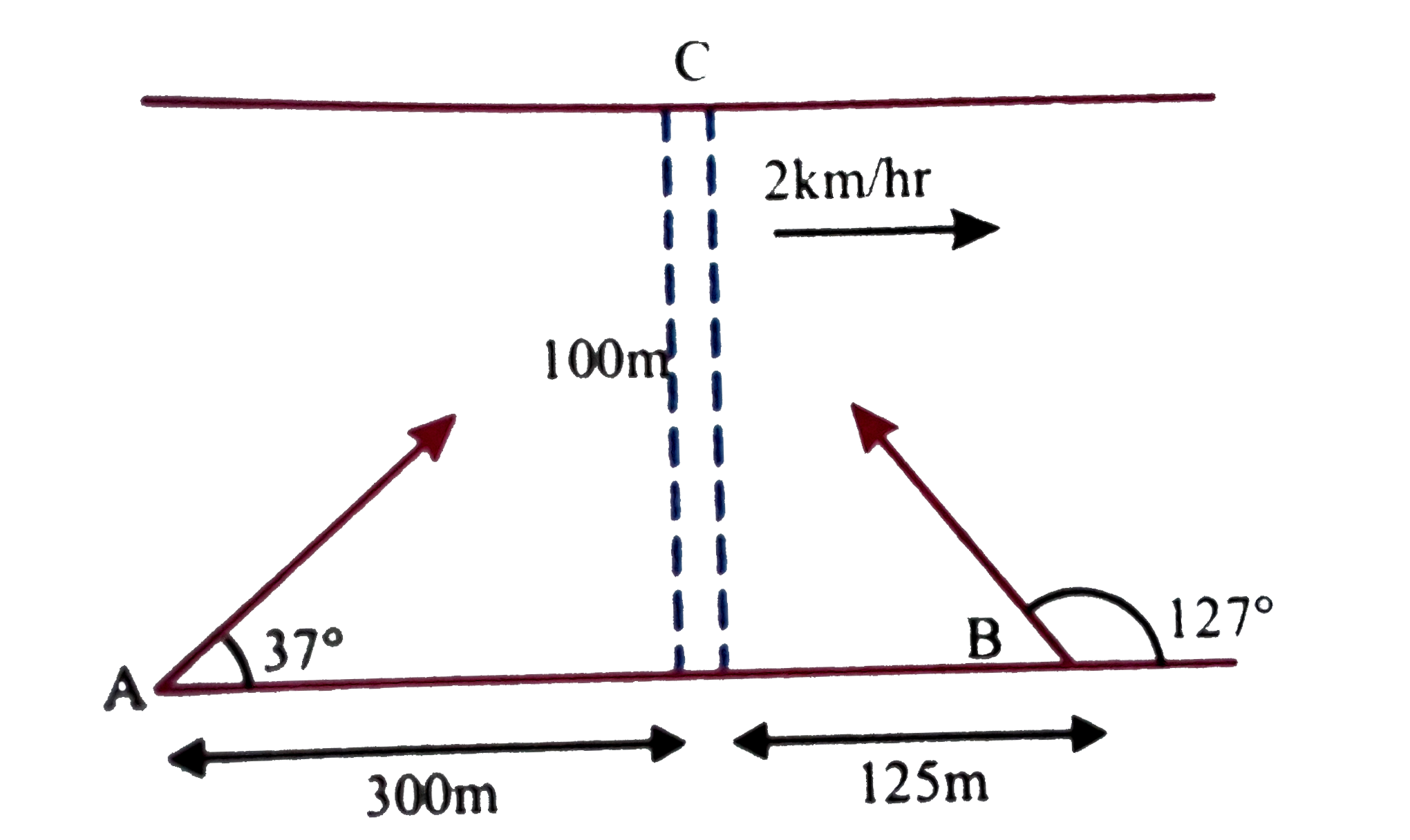
View SolutionTwo swimmers start a race. One who reaches the point C first on the other bank wins the race.A makes his strokes in a direction of 370 to the river flow with velocity 5km/hr relative to water. B makes his strokes in a direction 1270 to the river flow with same relative velocity.River is flowing with speed of 2km/hr and is 100m wide.speeds of A and B on the ground are 8km/hr and 6km/hr respectively.
View SolutionA poster of size 10 cm by 8 cm is pasted on a sheet of cardboard such that there is a margin of width 1.75 cm along each side of the poster. Find (a) The total area of the margin (b) The cost of the cardboard used at the rate of Re 0.60 per cm2
View SolutionTwo metal plates of area 2×10−4m2 each, are kept in water and one plate is moved over the other with a certain velocity. The distance between the plates is 2×10−4m. If the horizontal force applied to move the plate is 10−3N, calculate the velocity of the plate. Given η of water is10−3 decapoise.
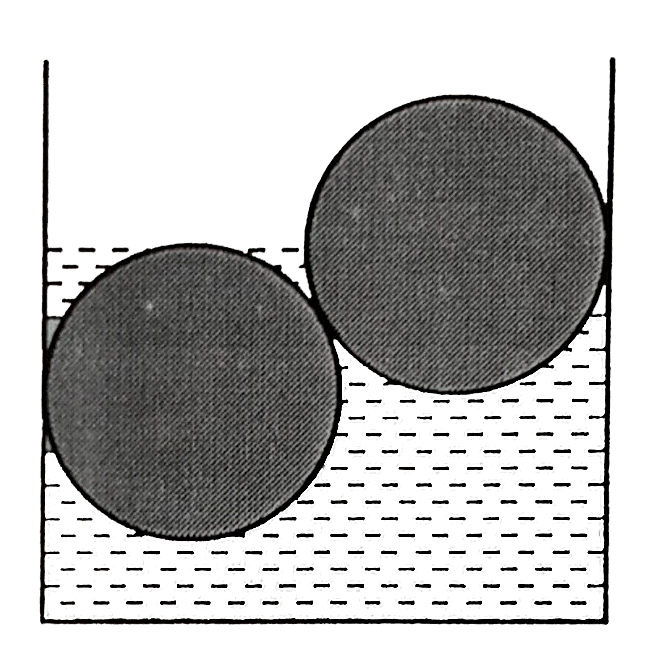
View SolutionThere are two cylinder shaped wooden billets, each having a mass of mass 45kg, in a vertical wall sewage, which contains water in it. the two billets have the same size and the same material, they touch each other and the walls of the sewage. One of them is totally under the water, whilst only half of the other one is immersed into the water. Friction is negligible everywhere. (density of water is 1000kg/m3 and g=10m/s2). Choose the correct option(s)
View SolutionWhen a liquid flows in a tube, there is relative motion between adjacent layers of the liquid. This force is called the viscous force which tends to oppose the relative motion between the layers of the liquid. Newton was the first person to study the factors that govern the viscous force in a liquid. According to Newton’s law of viscous flow, the magnitude of the viscous force on a certain layer of a liquid is given by
F=−ηAdvdx
where A is the area of the layer dvdx is the velocity gradient at the layer and η is the coefficient of viscosity of the liquid.
A river is 5 m deep. The velocity of water on its surface is 2ms−1 If the coefficient of viscosity of water is 10−3Nsm−2 , the viscous force per unit area is :
View SolutionA long straight copper wire, of circular cross ection, contains n conduction electrons per unit volume, each of charge q. Show that the current l in the wire is given by
l=nqvπa2
where v is the drift velocity and a is the radius of the wire.
At a radial distance r from the axis of the wire, what is the direction of the magnetic field B due to the current l? Assuming that the magnitude of the field is B=μ0l/2πr(r≥a), obtain an expression for the Lorentz force F on an electron moving with the drift velocity at the surface of the wire.
If l=10 A and a = 0.5mm, calcualte the magnitude of (a) the drift velocity and (b) the forcce, given that for copper, n=8.5×1028m−3
View SolutionConsider a point charge q=1mC placed at a corner of a cube of sides 10 cm . Determine the electric flux through each face of the cube.
Strategy : We will learn about the utility of symmetry in solving problems with the help of Gauss's law . Here we'll use the symmetry of the situation,which involves the faces joining at the corner at which the charge resides.

(a) A charge q is placed atthe corner of a cube. (b) By surrounding the charge with a series of cubes such that the charge is at the centre of a larger cube, we have created an arrangement sufficiently symmetric to be able to solve for desired flux values.
You can see from figure that for these faces →E.ˆn=0. Since the normal is perpendicular to the surfaces while the electric field goes off in a spherically symmetric pattern and lies in the sides . In other words , the electric field that originates at the charge is tangential to the surface of these three sides. This means there is no flux through these sides. The electric flux through each of the remaining three faces of the be must be equal by symmetry . We'll referto these faces with the label F.
To fidn the flux through each of the sides F, we acan use a technique that puts the single charge in the middle of a larger cube. It takes seven other similarly placed cubes to surrounds the points charge q completely in figre. The charge is at the dead centre of the enw larger cube. So, the flux through each of the six sides of the large cube will now have an electric flux of one sixth of the total flux F. So given that the total structure is completely symmetric, the flux through a side F is one fourth of the flux through the larger side.
View SolutionA river 3 m deep and 40 m wide is flowing at the rate of 2 km per hour. How much water will fall into the sea in a minute?
View SolutionA large wooden plate of area 10m2 floating on the surface of river is made to move horizontally wilth a speed of 2ms−1 by applying a tangential force. If the river is 1m deep and the water contact with the bed is stationary, find the tangential force needed to keep the plate moving. Coefficient of viscosity of water at the temperature of the river =10−2poise.
View Solution
