The heights of 50 students, measured to the nearest centimetres, have been found to be as follows: 161 150 154 165 168 161 154 162 150 151162 164 171 165 158 154 156 172 160 170153 159 161 170 162 165 166 168 165 164154 152 153 156 158 162 160 161 173 166161 159 162 167 168 159 158 153 154 159 (i) Represent the data given above by a grouped frequency distribution table, taking the class intervals as 160 - 165, 165 - 170, etc.(ii) What can you conclude about their heights from the table?
To solve the problem step by step, we will first create a grouped frequency distribution table for the heights of the 50 students.
Step 1: Organize the Data
We have the heights of 50 students. The heights range from 150 cm to 173 cm. We will create class intervals of 5 cm each, starting from 150 cm.
Step 2: Define Class Intervals
The class intervals will be:
- 150 - 155
- 155 - 160
- 160 - 165
- 165 - 170
- 170 - 175
Step 3: Tally the Frequencies
Now, we will count how many students fall into each class interval.
1. 150 - 155: Count the heights in this range.
- Heights: 150, 150, 154, 154, 154, 154, 152, 153, 153, 153
- Frequency: 10
2. 155 - 160: Count the heights in this range.
- Heights: 158, 158, 156, 156, 159, 159
- Frequency: 6
3. 160 - 165: Count the heights in this range.
- Heights: 161, 161, 161, 162, 162, 162, 164, 164, 165, 165, 165, 165
- Frequency: 12
4. 165 - 170: Count the heights in this range.
- Heights: 168, 168, 167, 170, 170, 170
- Frequency: 6
5. 170 - 175: Count the heights in this range.
- Heights: 171, 172, 173
- Frequency: 3
Step 4: Create the Frequency Distribution Table
Now we can summarize the data in a table format:
| Class Interval | Frequency |
|----------------|-----------|
| 150 - 155 | 10 |
| 155 - 160 | 6 |
| 160 - 165 | 12 |
| 165 - 170 | 6 |
| 170 - 175 | 3 |
| Total | 50 |
Step 5: Analyze the Data
Now we can conclude about the heights of the students based on the frequency distribution table.
- Heights less than 165 cm:
- 150 - 155: 10
- 155 - 160: 6
- 160 - 165: 12
- Total = 10 + 6 + 12 = 28 students
- Heights greater than or equal to 165 cm:
- 165 - 170: 6
- 170 - 175: 3
- Total = 6 + 3 = 9 students
Conclusion
From the table, we can conclude that:
- A majority of the students (28 out of 50) have heights less than 165 cm.
- Only 9 students have heights greater than or equal to 165 cm.
We have the heights of 50 students. The heights range from 150 cm to 173 cm. We will create class intervals of 5 cm each, starting from 150 cm.
The class intervals will be:
- 150 - 155
- 155 - 160
- 160 - 165
- 165 - 170
- 170 - 175
Now, we will count how many students fall into each class interval.
- Heights: 150, 150, 154, 154, 154, 154, 152, 153, 153, 153
- Frequency: 10
- Heights: 158, 158, 156, 156, 159, 159
- Frequency: 6
- Heights: 161, 161, 161, 162, 162, 162, 164, 164, 165, 165, 165, 165
- Frequency: 12
- Heights: 168, 168, 167, 170, 170, 170
- Frequency: 6
- Heights: 171, 172, 173
- Frequency: 3
Now we can summarize the data in a table format:
|----------------|-----------|
| 150 - 155 | 10 |
| 155 - 160 | 6 |
| 160 - 165 | 12 |
| 165 - 170 | 6 |
| 170 - 175 | 3 |
| Total | 50 |
Now we can conclude about the heights of the students based on the frequency distribution table.
- 150 - 155: 10
- 155 - 160: 6
- 160 - 165: 12
- Total = 10 + 6 + 12 = 28 students
- 165 - 170: 6
- 170 - 175: 3
- Total = 6 + 3 = 9 students
From the table, we can conclude that:
- A majority of the students (28 out of 50) have heights less than 165 cm.
- Only 9 students have heights greater than or equal to 165 cm.
Topper's Solved these Questions
Similar Questions
The heights of 50 students, measured to the nearest centimetres have been found to be as follows:
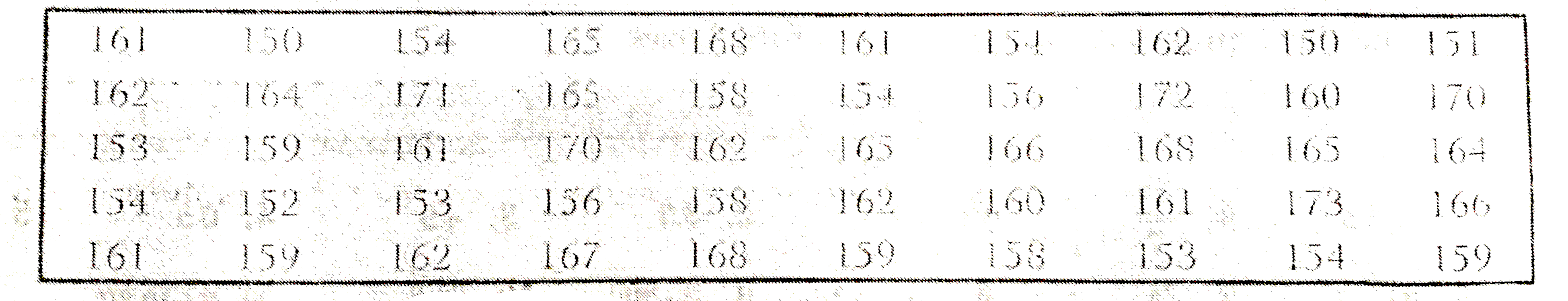
(i) Represent the data given above by a grouped frequency distribution table, taking class intervals as 160-165, 165-170 etc.
(ii) What can you conclude about their heights from the table ?
View SolutionThe heights, to the nearest cm, of 30 men are given below:
159170174173175160161164163165164171162170177185181180175165186174168168176176165175167180
Using class intervals 155 - 160, 160 - 165, ... draw up a grouped frequency distribution and use this to estimate the Arithmetic mean and standard deviation.
View SolutionThe values of measurement of a physical quantity in five trails were found to be 1.51,1.53,1.53,1.52,and1.54.
View SolutionHeights of 25 children (in cm) in a school are as given below:168, 165, 163, 160, 163, 161, 162, 164, 163, 162, 164, 163, 160, 163, 163, 164, 163, 160, 165, 163, 162 What is the mode of heights?
View SolutionThe median of 111, 129, 143, 118, 120, 125, 170, 162 is
View SolutionThe heights of 6 boys are 146 cm, 154 cm, 153 cm, 160 cm, 157 cm and 160 cm. Find their mean height.
View SolutionThe lengths of 40 leaves of a plant are measured correct to the nearest millimetre, and the data obtained is represented in the following table: Length (mm): 118-126 127-135 136-144 145-153 154-162 163-171 172-180 No. of leaves: 3 5 9 12 5 4 2. Find the mean length of leaf.
View SolutionFind the factors of
154
View SolutionEvaluate:
162.5÷5
View SolutionThe heights (in cm) of 30 students of class VIII are given below:
155,158,154,158,160,148,149,150,153,159,161,148,157,153,157,162,159,151,154,156,152,156,160,152,147,155,163,155,157,153
Prepare a frequency distribution table with 160−164 as one of the class intervals.
View Solution
