Doubtnut Promotions Sticky

Ratio of distance of the school from Mary’s home to the distanceof the school from John’s home is 2 : 1.(a) Who lives nearer to the school?(b) Complete the following table which shows some possible distances that Mary and John could live from the schoolIf the ratio of distance of Mary’s home to the distance of Kalam’s home from school is 1 : 2, then who lives nearer to the school?

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

a)John c)Mary

|
Updated on:21/07/2023
Class 6MATHSRATIO AND PROPORTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    The ratio of girls to boys at a certain school is 4:3. Which of the following could not be the number of students at the school ?

    A1430
    B1477
    C1547
    D2107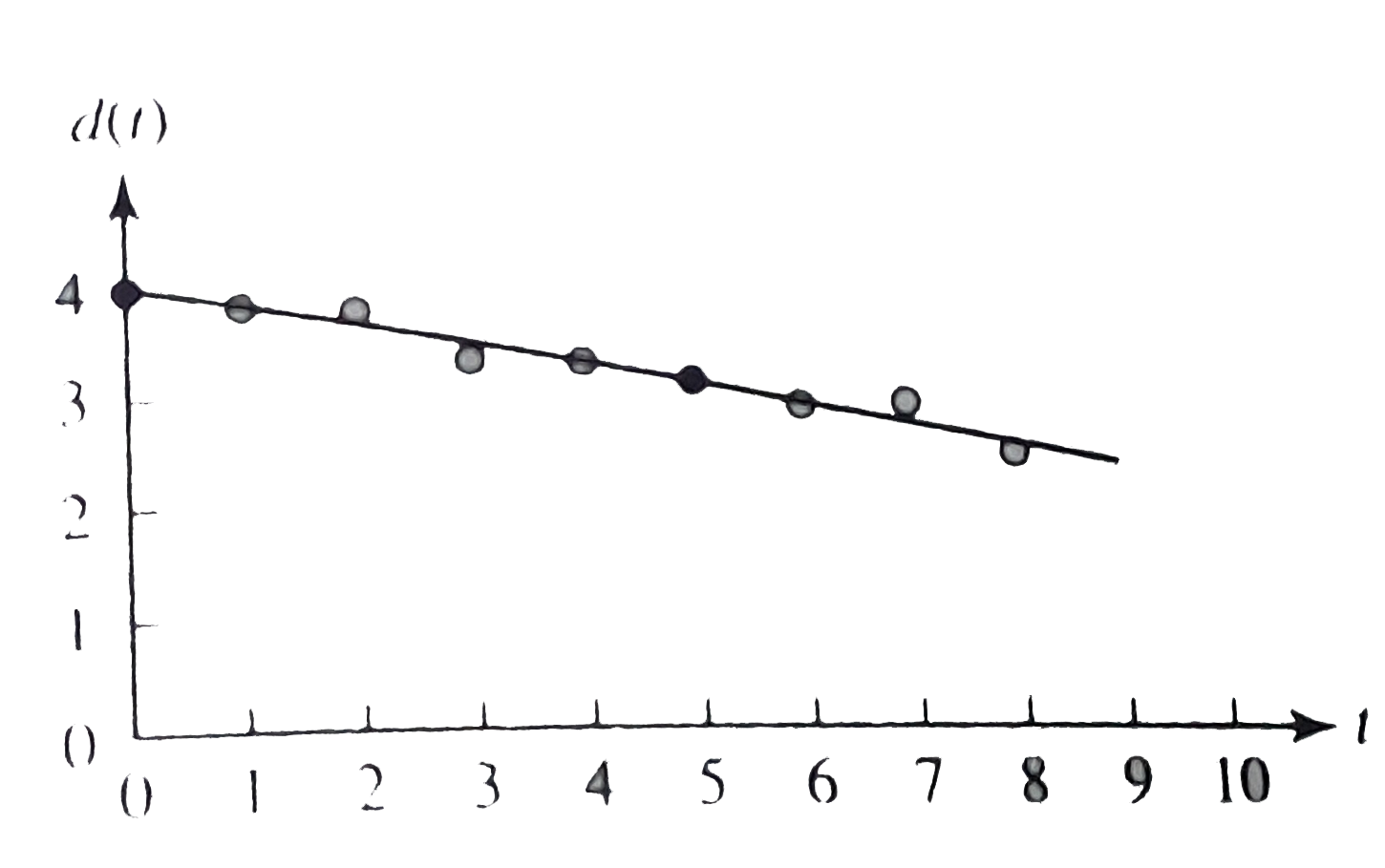
  • Question 2 - Select One

    ocr_image
    Joe bikes 4 kilometers to school . Because of the traffic and road conditions along the way , his speed varies. The dots on the graph above show his distance from the school at various times , starting at home at t=0 (black dot). After 5 minutes Joe is 3.2 kilometers from the school (second black dot). The data show that his speed is almost constant , and his distance from the school can be approximated by a straight line . The graph of the function that models Joe's distance from school as a function of time , in minutes , is shown as a solid line. Which of the following equations best represents this function ?

    Ad(t)=-0.5 t+4
    Bd(t)=-0.16 t +4
    Cd(t)=-6.25 t+4
    Dd(t)=0.16 t +4