A man wishes to estimate the distance of a nearby tower from him. He stands at a point A in front of the tower C and spots a very distant object O in line with AC. He then walks perpendicualr to AC upto B, a distaance of 100m and looks at O and C again. Since O is very distant, the direction of BO is practically the same as AO, but he finds the line of sight of C shifted from the original line of sight by an angle θ=40∘(θ is known as parallax). Estimate the distance fo the tower C from his original position A.
To estimate the distance of the tower C from the man's original position A, we can use the concept of parallax and trigonometry. Here’s a step-by-step solution:
Step 1: Understand the Geometry of the Situation
- The man stands at point A, looking at the tower C and a distant object O.
- He walks 100 meters perpendicular to the line AC to point B.
- The angle of parallax (the shift in the line of sight to the tower C) is given as θ = 40°.
Step 2: Set Up the Triangle
- In triangle ACB, we can identify:
- AB = 100 m (the distance the man walked)
- AC = H (the distance from A to the tower C, which we need to find)
- The angle ∠CBA = θ = 40°.
Step 3: Use Trigonometric Relationships
- We can use the tangent function, which relates the opposite side to the adjacent side in a right triangle:
tan(θ)=oppositeadjacent=ABAC
- Substituting the known values:
tan(40°)=100H
Step 4: Rearranging the Equation
- Rearranging the equation to solve for H:
H=100tan(40°)
Step 5: Calculate H
- Now we need to calculate the value of H using the tangent of 40°:
- First, find tan(40°). Using a calculator, we find:
tan(40°)≈0.8391
- Now substitute this value into the equation:
H=1000.8391≈119.2 m
Step 6: Conclusion
- Therefore, the estimated distance of the tower C from the man's original position A is approximately:
H≈119 m
- The man stands at point A, looking at the tower C and a distant object O.
- He walks 100 meters perpendicular to the line AC to point B.
- The angle of parallax (the shift in the line of sight to the tower C) is given as θ = 40°.
- In triangle ACB, we can identify:
- AB = 100 m (the distance the man walked)
- AC = H (the distance from A to the tower C, which we need to find)
- The angle ∠CBA = θ = 40°.
- We can use the tangent function, which relates the opposite side to the adjacent side in a right triangle:
tan(θ)=oppositeadjacent=ABAC
- Substituting the known values:
tan(40°)=100H
- Rearranging the equation to solve for H:
H=100tan(40°)
- Now we need to calculate the value of H using the tangent of 40°:
- First, find tan(40°). Using a calculator, we find:
tan(40°)≈0.8391
- Now substitute this value into the equation:
H=1000.8391≈119.2 m
- Therefore, the estimated distance of the tower C from the man's original position A is approximately:
H≈119 m
Topper's Solved these Questions
Similar Questions
How are we able to see nearby and also the distant objects clearly?
View SolutionThe angles of elevation of the top of a tower from two points at a distance of 4 m and 9 m from the base of the tower and in the same straight line with it are complementary. Prove that the height of the tower is 6m.
View SolutionThe angles of elevation of the top of a tower from two points at a distance of 4 m and 9 m from the base of the tower and in the same straight line with it are complementary. Prove that the height of the tower is 6 m.
View SolutionIf the angles of elevation of the top of a tower from two points at a distance of 4m and 9m from the base of the tower and in the same straight line with it are complementary, find the height of the tower.
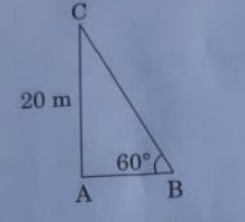
View SolutionIn the figure-2, the angle of elevation of the top of tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m. find the distance of the point from the foot of the tower.
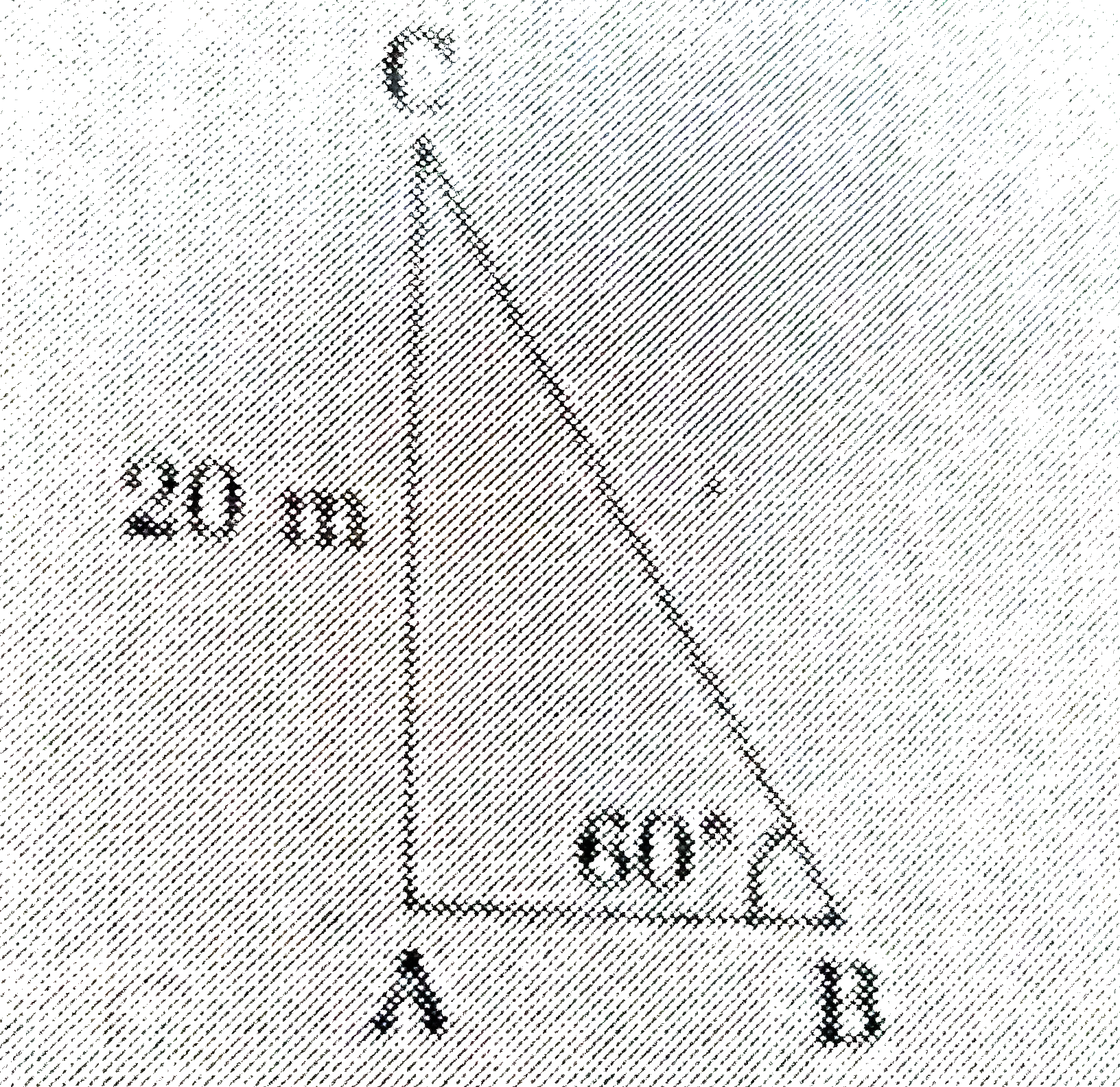
View SolutionThe angles of elevation of the top of a tower from two points at a distances a meter and b metres from the base of the tower and in the same straight line with it are complementary. Prove that the height of the tower is √ab metres.
View SolutionA man is standing at distance x from a plane mirror in front of him. He wants to see the entire wall in mirror which is at distance. y behind the man. Find the minimum size of the mirror required.
View SolutionA vertical tower is 20 m high. A man standing at some distance from the tower knows that the cosine of the angle of elevation of the top of the tower is 0.53. How far is he standing from the foot of the tower ?
View SolutionIn figure, the angle of elevation of the top of a tower AC from a point B on the ground is 60∘. If the height of the tower is 20m , find the distance of the point from the foot of the tower.
View SolutionIn figure, the angle of elevation of the top of a tower AC from a point B on the ground is 60∘. If the height of the tower is 20m , find the distance of the point from the foot of the tower.
View Solution