An insect trapped in a circular groove of radius 12 cm moves along the groove steadily and completes 7 revolutions in 100 s. (i) What is the angular speed and the linear speed of the motion ? (ii) Is the acceleration vector a constant vector ? What is its magnitude ?
To solve the problem step by step, we will break it down into parts as per the question.
Given Data:
- Radius of the circular groove, r=12cm=0.12m
- Number of revolutions, n=7
- Time taken, T=100s
Part (i): Finding Angular Speed and Linear Speed
1. Calculate the total angle in radians:
The total angle θ in radians for n revolutions is given by:
θ=n×2π=7×2π=14πradians
Hint: Remember that one complete revolution corresponds to 2π radians.
2. Calculate the angular speed ω:
Angular speed is defined as the total angle covered divided by the time taken:
ω=θT=14π100=0.14πradians/second
Hint: Angular speed is measured in radians per second, and you can find it by dividing the total angle by the time.
3. Calculate the linear speed v:
The linear speed is related to angular speed and radius by the formula:
v=ωr
Substituting the values:
v=(0.14π)×0.12=0.0168πm/s
Hint: Linear speed can be found by multiplying angular speed by the radius of the circular path.
Part (ii): Acceleration Vector
4. Determine if the acceleration vector is constant:
In circular motion, the acceleration can be divided into two components: tangential acceleration (At) and centripetal acceleration (Ac).
- Since the insect moves steadily, the tangential acceleration At=0 (no change in speed).
- The centripetal acceleration is given by:
Ac=ω2r
Hint: In uniform circular motion, the speed is constant, so the tangential acceleration is zero.
5. Calculate the magnitude of centripetal acceleration:
Substitute the values of ω and r:
Ac=(0.14π)2×0.12
First, calculate (0.14π)2:
(0.14π)2≈0.0196π2
Then multiply by 0.12:
Ac≈0.0196π2×0.12≈2.3×10−2m/s2
Hint: The magnitude of centripetal acceleration can be calculated using the square of angular speed multiplied by the radius.
6. Conclusion about the acceleration vector:
The acceleration vector is not constant because, although its magnitude remains the same, its direction changes continuously as the insect moves along the circular path.
Final Answers:
- Angular speed ω=0.14πradians/second
- Linear speed v=0.0168πm/s
- The acceleration vector is not constant, and its magnitude is approximately 2.3×10−2m/s2.
- Radius of the circular groove, r=12cm=0.12m
- Number of revolutions, n=7
- Time taken, T=100s
The total angle θ in radians for n revolutions is given by:
θ=n×2π=7×2π=14πradians
Angular speed is defined as the total angle covered divided by the time taken:
ω=θT=14π100=0.14πradians/second
The linear speed is related to angular speed and radius by the formula:
v=ωr
Substituting the values:
v=(0.14π)×0.12=0.0168πm/s
In circular motion, the acceleration can be divided into two components: tangential acceleration (At) and centripetal acceleration (Ac).
- Since the insect moves steadily, the tangential acceleration At=0 (no change in speed).
- The centripetal acceleration is given by:
Ac=ω2r
Substitute the values of ω and r:
Ac=(0.14π)2×0.12
First, calculate (0.14π)2:
(0.14π)2≈0.0196π2
Then multiply by 0.12:
Ac≈0.0196π2×0.12≈2.3×10−2m/s2
The acceleration vector is not constant because, although its magnitude remains the same, its direction changes continuously as the insect moves along the circular path.
- Angular speed ω=0.14πradians/second
- Linear speed v=0.0168πm/s
- The acceleration vector is not constant, and its magnitude is approximately 2.3×10−2m/s2.
Topper's Solved these Questions
- MOTION IN A PLANE BOOK - NCERT ENGLISHCHAPTER - MOTION IN A PLANE EXERCISE - EXERCISE
32 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - EXERCISE
21 Videos
- MOTION IN A STRAIGHT LINEBOOK - NCERT ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - EXERCISE
28 Videos
Similar Questions
An insect trapped in a circular groove of radius, 12 cm moves along the groove steadily and completes 7 revolutions in 100s. What is the angular speed and the linear speed of the motion ?
View SolutionAn insect trapped in a circular groove of radius 12 cm moves along the groove steadily and completes 7 revolutions in 100s.What is the magnitude of the centripetal acceleration.
View Solution
Knowledge Check
An insect trapped in circular groove of radius 12 cm moves along the groove steadily and completes 7 revolutions in 100s. The linear speed of the insect is
A4.3 cm s−1B5.3 cm s−1C6.3 cm s−1D7.3 cm s−1An object of mass 0.5 kg, moving in a circular path of radius 0.25m, experiences a centripetal acceleration of constant magnitude 9 m/s2. What is the object's angular speed?
A2.3rad/sB4.5rad/sC6rad/sD12rad/s
An insect trapped in a circular groove of radius, 12 cm moves along the groove steadily and completes 7 revolutions in 100s. What is the angular speed and the linear speed of the motion ?
What is the magnitude of the centripetal acceleration in above problem ?
View SolutionA car moving on a straight path covers a distance of 1 km due east in 100 s. What is (i) the speed and (ii) the velocity, of car?
View SolutionA satellite moving in a circular orbit at an altitude of 1000 km completes one revolution round the earth in 105 minutes. What is (z) its angular velocity and speed? Radius of the earth =6.4×106m.
View SolutionA cyclist starts form centre O of a circular park of radius 1km and moves along the path OPRQO as shown in the figure . If he maintains constant speed of 10ms−1, what is his acceleration at point (R )in magnitude and direction ?
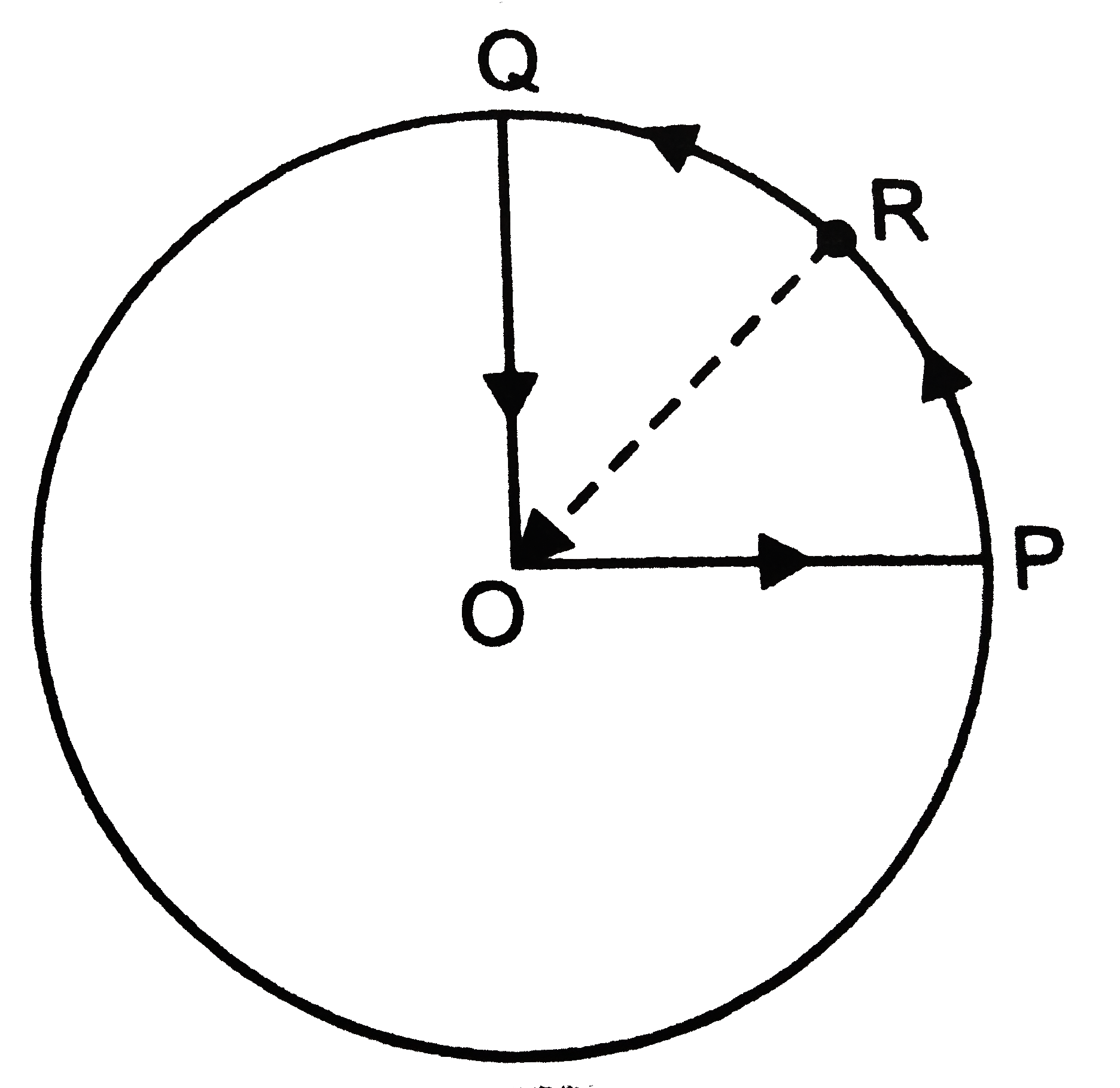 .
.
View SolutionIf a particle is moving on a circular path with constant speed, then the angle between the direction of acceleration and its position vector w.r.t. centre of circle will be
View SolutionA cyclist starts form centre O of a circular park of radius 1km and moves along the path OPRQO as shown Fig. 2 (EP).15. If he maintains constant speed of 10ms−1, what is his acceleration at point (R )in magnitude and direction ?
 .
.
View Solution
