Electrons are emitted with zero velocity from a metal surface when it is exposed to radiation of wavelength Å . Calculate threshold frequency and work function of the metal.
To solve the problem of calculating the threshold frequency v0 and the work function W0 of the metal when electrons are emitted with zero velocity from a metal surface exposed to radiation of wavelength 6800 Å, we will follow these steps:
Step 1: Convert Wavelength to Meters
The given wavelength is in angstroms, and we need to convert it to meters for our calculations.
Wavelength(λ)=6800Å=6800×10−10m
Step 2: Calculate Energy of the Incident Radiation
The energy E of the radiation can be calculated using the formula:
E=hcλ
where:
- h=6.626×10−34Js (Planck's constant)
- c=3×108m/s (speed of light)
- λ=6800×10−10m
Substituting the values:
E=(6.626×10−34Js)(3×108m/s)6800×10−10m
Calculating the above expression:
E≈2.92×10−19J
Step 3: Determine the Work Function W0
Since electrons are emitted with zero velocity, their kinetic energy is zero. Thus, the work function W0 is equal to the energy of the incident radiation:
W0=E=2.92×10−19J
Step 4: Calculate the Threshold Frequency v0
The threshold frequency v0 can be calculated using the formula:
v0=cλ
Substituting the values:
v0=3×108m/s6800×10−10m
Calculating the above expression:
v0≈4.42×1014Hz
Final Results
- Threshold Frequency v0: 4.42×1014Hz
- Work Function W0: 2.92×10−19J
The given wavelength is in angstroms, and we need to convert it to meters for our calculations.
Wavelength(λ)=6800Å=6800×10−10m
The energy E of the radiation can be calculated using the formula:
E=hcλ
where:
- h=6.626×10−34Js (Planck's constant)
- c=3×108m/s (speed of light)
- λ=6800×10−10m
E=(6.626×10−34Js)(3×108m/s)6800×10−10m
Calculating the above expression:
E≈2.92×10−19J
Since electrons are emitted with zero velocity, their kinetic energy is zero. Thus, the work function W0 is equal to the energy of the incident radiation:
W0=E=2.92×10−19J
The threshold frequency v0 can be calculated using the formula:
v0=cλ
Substituting the values:
v0=3×108m/s6800×10−10m
Calculating the above expression:
v0≈4.42×1014Hz
- Threshold Frequency v0: 4.42×1014Hz
- Work Function W0: 2.92×10−19J
Topper's Solved these Questions
Similar Questions
A monochromatic light soure of frequency f illuminates a metallic surface and ejects photoelectrons. The photoelectrons having maximum energy are just able to ionize the hydrogen atoms in ground state. When the whole experiment is repeated with an incident radiation of frequency 56f, the photoelectrons so emitted are able to excite the hydrogen atom beam which then emits a radiation of wavelength 1215Å. (a) What is the frequency of radiation? (b) Find the work- function of the metal.
View SolutionCalculate the threshold freqency of the metal from the photoelectrons are emitted with zero velocity when exposed to radiation of wavelength 6800 Å
Hint :KE=12mv2
hv=hv0+KE,KE=0
v=cλ
View SolutionCalculate the threshold freqency of the metalfrom the photoelectronsare emitted with zero velocity when exposed to radiation of wavelength 6800 Å
Hint :KE=12mv2
hv=hv0+KE,KE=0
v=cλ
View SolutionPhoto electrons are liberated by ultraviolet light of wavelength 3000Å from a metalic surface for which the photoelectric threshold wavelength is 4000Å. Calculate the de Broglie wavelength of electrons emitted with maximum kinetic energy.
View SolutionA metal sheet of silver is exposed to ultraviolet radiation of wavelength 1810A0. The threshold wavelength of silver is 2640A0 Calculate the maximum energy of emitted electron.
View SolutionThe kinetic energy of most energetic electrons emitted from a metallic surface is doubled when the wavelength λ of the incident radiation is changed from 400 nm to 310 nm. The work function of the metal is
View SolutionThe emission of electrons from a metal surface exposed rto light radaition of appropriate wavelength is called photoelectroic effect .The emmited electron are called photo=-weklectron work function of threshold energy may be defined as the minimum amount of energy required to ejercted electron from a most surface .According to Einstein
Maximum kinetic energy of ejected electron = Aborbed energy - Work function
12mv2max=h(v)−h(vn)=hv[1λ−01λn]
Where vn and λ0 are thereshold frequency and threshold wavelength respectively
Sopping potential : it is the miximum potential at which the photoelectric current becomes zero if V0 is the stopping potential eV0=h(v−v0)
Whaich of the following is the graph between the frequency (V) of the incident radiation and the stopping potential (v) ?
View SolutionThe emission of electrons from a metal surface exposed rto light radaition of appropriate wavelength is called photoelectroic effect .The emmited electron are called photo=-weklectron work function of threshold energy may be defined as the minimum amount of energy required to ejercted electron from a most surface .According to Einstein
Maximum kinetic energy of ejected electron = Aborbed energy - Work function
12mv2max=h(v)−h(vn)=hv[1λ−01λn]
Where vn and λ0 are thereshold frequency and threshold wavelength respectively
Sopping potential : it is the miximum potential at which the photoelectric current becomes zero if V0 is the stopping potential eV0=h(v−v0)
The stopping potential as a function on electron frtequency is plotted for two photoelectric surface A abd B The graph show that the work function of A is
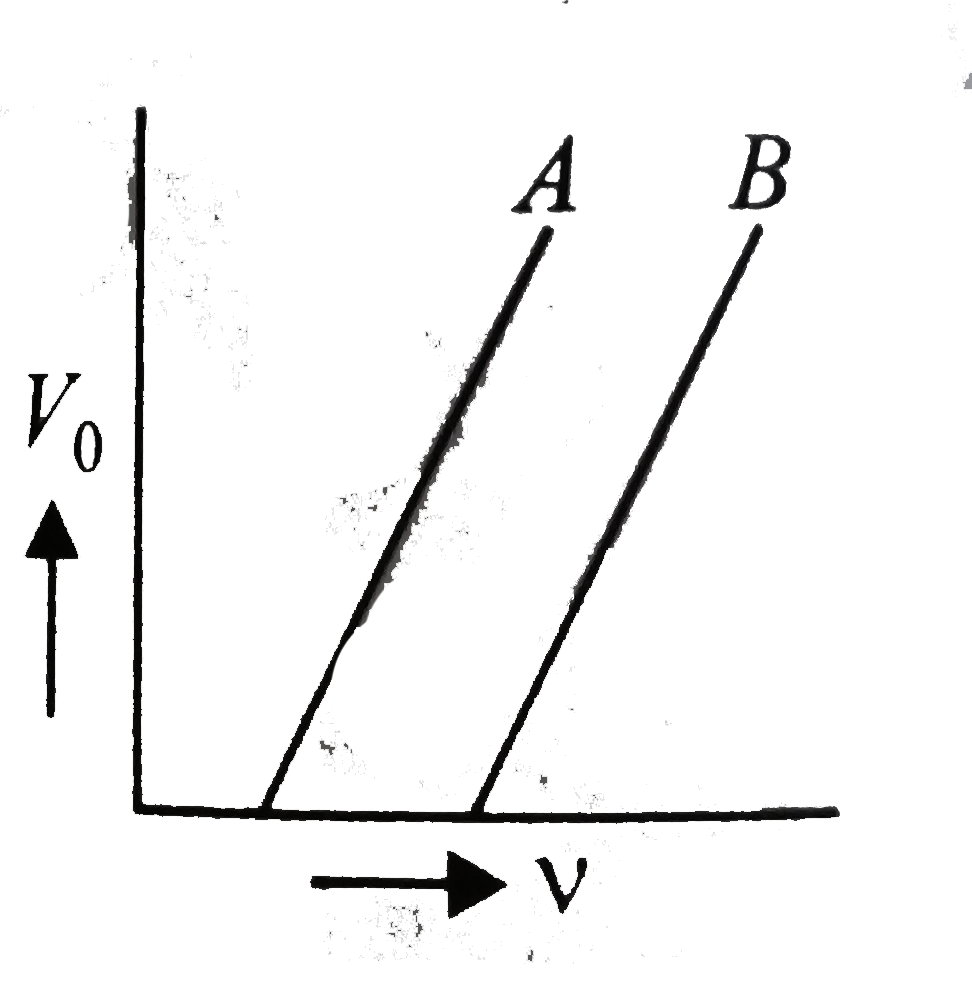
View SolutionThe kinetic energy of the most energetic photoelectrons emitted from a metal surface is doubled when the wavelength of the incident radiation is reduced from λ1 to λ2 The work function of the metal is
View SolutionThe emission of electrons from a metal surface exposed rto light radaition of appropriate wavelength is called photoelectric effect .The emmited electron are called photo=-weklectron work function of threshold energy may be defined as the minimum amount of energy required to ejected electron from a most surface .According to Einstein
Maximum kinetic energy of ejected electron = Absorbed energy - Work function
12mv2max=h(v)−h(vn)=hv[1λ−1λn]
Where vn and λ0 are thereshold frequency and threshold wavelength respectively
Sopping potential : it is the miximum potential at which the photoelectric current becomes zero if V0 is the stopping potential eV0=h(v−v0)
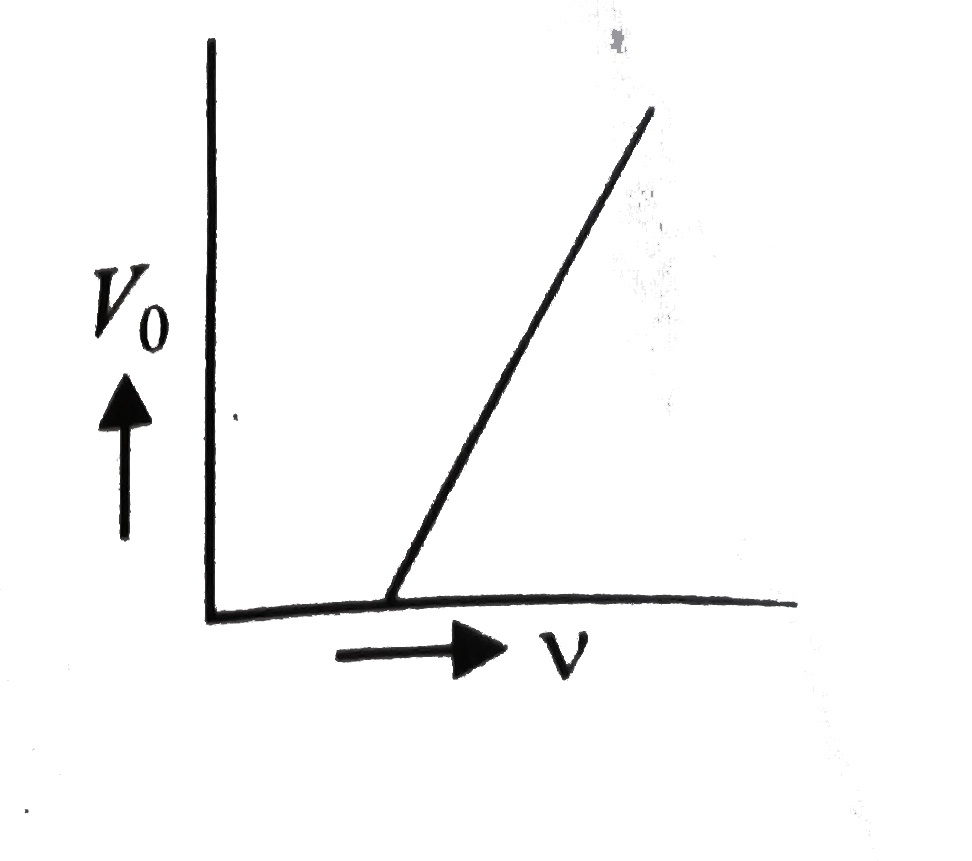
In the photoelectric currect effect the shape of strainght line graph between stopping potential (V0) and frequency of incident light (V) gves
View Solution
