2×108 atoms of carbon are arranged side by side. Calculate the radius of carbon atom if the length of this arrangement is 2.4cm.
To solve the problem of finding the radius of a carbon atom when 2x108 atoms are arranged side by side in a total length of 2.4cm, we can follow these steps:
1. Identify the Given Information:
- Total length of the arrangement = 2.4cm
- Total number of carbon atoms = 2x108
2. Calculate the Diameter of One Carbon Atom:
- Since the atoms are arranged side by side, the total length is equal to the number of atoms multiplied by the diameter of one atom.
- Let the diameter of one carbon atom be d.
- Therefore, we can set up the equation:
\(
\text{Total Length} = \text{Number of Atoms} \times \text{Diameter}
\)
\(
2.4 \, \text{cm} = (2 \times 10^8) \times d
\)
3. Rearranging the Equation to Solve for Diameter:
- Rearranging the equation gives:
\(
d = \frac{2.4 \, \text{cm}}{2 \times 10^8}
\)
4. Calculating the Diameter:
- Performing the calculation:
\(
d = \frac{2.4}{2 \times 10^8} = \frac{2.4}{2} \times 10^{-8} = 1.2 \times 10^{-8} \, \text{cm}
\)
5. Finding the Radius of the Carbon Atom:
- The radius r is half of the diameter:
\(
r = \frac{d}{2} = \frac{1.2 \times 10^{-8} \, \text{cm}}{2} = 0.6 \times 10^{-8} \, \text{cm}
\)
6. Converting Radius to Meters:
- To convert centimeters to meters, we use the conversion factor \(1 \, \text{cm} = 0.01 \, \text{m}\):
\(
r = 0.6 \times 10^{-8} \, \text{cm} = 0.6 \times 10^{-8} \times 0.01 \, \text{m} = 0.6 \times 10^{-10} \, \text{m} = 6.0 \times 10^{-11} \, \text{m}
\)
Final Answer:
The radius of a carbon atom is \(6.0 \times 10^{-11} \, \text{m}\).
- Total length of the arrangement = 2.4cm
- Total number of carbon atoms = 2x108
- Since the atoms are arranged side by side, the total length is equal to the number of atoms multiplied by the diameter of one atom.
- Let the diameter of one carbon atom be d.
- Therefore, we can set up the equation:
\(
\text{Total Length} = \text{Number of Atoms} \times \text{Diameter}
\)
\(
2.4 \, \text{cm} = (2 \times 10^8) \times d
\)
- Rearranging the equation gives:
\(
d = \frac{2.4 \, \text{cm}}{2 \times 10^8}
\)
- Performing the calculation:
\(
d = \frac{2.4}{2 \times 10^8} = \frac{2.4}{2} \times 10^{-8} = 1.2 \times 10^{-8} \, \text{cm}
\)
- The radius r is half of the diameter:
\(
r = \frac{d}{2} = \frac{1.2 \times 10^{-8} \, \text{cm}}{2} = 0.6 \times 10^{-8} \, \text{cm}
\)
- To convert centimeters to meters, we use the conversion factor \(1 \, \text{cm} = 0.01 \, \text{m}\):
\(
r = 0.6 \times 10^{-8} \, \text{cm} = 0.6 \times 10^{-8} \times 0.01 \, \text{m} = 0.6 \times 10^{-10} \, \text{m} = 6.0 \times 10^{-11} \, \text{m}
\)
The radius of a carbon atom is \(6.0 \times 10^{-11} \, \text{m}\).
Topper's Solved these Questions
Similar Questions
2×106 atoms of carbon are aranged state by side .Calculate the carbon atom if the length of this arrangement is 2.4cm
View SolutionCalculate the formal charge on C-atom in carbonate ion.
View SolutionThe correct relation for radius of atom and edge - length in case of fcc arrangement is
View SolutionExplain the arrangement of carbon atoms in graphite.
View SolutionOne tonne of air contains 2×10−3 g of carbon as smoke. Calculate the concentration of carbon in ppm in air.
View SolutionCalculate the formal charge on atoms in carbonate (CO2−3) .
View SolutionThe configuration of 1 and 2 carbon atom in the following compounds is :
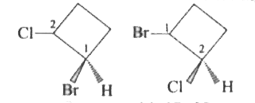
View SolutionCalculate
number of atoms in 1.2 g of carbon.
View SolutionWhen the atoms Li,Be,B and Na are arranged in order of increasing atomic radius, what is the correct order?
View SolutionNumber of sp2 hybrid carbon atoms in aspartame is
View Solution
