Theorem 7.2 : Angles opposite to equal sides of an isosceles triangle are equal.
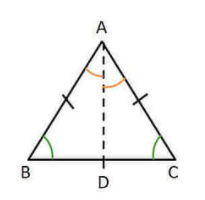
In △BAD and △CAD
AB=AC given
∠BAD=∠CAD by construction
AD=AD common
By SAS congruence rule,
△BAD and △CAD are congruent.
Thus,
∠B=∠C
Topper's Solved these Questions
- NCERT THEOREMSBOOK - NCERT ENGLISHCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 7.3
1 Video
- NCERT THEOREMSBOOK - NCERT ENGLISHCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 7.4
1 Video
- NCERT THEOREMSBOOK - NCERT ENGLISHCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 7.1
1 Video
- LINES AND ANGLESBOOK - NCERT ENGLISHCHAPTER - LINES AND ANGLESEXERCISE - Exercise 6.1
6 Videos
- NUMBER SYSTEMSBOOK - NCERT ENGLISHCHAPTER - NUMBER SYSTEMSEXERCISE - EXERCISE 1.4
2 Videos
Similar Questions
Theorem 7.3 : The sides opposite to equal angles of a triangle are equal.
View SolutionWhich of the following statements are true (T) and which are false (F): Side opposite to equal angles of a triangle may be unequal. Angle opposite to equal sides of a triangle are equal. The measure of each angle of an equilateral triangle is 600 If the altitude from one vertex of a triangle bisects the opposite side, then the triangle may be isosceles. The bisectors of two equal angles of a triangle are equal. If the bisector of the vertical angle of a triangle bisects the base, then the triangle may be isosceles. The two altitudes corresponding to two equal sides of a triangle need not be equal. If any two sides of a right triangle are respectively equal to two sides of other right triangle, then the two triangles are congruent. Two right triangles are congruent if hypotenuse and a side of one triangle are respectively equal to the hypotenuse and a side of the other triangle.
View SolutionFill in the blanks in the following so that each of the following statements is true.
(i) Sides opposite to equal angles of a triangle are .......
(ii) Angle opposite to equal sides of a triangle are .......
(iii) In an equilateral triangle all angles are .......
(iv) In a ABC if ∠A=∠C, then AB= .....
(v) If altitudes CE and BF of a triangle ABC are equal, then AB= ........
(vi) In an isosceles triangle ABC with AB=AC, if BD and CE are its altitudes, then BD is .......... CE.
(vii) In right triangles ABC and DEF, if hypotenuse AB=EF and side AC=DE, then ABC ≅ ....
View SolutionProve that the circle drawn on any one of the equal sides of an isosceles triangle as diameter bisects the base.
View SolutionProve that the circle drawn on any one of the equal sides of an isosceles triangle as diameter bisects the base.
View SolutionFind the measures of each of the two equal angles of an isosceles right-angled triangle.
View SolutionTheorem 7.4 (SSS congruence rule) : If three sides of one triangle are equal to the three sides of another triangle, then the two triangles are congruent.
View SolutionThe two equal sides of an isosceles triangle with fixed base b are decreasing at the rate of 3cm/s. How fast is the area decreasing when the two equal sides are equal to the base?
View SolutionThe two equal sides of an isosceles triangle with fixed base b are decreasing at the rate of 3cm/s. How fast is the area decreasing when the two equal sides are equal to the base?
View SolutionThe two equal sides of an isosceles triangle with fixed base b are decreasing at the rate of 3 cm per second. How fast is the area decreasing when the two equal sides are equal to the base ?
View Solution