If the diagonals of a quadrilateral bisect each other, then the quadrilateral is a parallelogram.
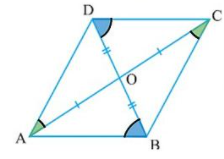
In △AOD and △COB
OA=OC (given)
∠AOD=∠COB (vertically opposite angles)
OD=OB (given)
Thus, by SAS congruency, △AOD and △COB are congruent.
Therefore, ∠OAD=∠OCB
For lines AB and CD with transversal BD,
∠ABO and ∠CDO are alternate angles and are equal.
Thus, AB∣∣CD
Similarly, we can prove that AD∣∣BC
Topper's Solved these Questions
- NCERT THEOREMSBOOK - NCERT ENGLISHCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 8.8
1 Videos
- NCERT THEOREMSBOOK - NCERT ENGLISHCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 8.9
1 Videos
- NCERT THEOREMSBOOK - NCERT ENGLISHCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 8.6
1 Videos
- LINES AND ANGLESBOOK - NCERT ENGLISHCHAPTER - LINES AND ANGLESEXERCISE - Exercise 6.1
6 Videos
- NUMBER SYSTEMSBOOK - NCERT ENGLISHCHAPTER - NUMBER SYSTEMSEXERCISE - EXERCISE 1.4
2 Videos
Similar Questions
Given statements in (a) and (b). Identify the statements given below as contrapositive or converse of each other.(a) If you live in Delhi, then you have winter clothes. (i) If you do not have winter clothes, then you do not live in Delhi. (ii) If you have winter clothes, then you live in Delhi.(b) If a quadrilateral is a parallelogram, then its diagonals bisect each other. (i) If the diagonals of a quadrilateral do not bisect each other, then the quadrilateral is not a parallelogram. (ii) If the diagonals of a quadrilateral bisect each other, then it is a parallelogram.
View SolutionState True or False
If the diagonals of a quadrilateral bisect each other at right angle, the quadrilateral is a square.
View SolutionIf the diagonals of a quadrilateral bisect each other at right angle, then the quadrilateral is a (a) parallelogram (b) rectangle (c) rhombus (d) kite
View SolutionThe diagonals of a parallelogram bisect each other.
View SolutionThe diagonals of a rectangle bisect each other
View SolutionProve using vectors: The diagonals of a quadrilateral bisect each other iff it is a parallelogram.
View SolutionProve that; If the diagonals of a quadrilateral bisect each other at right angles, then it is a rhombus.
View SolutionIf the diagonals of a quadrilateral bisect each other at right angle, prove that the quadrilateral is a rhombus.
A rhombus has all its four sides equal.
View SolutionIf one diagonal of a quadrilateral bisects the other, then it also bisects the quadrilateral.
View SolutionShow that if the diagonals of a quadrilateral bisect each other at right angles, then it is a rhombus.
View Solution
