If A(1,a),B(a,a2) and C(a2,a2) are the vertices of a triangle which are equidistant from the origin, then the centroid of the triangle ABC is at the point
Step by step video & image solution for If A(1, a), B(a, a^2) and C(a^2, a^2) are the vertices of a triangle which are equidistant from the origin, then the centroid of the triangle ABC is at the point by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
lf origin is centroid of triangle with vertices (a, 1 , 3), (- 2, b, -5) and (4, 7, c), then (a, b, c) ≡
04:03View SolutionABC is triangle. Locate a point in the interior of Δ ABC which is equidistant from all the vertices of ΔABC.
02:48View SolutionTwo vertices of a △ABC are B (- 3,1) and C (0,-2) and its centroid is at the origin. The third vertex A is:
01:54View SolutionABC is a triangle. Locate a point in the interior of ΔABCwhich is equidistant from all the vertices of ΔABC
02:49View SolutionSuppose z1,z2,z3 represent the vertices A, B and C respectively of a ΔABC with centroid at G. If the mid point of AG is the origin, then
01:57View SolutionABC is a triangle. Locate a point in the interior of △ ABC which is equidistant from all the vertices of △ ABC
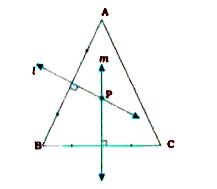 03:37View Solution
03:37View SolutionIf the origin is the centroid of a triangle ABC having vertices A(a, 1, 3), B(-2, b, -5) and C(4, 7, c), Find the values of a, b, c.
03:09View SolutionA(1,4),B(3,2)andC(7,5) are vertices of a triangle ABC. Find :
the co-ordinates of the centroid of triangle ABC.02:10View Solution

