The diagonals of a quadrilateral ABCD intersect each other at the point O such that AOBO=CODO.
Show that ABCD is a trapezium.
 Solution in Kannada
Solution in Kannada
Step by step video & image solution for The diagonals of a quadrilateral ABCD intersect each other at the point O such that (AO)/(BO)=(CO)/(DO). Show that ABCD is a trapezium. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLESBOOK - ZEN PUBLICATIONCHAPTER - TRIANGLESEXERCISE - TEXTUAL EXERCISES (EXERCISE 2.3)
16 Videos
- TRIANGLESBOOK - ZEN PUBLICATIONCHAPTER - TRIANGLESEXERCISE - TEXTUAL EXERCISES (EXERCISE 2.4)
9 Videos
- TRIANGLESBOOK - ZEN PUBLICATIONCHAPTER - TRIANGLESEXERCISE - TEXTUAL EXERCISES (EXERCISE 2.1)
7 Videos
- SURFACE AREAS AND VOLUMESBOOK - ZEN PUBLICATIONCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - ZEE ADDITIONAL QUESTIONS -HOTS [HIGHER ORDER THINKING SKILLS]-QUESTIONS
11 Videos
Similar Questions
If the areas of two similar triangles are equal, prove that they are congruent.

View SolutionLet ΔABC~ΔDEF and their areas be , respectively , 64cm2and121cm2 . If EF = 15.4 cm, find BC
View SolutionFill in the blanks using the correct word given in brackets :
All circles are ……..... ( congruent , similar )
View SolutionA sector of a circular is of radius 12 cm and angle 120∘. It is rolled up so that two bounding radii are joined together to form a cone. Find the volume of the cone.
View SolutionThe diagonals of a quadrilateral ABCD intersect each other at the point O such that AOBO=CODO. Show that ABCD is a trapezium.
View SolutionABCD is a trapezium in which AB||DC and its diagonals intersect each other at the point O. Show that AOBO=CODO.
View SolutionDetermine the point of symmetry of a regular hexagon.
View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionThe inequation represented by the graph given below is :
View SolutionThe inequation that best describes the graph given below is _________
View SolutionThe inequation that best describes the following graph is _________
View Solutionएक चतर्भुज ABCD के विकर्ण परस्पर बिन्दु O पर इस प्रकार प्रतिच्छेद करते हैं कि AOBO=CODO है दर्शाइए कि ABCD एक समलम्ब है ।
View Solutionचतुर्भुज ABCD के विकर्ण एक -दूसरे को बिन्दु O पर इस प्रकार प्रतिच्छेद करते है कि AOBO=CODO तो सिद्ध कीजिए कि ABCD एक समलम्ब चतुर्भुज है ।
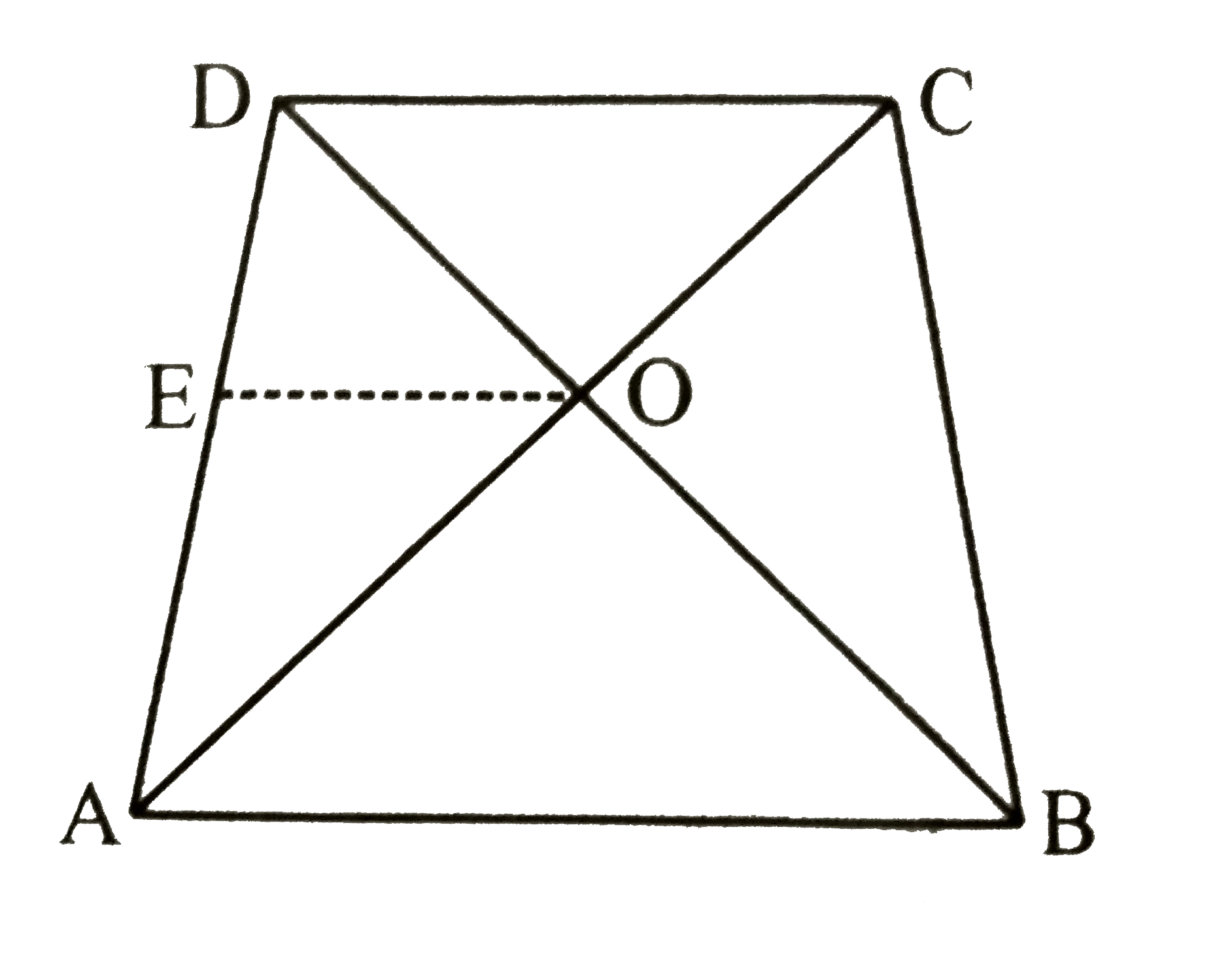
View SolutionThe diagonals of the quadrilateral ABCD intersect each other at O in such a way that AOBO=CODO. Prove that ABCD is a trapezium.
View Solution
