If each pair ofthe following three equations x: $x^{2} + p_{1} x + q_{1} = 0$ . $x^{2} + p_{2} x + q_{2} = 0$ , $x^{2} + p_{3} x + q_{3} = 0$ , has exactly one root common, prove that ${(p_{1} + p_{2} + p_{3})}_{1}^{2} = 4 (p_{1} p_{2} + p_{2} p_{3} + p_{3} p_{1} - q_{1} - q_{2} - q_{3}]$

Video Solution

Answer

Step by step video & image solution for If each pair ofthe following three equations x: x^2+p_1x + q_1 =0.x^2 + p_2x +q_2=0 , x^2+p_3x+q_3=0 , has exactly one root common, prove that (p_1+p_2+p_3)^2=4(p_1p_2+p_2p_3+p_3p_1-q_1-q_2-q_3] by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 MATHS COMPLEX NUMBERS AND QUADRATIC EQUATIONS

Similar Questions

If the lines $p_{1} x + q_{1} y = 1, p_{2} x + q_{2} y = 1 a n d p_{3} x + q_{3} y = 1,$ be concurrent, show that the point $(p_{1}, q_{1}), (p_{2}, q_{2}) a n d (p_{3}, q_{3})$ are collinear.
View Solution
Three charges $- q_{1}, + q_{2} and - q_{3}$ are placed as shown in the figure. The x - component of the force on $q_{1}$ is proportional to

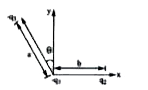
View Solution
A charge q_1 exerts some force on a second charge q_2 . If third charge q_3 is brought near charge q_1 , the force of q_1 exerted on q_2
View Solution
Three point charges $Q_{1}, Q_{2}, and Q_{3}$ in that order are placed equally spaced along a straight line. $Q_{2} and Q_{3}$ are equal in magnitude but opposite in sign. If the net force on $Q_{3}$ is zero, the value of $Q_{1}$ is
View Solution
$q_{1}$ , $q_{2}$ , and $q_{3}$ , are three point charges located in free space. br What is the force between $q_{1}$ , and $q_{2}$ , if $q_{3}$ , is absent?
View Solution
$q_{1}$ , $q_{2}$ , and $q_{3}$ , are three point charges located in free space. br What is the force between $q_{1}$ , and $q_{2}$ , if $q_{3}$ , is, present?
View Solution
If $x^{2} - 2 r p_{r} x + r = 0; r = 1, 2, 3$ are three quadratic equations of which each pair has exactly one root common, then the number of solutions of the triplet $(p_{1}, p_{2}, p_{3})$ is
View Solution
Let $V_{r}$ denote the sum of the first r terms of an arithmetic progression (AP) whose first term is r and the common difference is $(2 r - 1)$ . Let $T_{r} = V_{r + 1} - V_{r} - 2$ and Q_r =T_(r+1)-T_r for r=1,2 $W h i c h o \neq o f t h e f o l l o w \in g i s a c or r e c t s t a t e m e n t ? (A)$ Q_1, Q_2, Q_3………….., $a r e \in A . P . w i t h c o m m o n d \Leftrightarrow e r e n c e 5 (B)$ Q_1, Q_2, Q_3………….., $a r e \in A . P . w i t h c o m m o n d \Leftrightarrow e r e n c e 6 (C)$ Q_1, Q_2, Q_3………….., $a r e \in A . P . w i t h c o m m o n d \Leftrightarrow e r e n c e 11 (D)$ Q_1=Q_2=Q_3
View Solution

If each pair ofthe following three equations x: x2+p1x+q1=0.x2+p2x+q2=0 , x2+p3x+q3=0 , has exactly one root common, prove that (p1+p2+p3)2=4(p1p2+p2p3+p3p1−q1−q2−q3]

Similar Questions

Recommended Questions

If each pair ofthe following three equations x: $x^{2} + p_{1} x + q_{1} = 0$ . $x^{2} + p_{2} x + q_{2} = 0$ , $x^{2} + p_{3} x + q_{3} = 0$ , has exactly one root common, prove that ${(p_{1} + p_{2} + p_{3})}_{1}^{2} = 4 (p_{1} p_{2} + p_{2} p_{3} + p_{3} p_{1} - q_{1} - q_{2} - q_{3}]$