To draw a pair of tangents to a circle which are inclined to each other at angle x∘, it is required to draw tangents at the end points of those two radii of the circle, the angle between which is-
180∘ - x∘
90∘ +x∘
90∘ - x∘
180∘ + x∘
The correct Answer is:A
Similar Questions
To draw a pair of tangents to a circle inclined to each other at 40∘, the angle at the centre of the circle between the two radii is _______
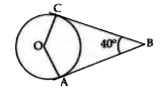
View SolutionTo draw a pair of tangents to a circle inclined to each other at an angle of 60∘, it is required to draw the two radii of the circle intersecting at an angle of
View SolutionTo draw a pair of tangents to a circle which are inclined to each other at an angle of 60^(@) , it is required to draw tangents at end points of those two radii of the circle, the angle between them should be
View SolutionTo draw a pair of tangents to a circle which are inclined to each other at an angle of 1000, it is required to draw tangents at end points of those two radii of the circle, the angle between which should be: 1000 (b) 500 (c) 800 (d) 2000
View SolutionTo draw a pair of tangents to a circle which are inclined to each other at an angle of 35°, it is required to draw tangents at the end points of two radii which are inclined to each other at an angle of ..........
View SolutionTo draw two tangents to a circle which are inclined at an angle of 60° the perpendiculars are to be drawn at the ends of two radii which are inclined at an angle of ..........
View SolutionTo draw two tangents to a circle which are perpendicular to each other, the perpendiculars are to be drawn at the ends of two radii which are inclined at an angle of……..
View Solution
