A lift is going up with constant velocity v0. Calculate the work done by normal force Fg from the reference frame of ground and lift in time t.
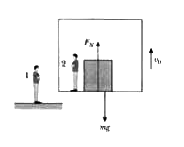
The correct Answer is:FN.ut; zero
Step by step video & image solution for A lift is going up with constant velocity v_(0). Calculate the work done by normal force F_(g) from the reference frame of ground and lift in time t. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PROBLEMS
58 Videos
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PRACTICE QUESTIONS (Single Correct Choice Type)
59 Videos
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PRACTICE QUESTIONS (Integer Type)
5 Videos
- WAVES-IBOOK - RESNICK AND HALLIDAYCHAPTER - WAVES-IEXERCISE - Practice Questions (Integer Type)
4 Videos
Similar Questions
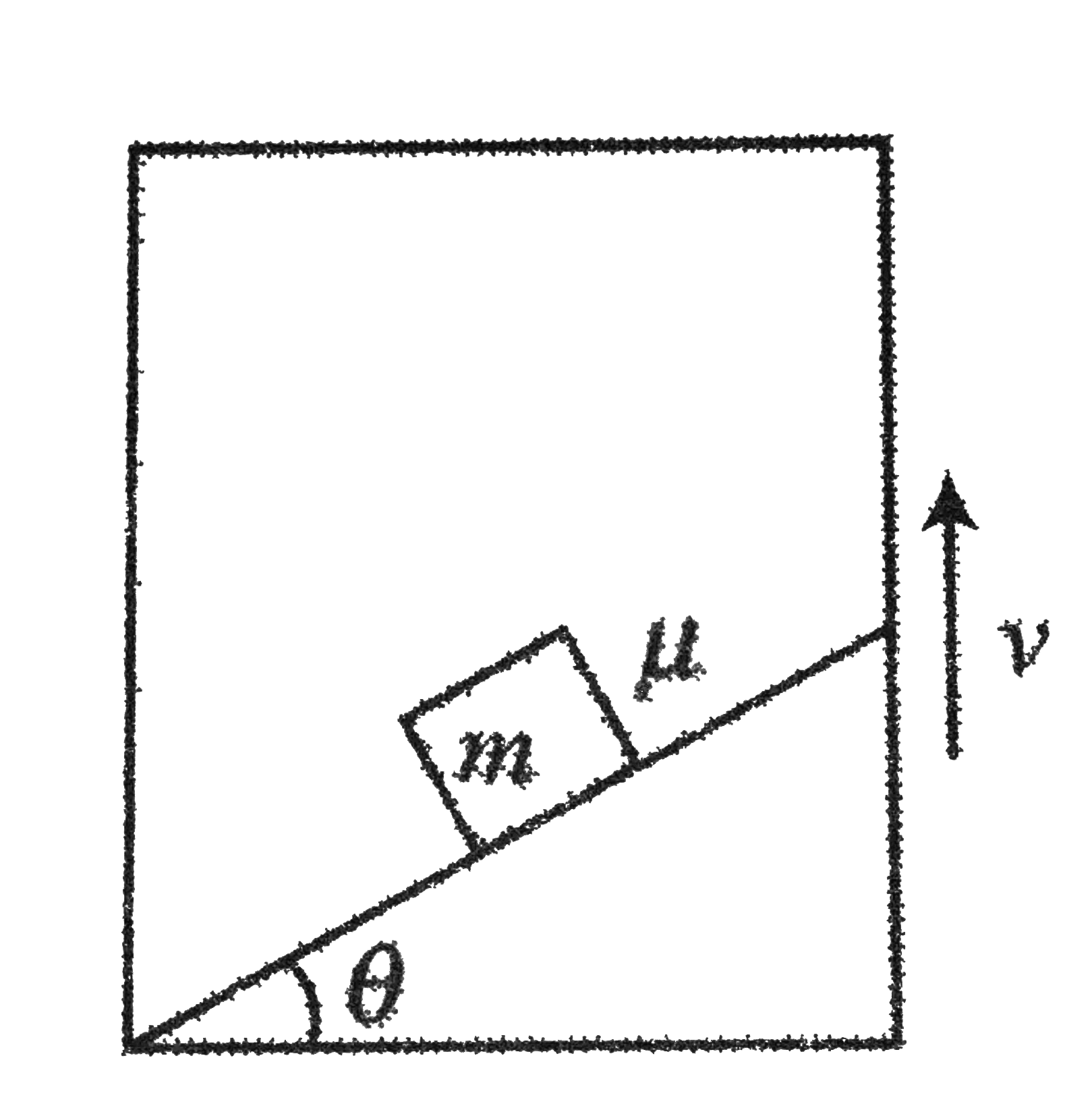
An inclined plane is moving up with constant velocity v. A block kept on incline is at rest. Calculate the work done by gravity, friction force, and normal reaciton on block in time interval of t.
View SolutionCalculate the work done by a student in lifting 0.5kg book from the ground and keeping it ona shelf 1.5m high.
View Solution
Knowledge Check
A block of 1 kg is kept on a rough surface of an elevator moving up with constant velocity of 5 m/s. In 10 second work done by normal reaction (no sliding on incline surface)
(i) from ground frame is 320 J
(ii) is equal to work done by friction force in elevator frame
(iii) is equal to work done by friction in ground frameA(i)B(ii), (iii)C(i), (ii)Donly (iii).A simple pendulum is oscillating in a lift. If the lift is going down with constant velocity, the time period of the simple pendulum is T1. If the lift is going down with some retardation its time period is T2, then
AT1>T2BT1<T2CT1=T2Ddepends upon the mass of the pendulum bob
A spring block system is kept inside a lift moving with a constant velocity v0 as shown in figure. Block is in equilibrium and at rest with respect to lift. The lift is suddenly stoped at time t=0. Taking upward direction as the positive direction, write x−t equation of the block.
View SolutionA stone falls down without initial velocity from a height h onto the Earth's surface. The air drag assumed to be negligible, the stone hits the ground with velocity v0=√2gh relative to the Earth. Obtain the same formula in terms of the reference frame "falling" to the Earth with a constant velocity v0.
View SolutionA uniform disc of mass m and radius R is projected horizontally with velocity v0 on a rough horizontal floor so that it starts with a purely sliding motion at t= 0. After t0 seconds it acquires a purely rolling motion.
(a) Calculate the velocity of the centre of mass of the disc at t0
(b) Assuming the coefficient of friction to be μ, calculate to. Also calculate the work done by the frictional force as a function of time and the total work done by it over a time t muchlonger than t0.
View SolutionA simple pendulam of length l is suspended from the ceiling of a moving lift. Find the time period of the pendulam when the lift is going up with accelerationF.
[Hint: Bring the pendulam to rest by applying inertia force (D' Alembert's principle) and consider its motion in this condition.]
View SolutionA simple pendulum is hung in a stationary lift and its periodic time is T. What will be the effect on its periodic time T if
(i) the lift is moving up with a uniform velocity v,
(ii) the lift is moving up with a uniform acceleration a, and (iii) the lift is moving down with a uniform acceleration a?
View SolutionA lift ascends with constant acceleration f, then with constant velocity, and finally stops under a constant retardation f. If the total distance ascended is s and the total time occupied is t, then the time during which the lift is ascending with constant velocity is (t2−4s/f)1/2
View Solution
