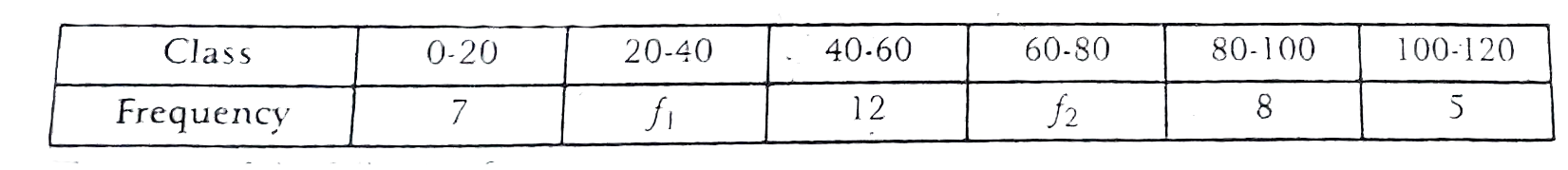
The table below gives an incomplete frequency distribution with two missing frequencies f1andf2
∣∣
∣
∣
∣
∣
∣
∣∣Value of xFrequency0f11f2243443∣∣
∣
∣
∣
∣
∣
∣∣
The total frequency is 18 and the arithmetic mean of x is 2.
What is the coefficient of variance ?
2003
50√59
600√5
150
The correct Answer is:A
To solve the problem, we need to find the missing frequencies f1 and f2 and then calculate the coefficient of variation. Here’s a step-by-step solution:
Step 1: Set up the frequency distribution
We have the following frequency distribution:
| Value of x | Frequency |
|------------|-----------|
| 0 | f1 |
| 1 | f2 |
| 2 | 4 |
| 3 | 4 |
| 4 | 3 |
The total frequency is given as 18. Therefore, we can write the equation:
f1+f2+4+4+3=18
Step 2: Simplify the equation
Calculating the sum of the known frequencies:
f1+f2+11=18
Now, we can isolate f1+f2:
f1+f2=18−11=7(Equation 1)
Step 3: Use the mean to find another equation
The arithmetic mean is given as 2. The formula for the mean is:
Mean=∑(x⋅f)∑f
Calculating ∑(x⋅f):
∑(x⋅f)=0⋅f1+1⋅f2+2⋅4+3⋅4+4⋅3
This simplifies to:
0+f2+8+12+12=f2+32
Now, substituting into the mean formula:
2=f2+3218
Step 4: Solve for f2
Multiplying both sides by 18:
36=f2+32
Now, isolating f2:
f2=36−32=4(Equation 2)
Step 5: Substitute f2 back into Equation 1
Now we substitute f2=4 into Equation 1:
f1+4=7
Thus,
f1=7−4=3
Step 6: Finalize the frequency distribution
Now we have:
| Value of x | Frequency |
|------------|-----------|
| 0 | 3 |
| 1 | 4 |
| 2 | 4 |
| 3 | 4 |
| 4 | 3 |
Step 7: Calculate the standard deviation
We need to calculate the standard deviation. First, we calculate ∑(x2⋅f):
∑(x2⋅f)=02⋅3+12⋅4+22⋅4+32⋅4+42⋅3
Calculating this gives:
0+4+16+36+48=104
Now, we can calculate the variance:
Variance=∑(x2⋅f)n−(∑(x⋅f)n)2
Where n=18:
Variance=10418−(3618)2
Calculating:
Variance=10418−4=104−7218=3218=169
Thus, the standard deviation σ is:
σ=√169=43
Step 8: Calculate the coefficient of variation
The coefficient of variation (CV) is given by:
CV=σMean×100
Substituting the values:
CV=432×100=43×12×100=2003
Final Answer
The coefficient of variation is 2003.
---
We have the following frequency distribution:
|------------|-----------|
| 0 | f1 |
| 1 | f2 |
| 2 | 4 |
| 3 | 4 |
| 4 | 3 |
Calculating the sum of the known frequencies:
The arithmetic mean is given as 2. The formula for the mean is:
Multiplying both sides by 18:
Now we substitute f2=4 into Equation 1:
Now we have:
|------------|-----------|
| 0 | 3 |
| 1 | 4 |
| 2 | 4 |
| 3 | 4 |
| 4 | 3 |
We need to calculate the standard deviation. First, we calculate ∑(x2⋅f):
The coefficient of variation (CV) is given by:
The coefficient of variation is 2003.
Topper's Solved these Questions
- SETS, RELATIONS, FUNCTIONS AND NUMBER SYSTEM BOOK - NDA PREVIOUS YEARSCHAPTER - SETS, RELATIONS, FUNCTIONS AND NUMBER SYSTEM EXERCISE - MCQ
271 Videos
- TRIGONOMETRY - RATIO & IDENTITY , TRIGONOMETRIC EQUATIONSBOOK - NDA PREVIOUS YEARSCHAPTER - TRIGONOMETRY - RATIO & IDENTITY , TRIGONOMETRIC EQUATIONSEXERCISE - MCQ
238 Videos
Similar Questions
Knowledge Check
The table below gives an incomplete frequency distribution with two missing frequencies f1andf2
∣∣ ∣ ∣ ∣ ∣ ∣ ∣∣Value of xFrequency0f11f2243443∣∣ ∣ ∣ ∣ ∣ ∣ ∣∣
The total frequency is 18 and the arithmetic mean of x is 2.
What is the value of f2?A4B3C2D1The table below gives an incomplete frequency distribution with two missing frequencies f1andf2
∣∣ ∣ ∣ ∣ ∣ ∣ ∣∣Value of xFrequency0f11f2243443∣∣ ∣ ∣ ∣ ∣ ∣ ∣∣
The total frequency is 18 and the arithmetic mean of x is 2.
What is the standard deviation?A√52B√53C43D169Let f1 be the frequency
Af1−f2=f3Bf2−f1=f3Cf3=12(f1+f2)Df1+f2=f3.
Observe the following data :

If the above data has mean = 50 , then missing frequencies f1andf2 are
View SolutionThe mean of the following frequency distribution is 62.8 and the sum of all frequencies is 50. Compute the missing frequencies f1 and f2:
View SolutionThe mean of the following frequency distribution is 57.6 and the number of observation is 50. Find the missing frequency f1&f2
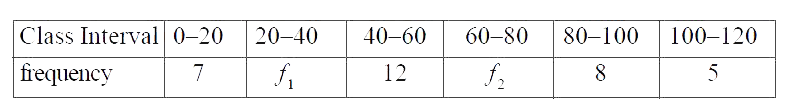
View SolutionThe total number of observations in the following distribution table is 120 and their mean is 50. Find the value of missing frequencies f1andf2 :

View SolutionConsider the following frequency distribution:
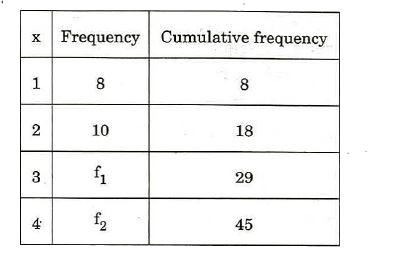
What are the values of f1, and f2, respectively?
View Solution