Rate of growth of baster is proportional to the number of bacteria present at that time. If x is the number of bacteria present any instant t, then which one of the following is correct ?(take proportional constant equal to 1)
x=log t
x=cet
ex=t
x=√t
The correct Answer is:B
Rate of growth of bacteria α number of bacteria present at that time
⇒dydxαx⇒dxdt=x ( proportional constant =1)
⇒∫1xdx=∫dt
⇒logx+logc
⇒logx−logc=t
⇒log(xc)=t
⇒xc=et
x=cet
Topper's Solved these Questions
Similar Questions
Rate of growth of bacteria is proportional to number of bacteria present at that time. If x is the number of bacteria present at any instant t, then which one of the following is correct? (take proportional constant equal to 1)
View SolutionThe rate at which a particular decay process occurs in a radio sample, is proportional to the number of radio active nuclei present . If N is the number of radio active nuclei present at some instant, the rate of change of N is dNdt=−λN. Consider radioactive decay of A to B which may further decay either to X or to Y, λ1,λ2andλ3 are decay constants for A to B decay , B to X decay and B of Y decay respectively. if at t=0 number of nuclei of A,B , X and Y are N0,N0,zero and zero respectively
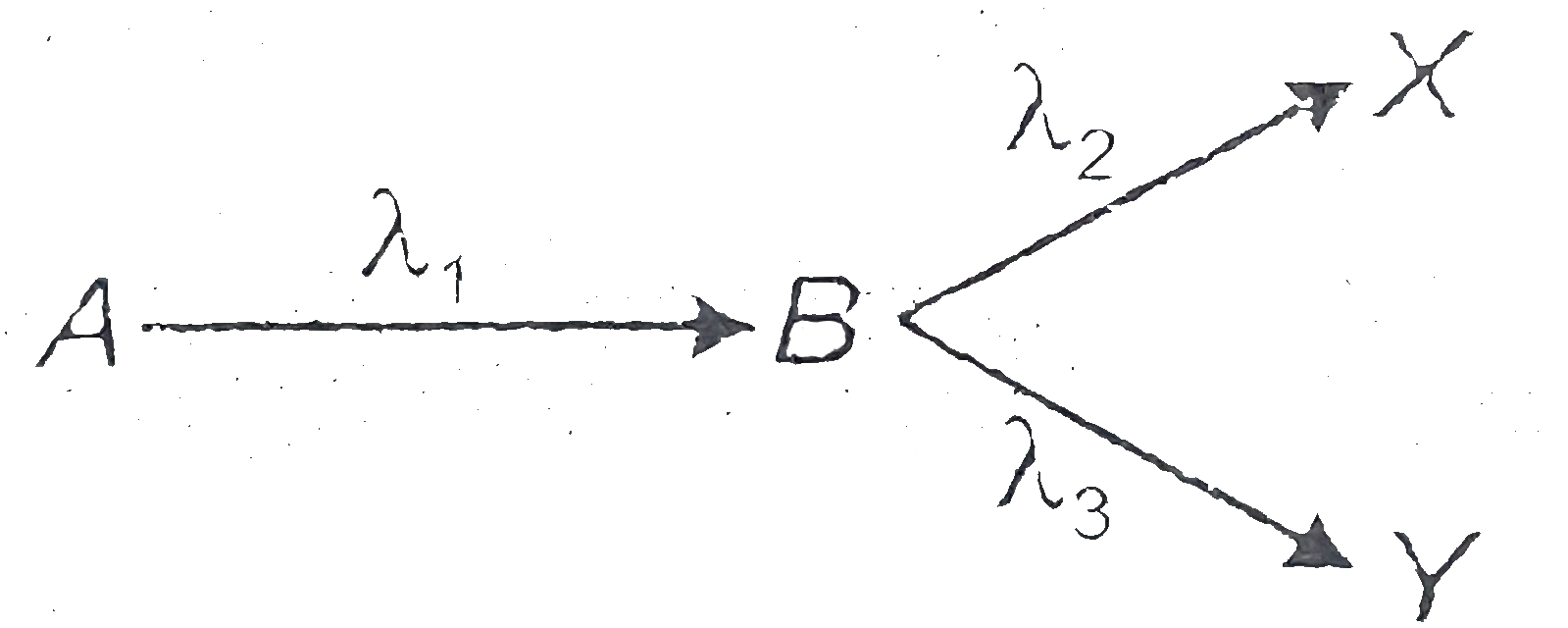
and N1,N2,N3,N4 are number of nuclei A,B , X and Y at any instant.
Rate of accumulation of B of any instant will be
View Solution
Knowledge Check
The rate of disintegration at a given instant, is directly proportional to the number of atoms present at that instant. This is the statement of
Alaw of radioactive decayBhalf lifeClaw of radioactive transformationDgroup displacement lawIf n1 is the number of radio-atoms present at tiem 't' the following expression will be a constant,
AnttBInnttCdInntdtDtntThe rate at which a particular decay process occurs in a radio active sample, is proportional to the number of radio active nuclei present. If N is the number of radio active nuclei present at some instant, the rate of change of N is dNdt=−λN.
_E01_161_Q01.png)
Consider radioavtive decay of A to B which may further decay, either to X or to Y, λ1,λ2 and λ3 are decay constants for A to B decay, B to X decay and B of Y decay respectively. If at t=0 number of nuclei of A, B, X and Y are N0,N0, zero and zero respectively and N1,N2,N3,N4 are number of nuclei A,B,X and Y at any intant.
At t=∞, which of the following is incorrect ?AN2=0BN3=N0λ2λ2+λ3CN4=2N0λ3λ2+λ3DN3+N4+N1+N2=2N0
The rate at which a particular decay process occurs in a radio sample, is proportional to the number of radio active nuclei present . If N is the number of radio active nuclei present at some instant, the rate of change of N is dNdt=−λN. Consider radioactive decay of A to B which may further decay either to X or to Y, λ1,λ2andλ3 are decay constants for A to B decay , B to X decay and B of Y decay respectively. if at t=0 number of nuclei of A,B , X and Y are N0,N0,zero and zero respectively

and N1,N2,N3,N4 are number of nuclei A,B , X and Y at any instant.
At t=∞, which of the following is incorrect ?
View SolutionThe rate at which a particular decay process occurs in a radio sample, is proportional to the number of radio active nuclei present . If N is the number of radio active nuclei present at some instant, the rate of change of N is dNdt=−λN. Consider radioactive decay of A to B which may further decay either to X or to Y, λ1,λ2andλ3 are decay constants for A to B decay , B to X decay and B of Y decay respectively. if at t=0 number of nuclei of A,B , X and Y are N0,N0,zero and zero respectively
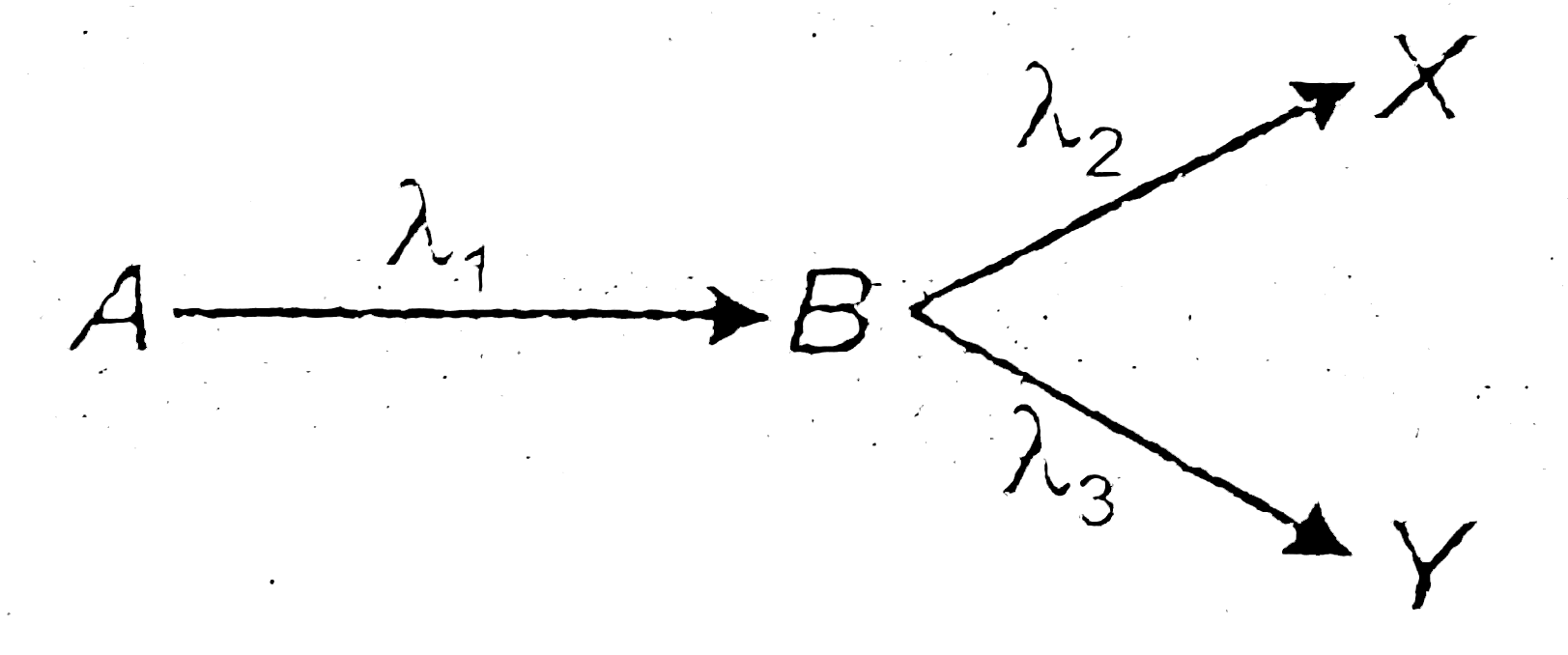
and N1,N2,N3,N4 are number of nuclei A,B , X and Y at any instant.
The number of nuclei of B will first increase then after a maximum value, it will decreases, if
View SolutionThe rate of increase of the bacteria in a culture is proportional to the number of bacteria present and it is found that the number doubles in 6 hours. Prove that the bacteria becomes 8 times at the end of 18 hours.
View SolutionBacteria increases at the rate proportional to the number of bacteria present. If the original number N doubles in 3 hours, find in how many hours the number of bacteria will by 4N ?
View SolutionThe rate of growth of a population is proportional to the number present. if the population of a city doubled in the past 25 years, and the present population is 100000, when will the city have a population of 500000? [Given, loge5=1.609 , loge2=0.6931 . ]
View Solution
