ΔDEF ~ ΔABC. If DE : AB = 2 : 3 and ar ΔDEF is equal to 44 square units then ar (ΔABC) (square unit) is
99
66
120
130
The correct Answer is:A
समान प्रश्न
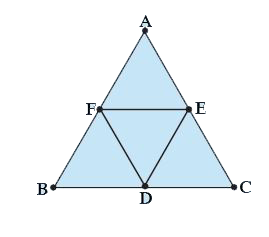
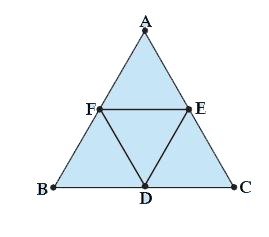
In the figure, ΔABC, D, E, F are the midpoints of sides BC, CA and AB respectively. Show that
(i) BDEF is a parallelogram
(ii) ar(ΔDEF)=14ar(ΔABC)
(iii) ar(BDEF)=12ar(ΔABC)
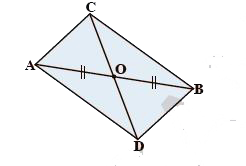
View SolutionIn the figure, ΔABC and ΔABD are two triangles on the same base AB. If line segment CD is bisected by ¯¯¯¯¯¯AB at O, show that ar (ΔABC)=ar(ΔABD).
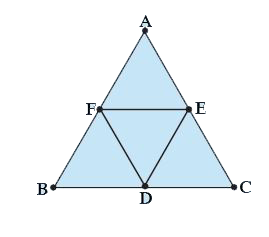
View SolutionIn the figure, ΔABC, D, E, F are the midpoints of sides BC, CA and AB respectively. Show that
(i) BDEF is a parallelogram
(ii) ar(ΔDEF)=14ar(ΔABC)
(iii) ar(BDEF)=12ar(ΔABC)
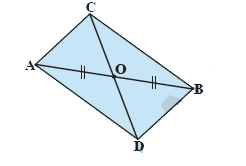
View SolutionIn the figure, ΔABC and ΔABD are two triangles on the same base AB. If line segment CD is bisected by ¯¯¯¯¯¯AB at O, show that ar (ΔABC)=ar(ΔABD).
View SolutionIn the figure, ΔABC, D, E, F are the midpoints of sides BC, CA and AB respectively. Show that
(i) BDEF is a parallelogram
(ii) ar(ΔDEF)=14ar(ΔABC)
(iii) ar(BDEF)=12ar(ΔABC)
View SolutionIf ΔABC is similar to ΔDEF such that 2 AB = DE and BC = 8 cm then EF is equal to.
View SolutionΔABC ~ ΔDEF. If AB = 4 cm, BC = 3.5 cm, CA = 2.5 cm and DF = 7.5 cm, then the perimeter of ΔDEF is
View Solution
