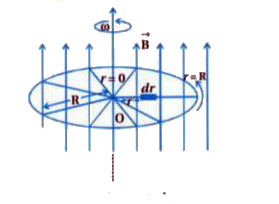
A wheel having n conducting concentric spokes is rotating about it geometrical axis with an angular velocity ω, in a uniform magnetic field B perpendicular to its plane prove that the induced emf generated between the rim of the wheel and the center is ωBR22, where R is the radius of the wheel. It is given that the rim of the wheel is conducting.
 Solution in Gujrati
Solution in Gujrati
Consider a small element dr on any spoke at a distance r from the center.
Linear velocity of this element v=rω, emf induced in a small element dr is,
dε=Bvl
=B(rω)dr
Total emf induced along the entire length of any spoke is ,
ε=∫R0Bωrdr
∴ε=Bω∫R0rdr
∴ε=Bω[r22]R0
∴ε=Bω[R22−022]
∴ε=BωR22 which is proved.
Now according to right hand screw rule with equation →F=−e(→v×→B) shows that free electrons in a spoke will experience force towards the center of the wheel therefore, the free electrons accumulate at the center of the wheel leaving the rim positively charged. So it act as battery with emf will be BωR22.
Here voltage obtained from all spokes are parallel so the resultant emf will be BωR22.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section C Ncert Exemplar Solution (MCQs)
16 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section C Ncert Exemplar Solution (Short Answer )
5 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section B (Numerical) Numerical From Textual Exercise
17 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - KUMAR PRAKASHANCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION D MCQS ASKED IN COMPETITIVE EXAMES (MCQS AKSED IN BOARD EXAM AND GUJCET)
14 Videos
- ELECTROMAGNETIC WAVESBOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - BOARD.S QUESTION PAPER MARCH - 2020 (PART - B) SECTION - C
5 Videos
Similar Questions
A square of side L meters lies in the xy-plane in a region, where the magnetic field is given by →B=B0(2ˆi+3ˆj+4ˆk)T where B0 is constant. The magnitude of flux passing through the square is
View SolutionA magnetic field in a certain region is given by →B=B0cos(ωt)ˆk and a coil of radius a with resistance R is placed in the xy-plane with its centre at the origin in the magnetic field (see figure). Find the magnitude and the direction of the current at (a, 0, 0) at t=π2ω,πω and t=3π2ω.
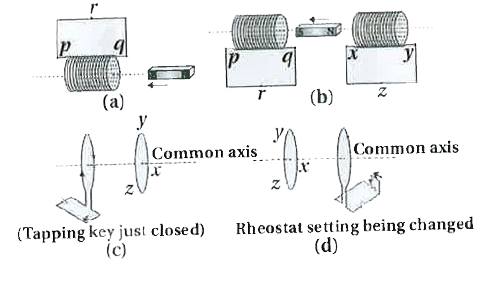
View SolutionPredict the direction of induced current in the situations described by the following figures (a) to (d).
View SolutionWhen air is replaced by dielectric medium of constant K, the maximum force of attraction between two charges separated by distance d, ..........
View SolutionDetermine the current in each branch of the given network.

View SolutionA wheel with 4 spokes is placed with its plane perpendicular to a uniform magnetic field B of magnitude 0.5 T. The field is directed into the plane of the paper and is present over the entire region of the wheel as shown in Fig. When the switch S is closed, there is initial current of 6 A between the axis and the rim and the wheel begins to rotate. Resistances of the spokes are 1, 2, 4 and 8 Omega , respectively. Resistance of rim is negligible. a. What is the direction of rotation of the wheel? b. Radius of the wheel is 0.2 m. Calculate initial torque acting on the wheel.
View SolutionA wheel has three spokes and is an uniform magnetic field perpendicular to its plane, with the axis of rotation of the wheel parallel to the magnetic field. When the wheel rotates with a uniform angualr velocity ω, the emf induced between the centre and rim of the wheel is 'e'. If another wheel having same radius but with six spokes is kept in the same field and rotated with a uniform angular velocity 'ω/2', the emf induced between the centre and the rim will be
View SolutionA non-conducting disk of radius R is rotating about its own axis with constant angular velocity omega in a perpendicular uniform magnetic field B as shown in figure. The emf induced between centre and rim of disk is
View SolutionThe spokes of a wheel are made of metal and their lengths are of one metre. On rotating the wheel about its own axis in a uniform magnetic field of 5×10−5 tesla normal to the plane of the wheel, a potential difference of 3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is-
View SolutionA wheel with 10 spokes each of length 'L' m is rotated with a unifrom angular velocity omega in a plane normal to the magnetic field 'B' . The emf induced between the axle and the rim of the wheel.
View SolutionA conducting wheel in which there are four rods of length l is rotating with angular velocity omega in a uniform magnetic field B . The induced potential difference between its centre and rim will be __
View SolutionA conducting wheel in which there are four rods of lengthe l as shown in figure is rotating with angular velocity ω in a uniform magnetic field B. The induced potential difference between its centre and rim will be

View SolutionIf a spoke is connected between the rim of the wheel and the centre (axlc) and Bμ is the earth 's horizontal component of total field perpendicular to the plane of the wheel and the wheel is rotated with an angular speed, then what will be the induced emf between the ends of the spoke ?
View SolutionA wheel with 20 metallic spokes each 1m long is rotated with a speed of 120 rpm in a plane perpendicular to magnetic field of 0.4G. The induced emf between axle and rim of wheel will be
View SolutionA wheel with N spokes is rotated in a plane - perpendicular to the magnetic field B such emf e is induced between axie and rim of the wheel . In the same wheel number of spokes is made 3 N and the wheel is rotated in the same manner in the same field then new emf is ________ .
View Solution
