असमीका 2log1/2(x−1)≤13−1logx2−x8 को संतुष्ट करने वाले पूर्णाकों की संख्या ≤10 होगी
The correct Answer is:9
Step by step video, text & image solution for असमीका 2log_(1//2) (x-1) le (1)/(3)- (1)/(log_(x^(2)-x)8) को संतुष्ट करने वाले पूर्णाकों की संख्या le 10 होगी by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ERRORLESS HINDICHAPTER - लघुगणक और आंशिक भिन्नें EXERCISE - JEE Advanced (More than one correct answers)5videos
लघुगणक और आंशिक भिन्नें
- BOOK - ERRORLESS HINDICHAPTER - फलन, सीमा, सांतत्य तथा अवकलनीयताEXERCISE - JEE ADVANCED( MATCH THE COLUMN TYPE)13videos
फलन, सीमा, सांतत्य तथा अवकलनीयता
- BOOK - ERRORLESS HINDICHAPTER - वर्ग समीकरण एवं असमिकायें EXERCISE - JEE Advanced (Matrix Match type questions)2videos
वर्ग समीकरण एवं असमिकायें
Similar Questions
यदि 3x=4x−1, तब x=
05:17View Solutionकॉलम I में दिए गए वक्तव्यों/व्यंजकों का कॉलम II में दिये गये मानों से सुमेल करे।
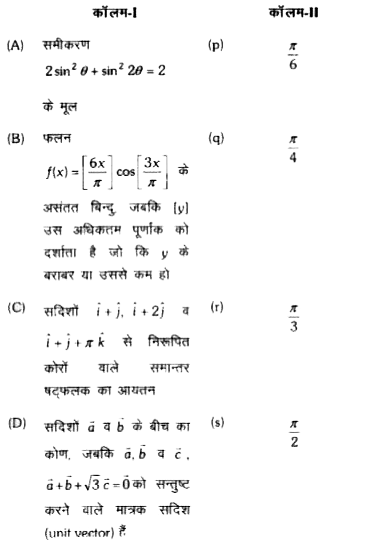05:57View Solution
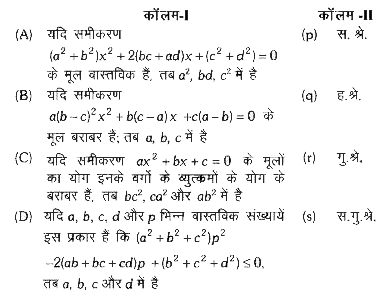04:45View SolutionNumber of integers ≤10 satisfying the inequality 2(log)12(x−1)≤13−1(log)x2−x8 is............
04:53View SolutionThe equation (log_(10)x+2)^(3)+(log_(10)x-1)^(3)=(2log_(10)x+1)3
03:23View SolutionNumber of integers ≤10 satisfying the inequality 2log1/2(x−1)≤13−1logx2−x8 is ________.
04:31View SolutionIf f(x)=−1+|x−1|,−1≤x≤3 and g(x)=2−|x+1|,−2≤x≤2, then find fog(x) and gof(x).
02:35View SolutionLet f(x) and g(x) be two functions given by f(x)=−1|x−1|,−1≤x≤3 and g(x)=2−|x+1|,−2≤x≤2
Then,View SolutionFind x if 91/log23≤log2x≤log827
05:29View SolutionIf 12≤log0.1x≤2, then
03:19View Solution(स्तम्भअ,स्तम्भब),(i.असमीकाx2−1≤0काहल,a.(−∞.−1)∪(1.∞)),(ii.असमीकाx−1x+1≤0काहलb.[−1.1]),(iii.असमीकाx2>1काहल,c.(−∞.−1)∪(1.∞)),(iv.असमीकाx−1x+1≥0काहल,d.(−1.1))
View Solutionफलन के लिए रोल प्रमेय की सत्यता की जाँच कीजिए
f(x)=⎧⎪⎨⎪⎩x2+10≤x≤1यदि3−x1<x≤204:32View Solutionlog4(x−1)=log2(x−3) के हेलो की संख्या होगी
04:30View SolutionIf log_(5)(6+(2)/(x))+log_((1//5)) (1+(x)/(10)) le 1 , then x lies in :
05:51View SolutionIf (1)/(2) le log_(0.1) x le 2 so
03:26View Solution