There are five terms in an arrithmetic progression. the Sum of these terms is 55, and the fourth term is five more than the sum of the first two terms.Find the terms of the Arithmaetic progression.Solution in Kannada
The correct Answer is:The five terms are .
Step by step video, text & image solution for There are five terms in an arrithmetic progression. the Sum of these terms is 55, and the fourth term is five more than the sum of the first two terms.Find the terms of the Arithmaetic progression. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ARITHMETIC PROGRESSIONSBOOK - ZEN PUBLICATIONCHAPTER - ARITHMETIC PROGRESSIONSEXERCISE - ZEN ADDITIONAL QUESTIONS (HOTS [HIGHER ORDER THINKING SKILLS] - QUESTIONS)
4 Videos
- ARITHMETIC PROGRESSIONSBOOK - ZEN PUBLICATIONCHAPTER - ARITHMETIC PROGRESSIONSEXERCISE - ZEN ADDITIONAL QUESTIONS (SHORT-ANSWER [SA] TYPE 2 - QUESTIONS)
14 Videos
- AREA RELATED TO CIRCLES BOOK - ZEN PUBLICATIONCHAPTER - AREA RELATED TO CIRCLES EXERCISE - ADDITIONAL QUESTIONS (HIGHER ORDER THINKING SKILLS)
7 Videos
- CIRCLESBOOK - ZEN PUBLICATIONCHAPTER - CIRCLESEXERCISE - ZEN ADDITIONAL QUESTIONS ( HOT [HIGHER ORDER THINKING SKILLS] - QUESTIONS) (IIT AND IMO)
9 Videos
Similar Questions
If m times the mth term of an A.P. is equal ton times the nth term and m≠n, show that (m+n)th term is zero.
View SolutionFind the 12th term from the end of AP 8, 10, 12, ..... , 130
View SolutionFind the area of the shaded region portion in the given figure if E is the midpoint of AB,AGE and BEF are quadrants, and DOC is a semicircle.

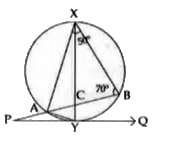
View SolutionIn the adjoining figure, XY is a diameter of the circle and PQ is a tangent to the circle at Y. Given that ⌊AXB=50∘ and ⌊ABX=70∘, calculate ⌊BAY and ⌊APY.
View SolutionAn aritmetic progression consists of five terms whose sum is 15 and sum of the squares of the extremes is 58. Find the terms of progression.
View SolutionIf the first term of an A.P. is 2 and the sum of first five terms is equal to one fourth of the sum of the next five terms, find the sum of first 30 terms.
View SolutionThe sum of the first four terms of a geometric series is 520. The sum of the first five terms is 844. The sum of the first six terms is 1330. (a) Find the common ratio of the geometric progression.(b) Find the sum of the first two terms.
View Solutionकिसी समांतर श्रेणी का प्रथम पद 2 हैं और प्रथम पाँच पदों का योग , उसके अगले (next) पाँच पदों के योग एक चौथाई हैं , तो सिद्ध कीजिए कि इसका 20 वाँ पद -112 हैं ।
View SolutionThe sum of the fourth and twelfth term of an arithmetic progression is 20. What is the sum of the first 15 terms of the arithmetic progression?
View SolutionThere are five terms in an arrithmetic progression. the Sum of these terms is 55, and the fourth term is five more than the sum of the first two terms.Find the terms of the Arithmaetic progression.
View SolutionIn an arithmetic progression sixth term is one more than twice the thrid term. Tha sum of the fourth and fifth terms is five times the secon term. Find the tenth term of the arithmatic progression.
View SolutionThe sum of an infinite geometric progression is 243 and the sum of its first five terms is 275 The first term of the progression
View SolutionThe first, second and seventh terms of an arithmetic progression (all the terms are distinct) are in geometric progression and the sum of these three terms is 93. Then, the fourth term of this geometric progression is
View SolutionIn an A.P the first term is 2 and the sum of first five terms is One fourth of the next five terms, then 20th term is
View SolutionIn an A.P., the first term is 2 and the sum of the first five terms in one-fourth of the next five terms then the 20th term is
View SolutionIf the first term of an A.P. is 2 and the sum of first five terms is equal to one fourth of the sum of the next five terms, find the sum of first 30 terms.
View Solution
