If sin3A=cos(A−10∘) where 3A is an acute angle, find the value of A.Solution in Kannada
The correct Answer is:A=25∘.
Step by step video, text & image solution for If sin 3A = cos (A-10^(@)) where 3A is an acute angle, find the value of A. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- AN INTRODUCTION TO TRIGONOMETRYBOOK - ZEN PUBLICATIONCHAPTER - AN INTRODUCTION TO TRIGONOMETRYEXERCISE - Exercise 11.1
16 Videos
- AN INTRODUCTION TO TRIGONOMETRYBOOK - ZEN PUBLICATIONCHAPTER - AN INTRODUCTION TO TRIGONOMETRYEXERCISE - Exercise 11.2
15 Videos
- AREA RELATED TO CIRCLES BOOK - ZEN PUBLICATIONCHAPTER - AREA RELATED TO CIRCLES EXERCISE - ADDITIONAL QUESTIONS (HIGHER ORDER THINKING SKILLS)
7 Videos
Similar Questions
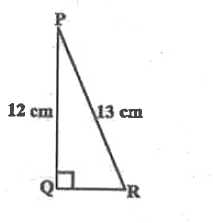
In ΔABC, right - angled at B,AB=24cm,BC=7cm. Determine :
(i) sinA,cosA
(ii) sinC,cosC
View Solutionsin60∘cos30∘+sin30∘cos60∘=?
View SolutionFind the area of the shaded region portion in the given figure if E is the midpoint of AB,AGE and BEF are quadrants, and DOC is a semicircle.

View SolutionIf sin3A=cos(A−26∘), where 3A is an acute angle, find the value of A.
View SolutionSin3A=Cos(A−26), where 3A is an acute angle triangle, Find the value of A.
View SolutionIf sin3A=cos(A−10∘) where 3A is an acute angle , then find the value of A.
View Solutionयदि sin3A=cos(A−26∘) हो, जहाँ, 3 A एक न्यून कोण है तो A का मान ज्ञात कीजिए ।
View Solutionयदि sin3A=cos(A−26∘) हो, जहाँ 3A एक न्यूनकोण है तो A का मान ज्ञात कीजिए।
View Solutionयदि sin3A=cos(A−26), हो, जहाँ 3A एक न्यूनकोण है, तो A का मान ज्ञात कीजिए।
View Solutionयदि sin3A=cos(A−26∘), जहाँ 3A एक न्यूनकोण है , तब A =
View Solutionयदि sin3A=cos(A−10∘) जहाँ 3A न्यूनकोण है, तो A का मान ज्ञात कीजिए|
View Solutionयदि tan3A=cot(A−10∘) और 3A एक न्यूनकोण है , तो A का मान ज्ञात कीजिए |
View Solutionयदि tan3A=cot(A−10∘) जहाँ 3A न्यूनकोण है , तो A का मान ज्ञात कीजिए |
View Solutionयदि cos3A=sin(A−34∘) हो, जहाँ 3A एक न्यून कोण है तो A का मान ज्ञात कीजिये।
View SolutionIf sin3A=cos(A−26∘), where 3A is an acute angle, then find the value of A.
View Solution
